TS Inter 1st Year Maths 1A Solutions Chapter 3 Matrices Ex 3(i)

Students must practice these TS Intermediate Maths 1A Solutions Chapter 3 Matrices Ex 3(i) to find a better approach to solving the problems.

TS Inter 1st Year Maths 1A Matrices Solutions Exercise 3(i)

Question 1.
2x + 3y – z = 0,
x – y – 2z = 0,
3x + y + 3z = 0
Answer:
The coefficient matrix obtained from the given equations is

Since the determinant of the coefficient matrix ≠ 0 the system has a trivial solution, x = y = z = 0 and ρ(A) = 3.

Question 2.
3x + y – 2z = 0,
x + y + z = 0,
x – 2y + z = 0
Answer:
The coefficient matrix obtained from the given equations is

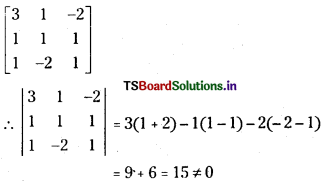
ρ(A) = 3; and the system has a trivial solution x = y = z = 0

Question 3.
x + y – 2z = 0,
2x + y – 3z = 0,
5x + 4y – 9z = 0
Answer:
The coefficient matrix is
A = \(\left[\begin{array}{lll}
1 & 1 & -2 \\
2 & 1 & -3 \\
5 & 4 & -9
\end{array}\right]\)
and \(\left|\begin{array}{lll}
1 & 1 & -2 \\
2 & 1 & -3 \\
5 & 4 & -9
\end{array}\right|\)
= 1(-9 + 12) – 1(-18 + 15) – 2
= 3 + 3 – 6 = 0
If \(\left[\begin{array}{ll}
1 & 1 \\
2 & 1
\end{array}\right]\) is any submatrix of order 2 x 2 and
\(\left|\begin{array}{ll}
1 & 1 \\
2 & 1
\end{array}\right|\) = 1 – 2 = -1 ≠ 0, ρ(A) < 3. Hence the system has a nontrival solution.

∴ System of equations is equivalent to
x + y – 2z = 0 and y – z = 0
Let z = k then y = k and x = k
∴ x = y = z = k for a real number k.

Question 4.
x + y – z= 0
x – 2y + z = 0
3x + 6y – 5z = 0
Answer:
Coefficient matrix
A = \(\left[\begin{array}{ccc}
1 & 1 & -1 \\
1 & -2 & 1 \\
3 & 6 & -5
\end{array}\right]\)
|A| = 1(10 – 6) – 1(-5 – 3) – 1(6 + 6)
= 4 + 8 – 12 = 0

∴ If \(\left[\begin{array}{cc}
1 & 1 \\
1 & -2
\end{array}\right]\) is a submatrix of order 2 and
\(\left|\begin{array}{cc}
1 & 1 \\
1 & -2
\end{array}\right|\) = -3 ≠ 0, ρ(A) = 2. System has a non-trivial solution ρ(A) < 3.
A = \(\left[\begin{array}{rrr}
1 & 1 & -1 \\
1 & -2 & 1 \\
3 & 6 & -5
\end{array}\right]\)
Use R₂ – R₁ and R₃ – 3R₁
A – \(\left[\begin{array}{rrr}
1 & 1 & -1 \\
0 & -3 & 2 \\
0 & 3 & -2
\end{array}\right]\)
System of equations is equivalent to x + y – z = 0
3y – 2z = 0
Let z = k, then 3y = 2k
⇒ y = \(\frac{2 \mathrm{k}}{3}\)
x = -y + z = –\(\frac{2 \mathrm{k}}{3}\) + k = \(\frac{k}{3}\)
x = \(\frac{k}{3}\), y = \(\frac{2 \mathrm{k}}{3}\), z = k
for any real number of k.