TS Inter 2nd Year Maths 2B System of Circles Important Questions

Students must practice these TS Inter 2nd Year Maths 2B Important Questions Chapter 2 System of Circles to help strengthen their preparations for exams.

TS Inter 2nd Year Maths 2B System of Circles Important Questions

Very Short Answer Type Question

Question 1.
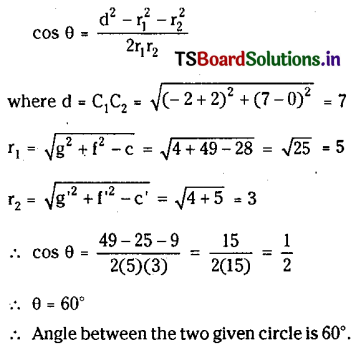
Find the angle between the circles x²+y²+4x-14y+28=0
x²+y²+4x – 5 = 0
Solution:
Comparing with general equation
x²+y²+2gx+2fy+c=0, we have
g=2, 1=-7, c=28, C₁=(-2,7)
g’=2, f’=0, c=-5, C₂=(-2,0)
Let θ be the angle between circles (1) and (2) then

Question 2.
If the angle between the circles x²+y²-12x- 6y+41=0 ………………..(1) x²+y²+kx+6y-59=0 …………… (2) is 45° find k.
Solution:


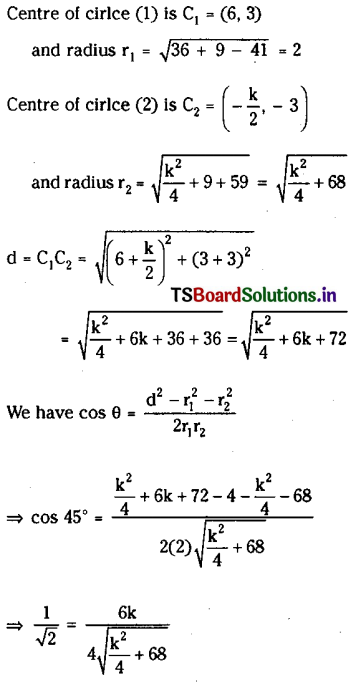
⇒ k² + 27². 18k²
⇒ 17k² = 27²
⇒ k²=16
k = ± 4

Short Answer Type Questions

Question 1.
find the equation of the circle passing through the points of the intersection of the circles
x²+y²– 8x-6y+21=0 …………… (1)
x²+y²– 2x-15=0 …………. (2) and (1, 2)
Solution:
The equation of circle passing through the point of intersection o! circles S = 0, S’ = 0 is S+ λS’+ 0 where λ is a parameter.
∴(x²+y²-8x-6y+21) +λ(x²+y²-2x-15)=0 ……………….. (1)
If this passes through (1, 2) then
(1+4-8-12+21)+λ(1+4-2-15)=0
= 6-12λ=0 ⇒ λ= \(\frac{1}{2}\)
Hence the equation of the required circle
is (x²+y²-8x-6y+ 21) + \(\frac{1}{2}\) (x²+y²-2x-15) = 0
= 3(x²+y²)-18x-12y+ 27 = 0

Question 2.
Find the equation and length of the common chord of the circles
S ≡ x² +y²+ 3x + 5y+ 4 = 0 ……………….. (1)
and S ≡ x² +y²+ 5x+ 3y + 4 = 0  …………………. (2)
Solution:
The common chord of two intersecting circles is the radical axis given by S – S’ = 0.
⇒  2x + 2y = 0
⇒ x-y=0 ……………… (3)
Centre of cricle (1) is \(\mathrm{C}_1=\left(-\frac{3}{2},-\frac{5}{2}\right)\) and

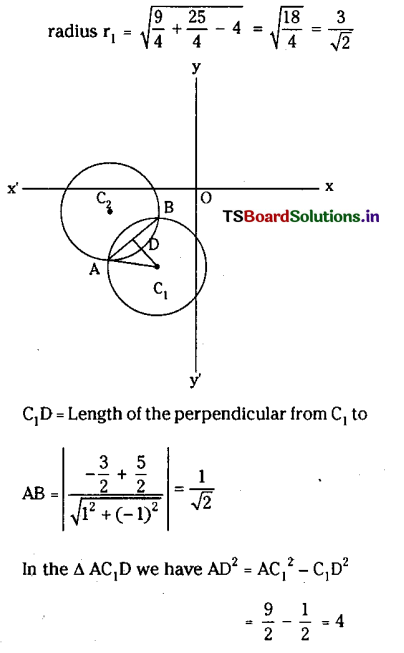
∴ AD = 2
∴ Length of the common chord AB = 2(AD) = 2(2) 4

Question 3.
Find the equation of the circle whose diameter is the common chord of the circles
S = x² +y²+2x+3y+ 1=0 …………………. (1)
and S’= x² +y²+4x+3y+2=0 …………………. (2)
Solution:
The common chord is the radical axis of (1) and (2) given by S – S’ = 0.
⇒ 2x-1 =0
⇒  2x+1=0 ………………. (3)
The equation of any circle passing through the point of intersection of (1) and (3) is
S + λL = 0.
(x² +y²+2x+3y+1)+λ(2x+1) = 0
x² +y²+2(λ+ 1)x+3y+(1 +λ) = 0 ……………….. (4)
Centre of this circle = [- (λ + 1), \(-\frac{3}{2}\)]
For the circle (4), 2x + 1 -0 is one chord. This will be the diameter of the circle (4).
If the centre of (4) lies on (3) then
– 2(λ + 1) + 1 = 0
⇒ λ=-\(-\frac{3}{2}\)
∴ The equation of circle whose diameter is the common chord of (1) and (2) is
x² +y² + 2x + 3y + 1) – (2x + 1) = 0
2(x² +y²)+2x+6y+1=0

Question 4.
Find the equation of a circle which cuts each of the following circles orthogonally
S’ = x²+y²+3x+2y+1=0 ……….. (1)
S”=x²+y²-x+6y+5=0 ………… (2)
S”’=x²+y+5x-6y+15=0 …………… (3)
Solution:
Radical axis of circles (1) and (2) is S’ -S”= 0.
⇒ 4x-4y-4=0
⇒ x-y-1=0   ………………… (4)
Radical axis of circles (2) and (3) is S’’ -S”’= 0.
⇒ -6x+ 14y-10 =0
⇒ 3x-7y+5= 0 ……………. (5)
Solving (4) and (5) we get

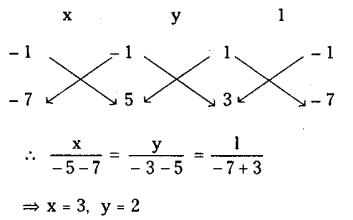
∴ Radical centre – (3, 2)
Also the length of the tangent from (3, 2) to
S’=\(\sqrt{9+4+9+4+1}=\sqrt{27}\)
Hence equation of circle which cuts orthogonally each of the given circles is obtained by taking radical centre as centre and length of the tangent as radius.
∴ Equation of the required circle is
(x-3)² +(y-2)²= 27
⇒ x²+y²-6x-4y-14=0.

Long Answer Type Questions

Question 1.
Find the equation of the circle which passes through (1, 1) and cuts orthogonally each of the circles
x²+y²-8x-2y+ 16=0 …………. (1)
and x²+y²-4x-4y-1=0 …………. (2)
Solution:
Let the equation of the required circle be
x²+y²+2gx+2fy+c = 0 ………….. (3)
1f this passes through (1, 1) then
1+1 + 2g. 2f + c = 0
⇒ 2g+2f+c- 2 ………….. (4)
If (3) is orthogonal to (1) then
2g(-4) + 2f(-1) = c + 16
=-8g-21=c+16 …………………. (5)
11(3) is orthogonal to (2) then
2g(-2) + 2f(-2) =c-1
= – 4g – 41=c – 1 ………….. (6)
From (5) and (6) we have
– 4g + 21=17
⇒ 4g+2f=-17 …………….. (7)
From (4) and (5) we have
– 6g=+ 14=g= – \(\frac{7}{3}\)
∴ From (7)

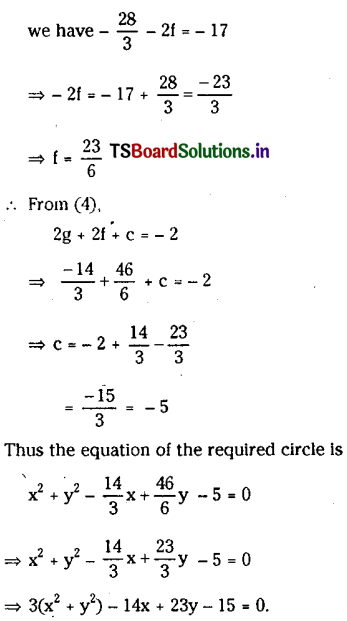
Question 2.
Find the equation of circle which is orthogonal to each of the following three circles x²+y²+ 2x + 17y+ 4=0
x²+y²+7x+6y+ 11=0 and x²+y²-x+22y+3=0
Solution:
Denote the given circles by
S=x²+y²+2x+ 17y+4 = 0 ……………….. (1)
S’=x²+y²+7x+6y+11 = 0 ……………… (2)
and S”=x²+y² x+22y+3 = 0 …………………. (3)
The radical axis of S = 0, S’ = 0 is s – S = 0.
⇒ – 5x+11y – 7=0
⇒ 5x – 11y-7=0 ……………….. (4)
The radical axis of S’ = 0. S” = 0 is S’ = S’’ = 0.
⇒ 8x-16y+8=0
⇒ x-2y+1=0 …………… (5)
Solving equations (4) and (5), we get the coordinates of radical centre.

⇒ x = 3, y = 2 ∴ C (3, 2) is the radical centre.
Length of the tangent from C(3, 2) to the circles = 0 is \(\sqrt{9+4+6+34+4}=\sqrt{57}\)
The equation of the circle which cuts or orthogonally each of the three circles if obtained by considering the equation of circle with radical centre (3,2) as centre and length of the tangent \(\sqrt{57}\) as the radius.
∴ Equation of the required circle is
(x-3)² + (y – 2)² = 57
= x² + y²-6x-4y-44 = 0

Question 3.
If the straight line represented by x cos α + y sin α = p intersects the code x² + y² = a² at the points A and B then show that the equation of circle with \(\overline{\mathbf{A B}}\) as diameter is (x²+y²-a²)-2p(x cosα+ysinα – p)=0.
Solution:
Given x cos α + y sin α p …….. (1) intersects the circle
x² + y² = a² ……….. (2) at points A and B.
So the equation of circle passing through the point of intersection of (1) and (2) is
x² + y² = a²+λ(xcos α + ysinα-p) = 0 …………. (3)
Where λ is a parameter.
∴ Centre of (3) is  \(\left(\frac{-\lambda \cos \alpha}{2}, \frac{-\lambda \sin \alpha}{2}\right)\)
If the circle given by (3) has \(\overline{\mathrm{AB}}\) as diameter then the centre of it must lie on (1). Then

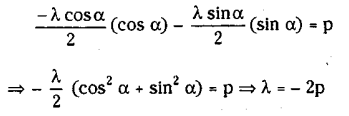
Hence the equation of the required circle is (x² + y²– a²) – 2p(xcosα+ysinα – p)=0.

Question 4.
Show that the circles
S=x²+y²-2x-4y-20 =0 …………….. (1)
and S’=x²+y²+6x – 2y- 90=0 …………… (2)
touch each other Internally. Find their point of contact and the equation of common tangent
Solution:
Let C₁ C₂ be the centres of circles (1) and (2) and r₁ r₂ be the radii of circles (1) and (2).
Then C₁ = (1, 2) and r₁ = \(\sqrt{1+4+20}\) = 5

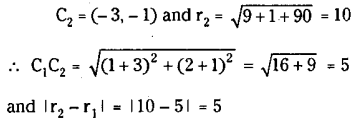
Since C₁ C₂ – |r₁ r₂|, the two circles touch
Internally. The point of contact P divides C₃ C₄ externally In the ratio of the radii of circles 5: 10 1: 2.

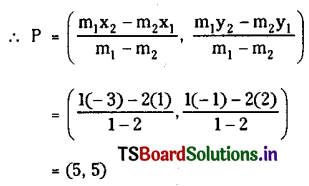
Since the two circles touch internally the common tangent at the point of contact is only the radical axis of
S = 0 and S’- 0 given by S – S’ – 0.
= – 8x – 6y + 70 = 0
= 4x + 3y – 35 = 0