TS Inter 1st Year Maths 1A Solutions Chapter 7 Trigonometric Equations Ex 7(a)

Students must practice these TS Intermediate Maths 1A Solutions Chapter 7 Trigonometric Equations Ex 7(a) to find a better approach to solving the problems.

TS Inter 1st Year Maths 1A Trigonometric Equations Solutions Exercise 7(a)

I.
Question 1.
Find the principal solutions of the angles in the equations. (V.S.A)
(i) 2 cos² θ = 1
Answer:
cos² θ = \(\frac{1}{2}\)
⇒ principal values are θ = 45°,135° (∵ cos θ = ±\(\frac{1}{\sqrt{2}}\))

(ii) √sec θ + 2 = 0
Answer:
sec θ = \(\frac{-2}{\sqrt{3}}\), cos θ = – \(\frac{\sqrt{3}}{2}\)
∴ Principal solution is θ = 150°.

(iii) √3 tan²θ = 1
Answer:
tan²θ = \(\frac{1}{3}\) ⇒ tan θ = ± \(\frac{1}{\sqrt{3}}\)
∴ θ = ± \(\frac{\pi}{6}\)

Question 2.
Solve the following equations. (VSA)
(i) cos 2θ = \(\frac{\sqrt{5}+1}{4}\), θ ∈ [0, 2π]
Answer:
cos 2θ = \(\frac{\sqrt{5}+1}{4}\) = cos 36° = cos \(\left(\frac{\pi}{5}\right)\)
and \(\frac{\pi}{5}\) ∈ [0, 2π] ∴ 2θ = \(\frac{\pi}{5}\) ⇒ θ = \(\frac{\pi}{10}\)
is the principal solution.
and 2θ = 2nπ ± \(\frac{\pi}{5}\) where n ∈ Z is the general solution
∴ θ = nπ ± \(\frac{\pi}{10}\)
Values of θ in [0, 2π] are \(\left\{\frac{\pi}{10}, \frac{9 \pi}{10}, \frac{11 \pi}{10}, \frac{19 \pi}{10}\right\}\)
for n = 0, n = 1 and n = 2.

(ii) tan²θ = 1, θ ∈ [- π, π]
Answer:
tan θ = ± 1 + tan \(\left(\pm \frac{\pi}{4}\right)\)
The principal solutions are θ = ± \(\frac{\pi}{4}\)
and general solution is θ = nπ ± \(\frac{\pi}{4}\), n ∈ Z
For n = – 1, 0, 1 we have \(\left\{-\frac{3 \pi}{4}, \frac{-\pi}{4}, \frac{\pi}{4}, \frac{3 \pi}{4}\right\}\) is
the solution set [or the given equation in [-π, π].

(iii) sin 3θ = \(\frac{\sqrt{3}}{2}\), θ ∈ [- π, π]
Answer:

(iv) cos² θ = \(\frac{3}{4}\), θ ∈ [0, π]
Answer:

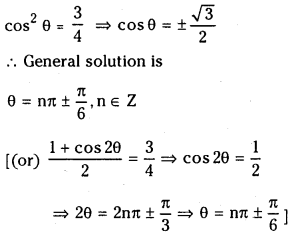
For values of n = 0, 1 we have solution set for the given equation in [0, π] is \(\left\{\frac{\pi}{6}, \frac{5 \pi}{6}\right\}\)

(v) 2 sin² θ = sin θ, θ ∈ (0, π)
Answer:
2 sin² θ = sin θ
⇒ sin θ (2 sin θ – 1) = 0
⇒ sin θ = 0 or sin θ = \(\frac{1}{2}\)
When sin θ = 0 we have θ = nπ
and sin θ = \(\frac{1}{2}\) ⇒ θ = nπ + (- 1)ⁿ \(\frac{\pi}{6}\)
Since θ ∈ (0, π), solution set is \(\left\{\frac{\pi}{6}, \frac{5 \pi}{6}\right\}\)

Question 3.
Find general solutions of the following equations. (V.S.A)
(i) sin θ = \(\frac{\sqrt{3}}{2}\), cos θ = – \(\frac{1}{2}\)
Answer:
sin θ = \(\frac{\sqrt{3}}{2}\), cos θ = – \(\frac{1}{2}\)
⇒ θ has in II quadrant.
∴ The principal value of θ is π – \(\frac{\pi}{3}\) = \(\frac{2 \pi}{3}\)
∴ The general solution is
θ = 2nπ + \(\frac{2 \pi}{3}\), n ∈ Z

(ii) tan x = – \(\frac{1}{\sqrt{3}}\), sec x = \(\frac{2}{\sqrt{3}}\)
Answer:
Given tan x = – \(\frac{1}{\sqrt{3}}\) and sec x = \(\frac{2}{\sqrt{3}}\)
⇒ x lies in IV quadrant.
∴ The principal values of x are \(\frac{-\pi}{6}\) or 2π – \(\frac{\pi}{6}\) = \(\frac{11 \pi}{6}\)
n ∈ Z (or) x = 2nπ – \(\frac{\pi}{6}\), n ∈ Z

(iii) cosec θ = – 2, cot θ = – √3
Answer:
cosec θ = – 2, cot θ = – √3
⇒ θ lies in IV quadrant.
The principal value of θ is
θ = 2π – \(\frac{\pi}{6}\) = \(\frac{11\pi}{6}\) or θ = – \(\frac{\pi}{6}\)
∴ General solution of θ is θ = 2nπ + \(\frac{11\pi}{6}\)
or θ = 2nπ – \(\frac{\pi}{6}\)

Question 4.
(i) If sin (270° – x) = cos 292° then find x in (0, 360°) (V.S.A)
Answer:
sin (270° – x) = cos 292°
= sin (270° – x) = cos (270° + 22°) sin 22°
270° – x = 22° ⇒ x = 270° – 22 = 248°

(ii) If x < 90°and sin (x + 28°) = cos(3x – 78°) then find x.
Answer:
Given sin (x + 28°) = cos (3x – 78°)
= sin [90° – (3x – 78°)]
∴ x + 28° = 90 – (3x – 78°)
⇒ 3x + x = 168° – 28° = 140°
⇒ 4x = 140° ⇒ x = 35°

Question 5.
Find the general solutions of the following equations.
(i) 2 sin² θ 3 cos θ
Answer:
Given 2 sin²θ = 3 cos θ
⇒ 2(1 – cos²θ) = 3 cos θ
⇒ 2 cos² θ + 3 cos θ – 2 = 0
⇒ 2 cos² θ + 4 cos θ – cos θ – 2 = θ
⇒ 2 cos θ(cos θ + 2) – 1 (cos θ + 2) = θ
⇒ (2cos θ – 1) (cos θ + 2) = 0
⇒ cos θ = \(\frac{1}{2}\) or cos θ = – 2 (not admissible)
⇒ cos θ = \(\frac{1}{2}\) ⇒ principal solution is α = – \(\frac{\pi}{3}\)
∴ General solution is θ = 2nπ ± α
= 2nπ ± \(\frac{\pi}{3}\)

(ii) sin² θ – cos θ = \(\frac{1}{4}\)
Answer:
sin² θ – cos θ = \(\frac{1}{4}\)
⇒ 1 – cos² θ – cos θ = 1
⇒ 4 – 4 cos² θ – 4 cos θ = 1
⇒ 4 cos² θ + 4 cos θ – 3 = 0
⇒ 4cos² θ + 6 cos θ – 2 cos θ – 3 = 0
⇒ 2 cos θ(2 cos θ + 3) – 1(2 cos θ + 3) = 0
⇒ (2 cos θ – 1)(2 cos θ + 3)= 0
⇒ cos θ = \(\frac{1}{2}\) or cos θ = \(\frac{-3}{2}\) (not admissible)
If cos θ = \(\frac{1}{2}\) then the principal value α = \(\frac{\pi}{3}\)
∴ General solution is θ = 2nπ ± \(\frac{\pi}{3}\), n ∈ z

(iii) 5 cos² θ + 7 sin² θ = 6
Sol.
Given 5 cos² θ + 7 sin² θ = 6
Dividing by cos² θ we get,
⇒ 5 + 7 tan² θ = 6 sec² θ
⇒ 5 + 7 tan² θ = 6(1 + tan² θ)
⇒ tan² θ – 1 = 0 ⇒ tan² θ = 1
= tan θ = ±1
∴ θ = nπ ± \(\frac{\pi}{4}\), n ∈ Z is the general solution.

(iv) 3 sin⁴ x + cos⁴ x = 1
Answer:
3 sin⁴ x + cos⁴ x = 1
⇒ 3(sin² x)² + cos⁴ = 1
⇒ 3(1 – cos² x)² + cos⁴ x = 1
⇒ 4 cos⁴ x – 6 cos² x + 2 = 0
⇒ 2 cos⁴ x – 3 cos² x + 1 = 0
⇒ 2 cos⁴ x – 2 cos² x – cos² x + 1 = 0
⇒ 2 cos² x (cos²x – 1) – 1(cos² x – 1) = 0
⇒ (2cos² x – 1) (cos² x – 1) = 0

Case (i): 2 cos² x – 1 = 0
⇒ cos² x = \(\frac{1}{2}\) ⇒ cos x = ± \(\frac{1}{\sqrt{2}}\)
General solution is
x = 2nπ ± \(\frac{\pi}{4}\), n ∈ Z if cos x = \(\frac{1}{\sqrt{2}}\) and
x = 2nπ ± \(\frac{3 \pi}{4}\), n ∈ Z for cos x = – \(\frac{1}{\sqrt{2}}\)

Case(ii): cos²x – 1 = 0
⇒ cos²x = 1
cos x = ±1
If cos x = 1 then x = 2nπ, n ∈ Z and
If cos x = – 1 then x = 2nπ ± π, n ∈ Z
∴ General solution is x = 2nπ ± \(\frac{3 \pi}{4}\), n ∈ Z
x = 2nπ ± π, x = 2nπ, and x = 2nπ ± π

II.
Question 1.
Solve the following equations and write general solutions. (SA)
(i) 2 sin² θ – 4 = 5 cos θ
Answer:
2 sin2 θ – 4 = 5 cos θ
⇒ 2(1 – cos² θ) – 5 cos θ – 4 = 0
⇒ 2 cos² θ + 5 cos θ + 4 – 2 = 0
⇒ 2 cos² θ + 5 cos θ + 2 = 0
⇒ 2 cos² θ + 4 cos θ + cos θ + 2 = 0
⇒ 2 cos θ (cos θ + 2) + 1(cos θ + 2) = 0
⇒ (cos θ + 2) (2 cos θ + 1) = 0
cos θ + 2 = 0 is not admissible.
Consider 2 cos θ + 1 = 0 ⇒ cos θ = –\(\frac{1}{2}\), the
principal solution is α = \(\frac{2 \pi}{3}\)
∴ General solution is θ = 2nπ ± \(\frac{2 \pi}{3}\), n ∈ Z

(ii) 2 + √3 sec x – 4 cos x = 2√3
Answer:
2 + \(\frac{\sqrt{3}}{\cos x}\) – 4 cos x = 2√3
⇒ 2 cos x + √3 – 4 cos² x = 2√3 cos x
⇒ 4 cos² x – 2 cos x + 2√3 cos x – √3 = 0
⇒ 2 cos x(2 cos x + √3) – 1(2 cos x + √3) = 0
⇒ (2 cos x – 1) (2 cos x + √3) = 0

Case (i): If 2 cos x – 1 = 0 then cos x = \(\frac{1}{2}\) and the principal solution is α = \(\frac{\pi}{3}\)
∴ General solution is x = 2nπ ± \(\frac{\pi}{3}\), n ∈ Z

Case (ii): 2 cos x + √3 = 0 cos x = – \(\frac{\sqrt{3}}{2}\)
∴ The principal solution is α = 150° = \(\frac{5 \pi}{6}\)
∴ The general solution is
x = 2nπ ± \(\frac{5 \pi}{6}\), n ∈ Z

(iii) 2 cos² θ + 11 sin θ = 7
Answer:
Given equation is 2 cos² θ + 11 sin θ = 7
⇒ 2 (1 – sin² θ) + 11 sin θ = 7
⇒ – 2 sin² θ + 11 sin θ = 5
⇒ 2 sin² θ – 11 sin θ + 5 = 0
⇒ 2 sin² θ – 10 sin θ – sin θ + 5 = 0
⇒ 2 sin² θ (sin θ – 5) – 1(sin θ – 5) = 0
⇒ (2 sin θ – 1) (sin θ – 5) = 0
If sin θ – 5 = 0 then sin θ = 5 is not admissible.
If 2 sin θ – 1 = 0 ⇒ Sin θ = \(\frac{1}{2}\) and the principal solution is α = \(\frac{\pi}{6}\)
∴ General solution is θ = nπ + (- 1)ⁿ \(\frac{\pi}{6}\), n ∈ z

(iv) 6 tan² x – 2 cos² x = cos 2x
Answer:
Given 6 tan² x – 2 cos² x = cos 2x
⇒ 6(sec² x – 1) – 2 cos² x = 2 cos² x – 1
⇒ 6\(\left(\frac{1}{\cos ^2 x}-1\right)\) – 2 cos² x = 2 cos² x – 1
⇒ \(\frac{6}{\cos ^2 x}\) – 4 cos² x – 5 = 0
⇒ 6 – 4 cos⁴ x – 5 cos² x = 0
⇒ 4 cos⁴ x + 5 cos² x – 6 = 0
⇒ 4 cos⁴ x + 8 cos² x – 3 cos² x – 6 = 0
⇒ 4 cos⁴ x (cos² x + 2) – 3(cos⁴ x + 2) = o
⇒ (4 cos² x – 3) (cos²x + 2) = o
If cos² x + 2 = 0 then solution is admissible
and if 4 cos² x – 3 = 0 ⇒ cos²x = \(\frac{3}{4}\)
⇒ cos x = ± \(\frac{\sqrt{3}}{2}\)
and the general solution is x = nπ ± \(\frac{\pi}{6}\), n ∈ Z

(v) 4 cos² θ + √3 = 2(√3 + 1) cos θ
Answer:
4 cos² θ – 2(√3 + 1) cos θ + √3 = 0
⇒ 4 cos² 0 – 2√3 cos θ – 2 cos θ + √3 = 0
⇒ 2 cos θ(2 cos θ – √3) – 1 (2 cos θ – √3) = 0
⇒ (2 cos θ – 1) (2 cos θ – √3) = 0
If cos θ = \(\frac{1}{2}\) then θ = 2nπ ± \(\frac{\pi}{3}\), n ∈ Z is the general solution.
If 2 cos θ – √3 = 0 then cos θ = \(\frac{\sqrt{3}}{2}\)
Hence the general solution in this case is
θ = 2nπ ± \(\frac{\pi}{6}\), n ∈ Z

(vi) 1 + sin 2x = (sin 3x – cos 3x)²
Answer:
1 + sin 2x = sin² 3x. cos² 3x – 2 sin 3x cos 3x
= 1 – sin 6x
⇒ sin 6x + sin 2x = 0
⇒ 2 sin\(\left(\frac{6 x+2 x}{2}\right)\) cos\(\left(\frac{6 x-2 x}{2}\right)\) = 0
⇒ sin 4x cos 2x = 0
If cos 2x = 0 then 2x = (2n + 1) \(\frac{\pi}{2}\)

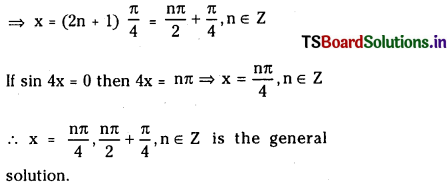
(vii) 2 sin² x + sin² 2x = 2
Answer:
2 sin² x + (2 sin x cos x)² = 2
⇒ sin² x + 2 sin² x cos² x – 1 = 0
⇒ 2 sin² x cos² x – (1 – sin² x) = o
⇒ 2 sin² x cos² x – cos² x = 0
⇒ cos² x (2 sin² x – 1) = o

Case (i): cos² x = 0 ⇒ cos x = 0
⇒ (2n + 1)\(\frac{\pi}{2}\), n ∈ Z is the general solution.

Case (ii): 2 sin²x – 1 = 0 ⇒ sin²x = \(\frac{1}{2}\)

Question 2.
Solve the following equations. (S.A)
(i) √3 sin θ – cos θ = √2 (May 2014)
Answer:
Given √3 sin θ – cos θ = √2
Divide both sides by √3 + 1 = 2

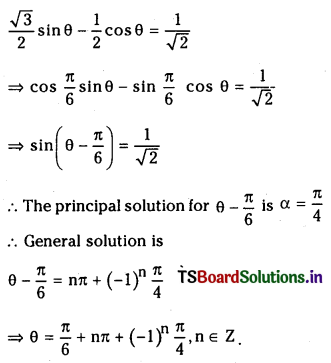
(ii) cot x + cosec x = √3
Answer:
\(\frac{\cos x}{\sin x}+\frac{1}{\sin x}\) = √3
⇒ cos x + 1 = √3 sin x
⇒ √3 sin x – cos x – 1= 0
Divide both sides by √3 + 1 = 2,

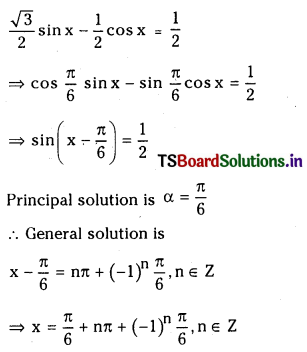
(iii) sin x + √3 cos x = √2 (March 2010)
Answer:
Given sin x + √3 cos x = √2
Divide both sides by √1 + 3 = 2, we get

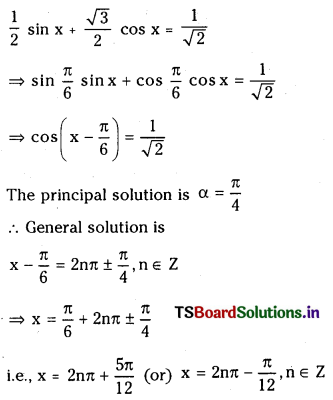
Question 3.
Solve the following equations. (S.A)
(i) tan θ + sec θ = √3, 0 ≤ θ ≤ 2π
Answer:
Given tan θ + sec θ = √3
⇒ \(\frac{\sin \theta}{\cos \theta}+\frac{1}{\cos \theta}\) = √3
⇒ sin θ + 1 = √3 cos θ
⇒ √3 cos θ – sin θ = 1
Divide both sides by √3 + 1 = 2, we get

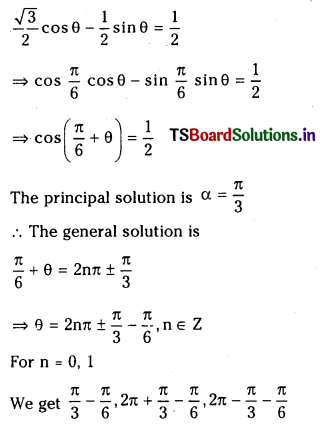
We find that \(\frac{\pi}{6}\) is the only solution in (0, 2π)
Since θ = \(\frac{3 \pi}{2}\) is not admissible since tan θ is not defined for odd multiples of \(\frac{\pi}{2}\).
Also \(\frac{13 \pi}{6}\) ∉ (0, 2π)

(ii) cos 3x + cos 2x = sin \(\frac{3 x}{2}\) + sin \(\frac{x}{2}\), 0 ≤ x ≤ 2π.
Answer:
Given cos 3x + cos 2x = sin \(\frac{3 x}{2}\) + sin \(\frac{x}{2}\)

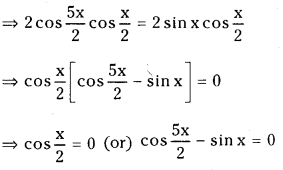
Case (i) cos \(\frac{x}{2}\) = 0 ⇒ \(\frac{x}{2}\) = (2n + 1)\(\frac{\pi}{2}\), n ∈ Z
x = (2n + 1)π, n ∈ Z
Put n = 0, we get {π} is the solution in [0, 2π]

Case (ii):

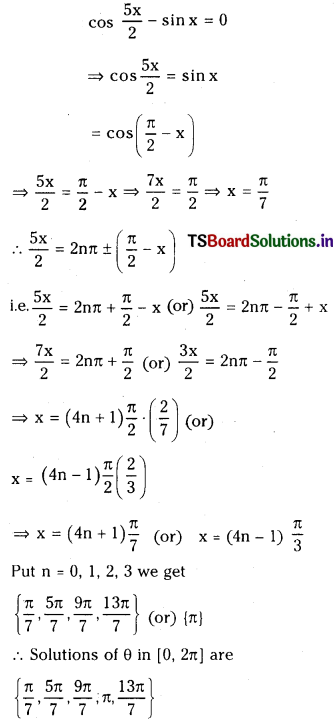
(iii) cot² x – (√3 + 1) cot x + √3 = 0; 0 < x < \(\frac{\pi}{2}\). (Mar. ‘14, ’12)
Answer:
Given cot² x – (√3 + 1) cot x + √3 = o
⇒ cot² x – √3 cot x – cot x + √3 = 0
⇒ cot x (cot x – √3) – 1 (cot x – √3) = 0
⇒(cot x – 1) (cot x – √3) = 0

Case (i) cot x – 1 = 0 = cot x = 1;
Principal solution is α = \(\frac{\pi}{4}\)
∴ General solution is x = nπ + \(\frac{\pi}{4}\), n ∈ Z

Case(ii): cot x – √3 = 0 ⇒ cot x = √3
Principal solution is α = \(\frac{\pi}{6}\)
∴ General solution is x = nπ + \(\frac{\pi}{6}\), n ∈ Z
For n = 0, we get x = \(\frac{\pi}{4}\) and x = \(\frac{\pi}{6}\) and they belong to \(\left(0, \frac{\pi}{2}\right)\)
∴ Solutions are \(\left\{\frac{\pi}{6}, \frac{\pi}{4}\right\}\)

(iv) sec x cos 5x + 1 = 0. 0 < x < 2π.
Answer:
Given sec x cos 5x + 1 = 0. 0 < x < 2π.

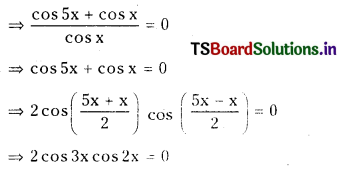
Case (i):

III.
Question 1.
(i) Solve sin x + sin 2x + sin 3x = cos x + cos 2x + cos 3x (E.Q)
Answer:
(sin 3x + sin x) + sin 2x = (cos 3x + cos x) + cos 2x
⇒ 2 sin 2x cos x + sin 2x = 2 cos 2x cos x + cos 2x
⇒ sin 2x (2 cos x + 1) = cos 2x (2cos x + 1)
⇒ (2 cos x + 1) (sin 2x – cos 2x) = 0

Case (i):2 cos x + 1 = 0 ⇒ cos x = – \(\frac{1}{2}\)
Principal solution is α = \(\frac{2 \pi}{3}\)
∴ General solution is x = 2nπ ± \(\frac{2 \pi}{3}\), n ∈ Z

Case (ii): sin 2x – cos 2x = 0 ⇒ tan 2x = 1
Principal solution is α = \(\frac{\pi}{4}\);
∴ General solution is 2x = nπ ± \(\frac{\pi}{4}\)
⇒ x = \(\frac{\mathrm{n} \pi}{2}+\frac{\pi}{8}\), n ∈ Z
∴ General solution is

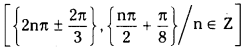
(ii) If x + y = \(\frac{2 \pi}{3}\), and sin x + sin y = \(\frac{3}{2}\) find x and y.
Answer:

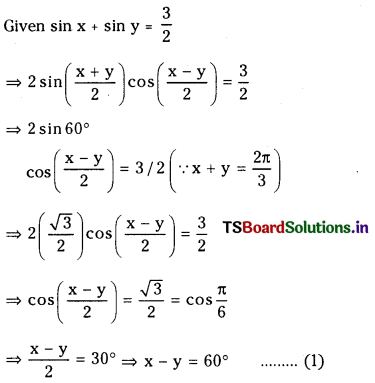
Given x + y = 120° ………………. (2)
Solving x = 90°, y = 30°

(iii) If sin 3x + sin x + 2 cos x = sin 2x + 2 cos 2x, find the general solution.
Answer:
Given sin 3x + sin x + 2 cos x = sin 2x + 2 cos 2x
⇒ 2 sin 2x cos x + 2 cos x = 2 sin x cos x + 2 cos² x
⇒ 2 cos x(sin 2x + 1) = 2 cos x(sin x + cos x)
⇒ 2 cos x[sin 2x + 1 – sin x – cos x] = 0
⇒ cos x = 0 or sin 2x + 1 – sin x – cos x = 0
⇒ cos x = 0 or (sin 2x – sin x) + (1 – cos x) = 0
⇒ cos x = 0 (or) 2 cos \(\frac{3 x}{2}\) sin \(\frac{x}{2}\) + 2 sin²\(\frac{x}{2}\) = 0
⇒ cos x = 0 or 2 sin \(\frac{x}{2}\) (cos \(\frac{3 x}{2}\) + sin \(\frac{x}{2}\)) = 0
⇒ cos x = 0 or sin \(\frac{x}{2}\) = 0 or cos \(\frac{3 x}{2}\) + sin \(\frac{x}{2}\) = 0

Case (i): cos x = 0 ⇒ x = (2n + 1)\(\frac{\pi}{2}\), n ∈ Z

Case (ii): sin \(\frac{x}{2}\) = 0 ⇒ \(\frac{x}{2}\) = nπ ⇒ 2nπ, n ∈ Z

Case (iii):

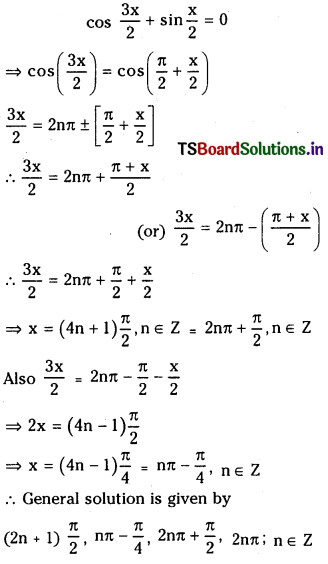
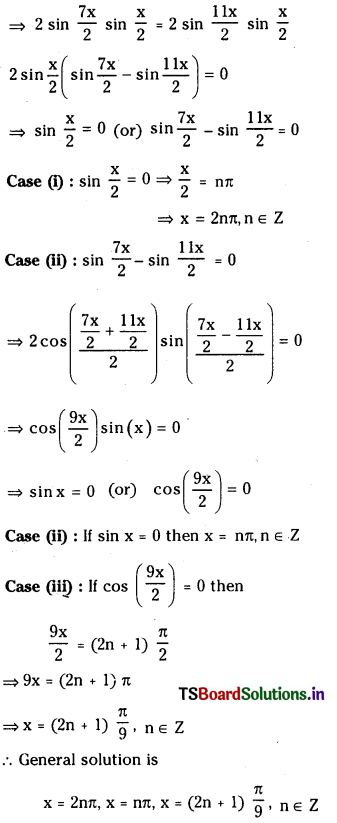
(iv) Solve cos 3x – cos 4x = cos 5x – cos 6x
Answer:
Given cos 3x – cos 4x = cos 5x – cos 6x

Question 2.
Solve the following equations. (E.Q)
(i) cos 2θ + cos 8θ = cos 5θ
Answer:
Given cos 2θ + cos 8θ = cos 5θ
⇒ 2 cos 5θ cos 3θ = cos 5θ
⇒ cos 5θ (2 cos 3θ – 1) = 0

Case (i): cos 5θ = 0 ⇒ 5θ = (2n + 1)\(\frac{\pi}{2}\)
⇒ θ = (2n + 1)\(\frac{\pi}{10}\), n ∈ Z

Case (ii): 2 cos 3θ – 1 = 0
⇒ cos 3θ = \(\frac{1}{2}\)
Principal solution is α = \(\frac{\pi}{3}\)
∴ General solution is 3θ = 2nπ ± \(\frac{\pi}{3}\)
⇒ θ = \(\frac{2 n \pi}{3} \pm \frac{\pi}{9}\), n ∈ Z
∴ General solutions are

(ii) cos θ – cos 7θ = sin 4θ
Answer:
2 sin\(\left(\frac{\theta+7 \theta}{2}\right)\) sin\(\left(\frac{7 \theta-\theta}{2}\right)\) = sin 4θ
⇒ 2sin 4θ sin 3θ = sin 4θ
⇒ sin 4θ (2 sin 3θ – 1) = θ

Case (i): sin 4θ = 0 ⇒ 4θ = nπθ ⇒ \(\frac{\mathrm{n} \pi}{4}\), n ∈ Z

Case (ii): 2 sin 3θ – 1 = 0

(iii) sin θ + sin 5θ = sin 3θ, 0 < θ < π
Answer:
Given sin θ + sin 5θ = sin 3θ
⇒ 2 sin \(\left(\frac{\theta+5 \theta}{2}\right)\) cos \(\left(\frac{\theta-5 \theta}{2}\right)\) = sin 3θ
⇒ 2 sin 3θ cos 2θ = sin 3θ
⇒ sin 3θ (2 cos 2θ – 1) = 0
⇒ sin 3θ = 0 or 2 cos 2θ – 1 = 0

Case (i): sin 3θ = 0 and the general solution is given by 3θ = nπ ⇒ θ = \(\frac{\mathrm{n} \pi}{3}\), n ∈ Z

Case (ii): 2 cos 2θ – 1 = 0
⇒ cos 2θ = \(\frac{1}{2}\) and the principal solution is α = \(\frac{\pi}{3}\)

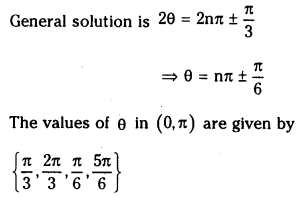
Question 3.
(i) If tan pθ = cot qθ and p ≠ – q, show that the solutions are in A.P. with common difference \(\frac{\pi}{\mathbf{p}+\mathbf{q}}\). (E.Q)
Answer:

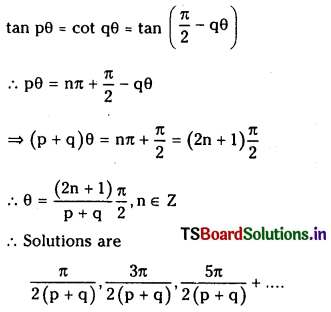
The solutions form an arithmetical progression with \(\frac{2 \pi}{2(p+q)}=\frac{\pi}{p+q}\) as common difference.

(ii) Show that the solutions of cos pθ = sin qθ form two series each of which is an A.P.
Find also the common difference of each A.P. (p ≠ ±q)
Answer:
cos pθ = sin qθ ⇒ cos pθ – sin qθ = 0

(iii) Find the number of solutions of the equation tan x + sec x = 2 cos x; cos x ≠ 0, lying in the interval (0, π).
Answer:
Given tan x + sec x = 2 cos x
⇒ \(\frac{\sin x}{\cos x}+\frac{1}{\cos x}\) = 2 cos x
⇒ 1 + sin x = 2 cos 2x
⇒ 1 + sin x = 2 (1 – sin² x)
⇒ 2 sin² x + sin x – 1 = 0
⇒ 2 sin² x + 2 sin x – sin x – 1 = 0
⇒ 2 sin x(sin x + 1) – 1 (sin x + 1) = 0
⇒ (2 sin x – 1) (sin x + 1) = 0

Case (i): 2 sin x – 1 = 0 ⇒ sin x = \(\frac{1}{2}\),
Principal solution is α = \(\frac{\pi}{6}\); and
general solution is x = nπ + (- 1)ⁿ \(\frac{\pi}{6}\)
solutions in (0, π) are \(\left\{\frac{\pi}{6}, \frac{5 \pi}{6}\right\}\)

Case (ii) sin x + 1 ⇒ sin x = – 1
⇒ x = – \(\frac{\pi}{2}\) (or) \(\frac{3 \pi}{2}\)
∴ Number of solutions in (0, π) for the given equation is two.

(iv) Solve sin 3α = 4 sin α sin(x + α) sin(x – α) where α ≠ nπ, n ∈ Z.
Answer:
Given
sin 3α = 4 sin α sin(x + α)sin(x – α)
= 4 sin α(sin² x – sin² α)
= 3 sin α – 4 sin³ α
= 4 sin α (sin²x – sin²α)
⇒ 3 sin α = 4 sin α sin² x
⇒ sin α (3 – 4 sin² x) = 0
⇒ 3 – 4 sin² x = 0 ⇒ sin²x = \(\frac{3}{4}\)
⇒ sin x = ±\(\frac{\sqrt{3}}{2}\)
∴ General solution is
x = nπ + (- 1)ⁿ (±\(\frac{\pi}{3}\)), n ∈ Z.
Since the principal solution is α = ±\(\frac{\pi}{3}\)

Question 4.
(i) If tan (π cos θ) = cot (π sin θ), then prove that cos \(\left(\theta-\frac{\pi}{4}\right)\) = ± \(\frac{1}{2 \sqrt{2}}\) (E.Q.)
Answer:
Given that tan (π cos θ) = cot (π sin θ) = tan (\(\frac{\pi}{2}\) – π sin θ)

(ii) Find the average of θ if cos θ + sin θ is positive.
Answer:

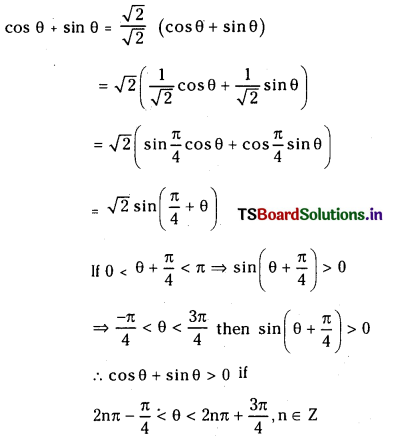
Question 5.
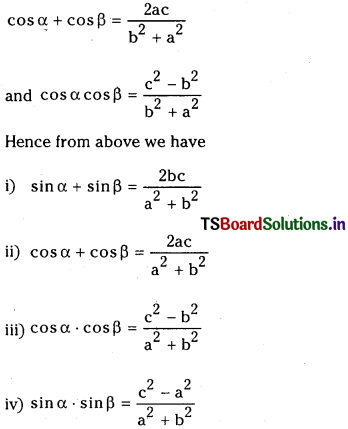
If α, β are the solutions of the equation a cos θ + b sin θ = c, where a, b, c ∈ R and If a² + b² >0, cos α ≠ cos β and sin α ≠ sin β then show that (E.Q)
(i) sin α + sin β = \(\frac{2 b c}{a^2+b^2}\)
(ii) cos α + cos β = \(\frac{2 a c}{a^2+b^2}\)
(iii) cos α . cos β = \(\frac{c^2-b^2}{a^2+b^2}\)
(iv) sin α . sin β = \(\frac{c^2-a^2}{a^2+b^2}\)
Answer:
Given a cos θ + b sin θ = c
⇒ a cos θ = c – b sin θ
⇒ a² cos² θ = c² – 2bc sin θ + b² sin² θ
⇒ a² (1 – sin²θ) = c² – 2bc sin θ + b² sin² θ
⇒ (a² + b²)sin²θ – 2bc sin θ + (c² – a²) = 0
This is a quadratic in sin θ and let the roots be sin α and sin β. Then
sin α + sin β = \(\frac{2 b c}{a^2+b^2}\)
sin α . sin β = \(\frac{c^2-a^2}{a^2+b^2}\)
Also b sin θ = c – a cos θ
⇒ b² sin² θ = c² – 2ac cos θ + a² cos² θ
⇒ b² (1 – cos² θ) = c² – 2ac cos θ + a² cos² θ
⇒ (b² + a²)cos² θ – 2ac cos θ + (c² – b²) = 0
This is a quadratic in cos θ and let cos α, cos β be the roots. Then

Question 6.
(i) Find the common roots of the equations cos 2x + sin 2x cot x and 2 cos² x + cos² 2x = 1. (E.Q)
Answer:
Let tan x = A and given that
cos 2x + sin 2x = cot x

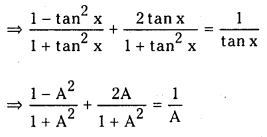
⇒ (1 – A² + 2A)A = (1 + A²)
⇒ A – A³ + 2A² = A² + 1
⇒ A³ – A² – A + 1 = 0
∴ A = 1 satisfy the equation.
By synthetic division

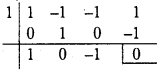
∴ A³ – A² – A + 1 = 0
⇒ (A – 1)(A³ – 1) = 0
⇒ A = 1, A = – 1
When A = ±1 then tan x = ±1.
⇒ x = nπ ± \(\frac{\pi}{4}\) ……………….. (1)
Also given 2 cos² x + cos² 2x = 1
⇒ (2 cos² x – 1) + cos² 2x = 0
⇒ cos 2x(1 + cos 2x) = 0
⇒ cos 2x = 0 (or) cos 2x = – 1

Case (i): When cos 2x = 0 then
2x = (2n + 1) \(\frac{\pi}{2}\)
⇒ x = (2n + 1)\(\frac{\pi}{4}\), n ∈ Z ……………….. (2)
From (1) and (2) we have
(2n + 1)\(\frac{\pi}{4}\), n ∈ Z is the common root for the given two trigonometric equations.

(ii) Solve the equation
\(\sqrt{6-\cos x+7 \sin ^2 x}\) + cos x = 0
Answer:
Given
\(\sqrt{6-\cos x+7 \sin ^2 x}\) + cos x = 0
∴ 6 – cos x + 7 sin² x = cos²x
⇒ 7(1 – cos²x – cos x + 6 = cos²x
⇒ 8 cos x + cos x – 13 = 0
∴ cos x = \(\frac{-1 \pm \sqrt{1+4(8)(13)}}{16}\)
= \(\frac{-1 \pm \sqrt{417}}{16}\)
The solution is not possible since – 1 ≤ cos x ≤ 1

(iiii) It |tan x| = tan x + \(\frac{1}{\cos x}\) and x ∈ [0, 2π], find the value of x.
Answer:
|tan x| = tan x if x lies either in 1 or in III quadrants.
∴ tan x = tan sec x
⇒ sec x = 0 since sec x ∉ (- 1, 1)
Also |tan x| = – tan x If x lies in either II or IV quadrants.
∴ – tan x = tan x + sec x
⇒ – 2 tan x = sec x