TS Inter 1st Year Maths 1A Solutions Chapter 1 Functions Ex 1(a)

Students must practice these TS Intermediate Maths 1A Solutions Chapter 1 Functions Ex 1(a) to find a better approach to solving the problems.

TS Inter 1st Year Maths 1A Functions Solutions Exercise 1(a)

I.
Question 1.
If the function f is defined by

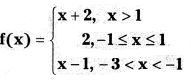
then find the values of
(i) f(3)
(ii) f(0)
(iii) f(-1.5)
(iv) f(2) + f(- 2)
(v) f(- 5)
Answer:
(i) f(3), For x > 1; f(x) = x + 2
f(3) = 3 + 2 = 5

(ii) f(0), For – 1 ≤ x ≤ 1; f(0) = 2

(iii) f(-1.5), For – 3 < x < – 1; f(x) = x – 1
∴ f(-1.5) = -1.5- 1 = – 2.5

(iv) f(2) + f(-2); For x > 1, f(x) = x + 2
∴ f(2) = 2 + 2 = 4
For – 3 < x < – 1;
f(x) = x – 1
f(-2) = -2 – 1 = -3
f(2) + f (-2) = 4 – 3 = 1

(v) f(-5); is not defined such domain of ‘f’ is {x / x > – 3].

Question 2.
If f : R {0} → R defined by f(x) = x³ – \(\frac{1}{x^3}\), then show that f(x) + f\(\left(\frac{1}{x}\right)\) = 0.
Answer:
Given f(x) = x³ – \(\frac{1}{x^3}\)

Question 3.
If f: R → R defined by f(x) = \(\frac{1-x^2}{1+x^2}\), then show that f(tan θ) = cos 2θ
Answer:
Given f(x) = \(\frac{1-x^2}{1+x^2}\) ∀ x ∈ R

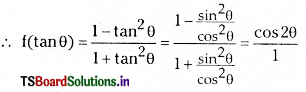
= cos 2θ

Question 4.
If f: R – (±1) → R is defined by f(x) = log\(\left|\frac{1+x}{1-x}\right|\), then show that f\(\left(\frac{2 x}{1+x^2}\right)\) = 2f(x).
Answer:
Given f: R – (±1) → R defined by f(x) = log\(\left|\frac{1+x}{1-x}\right|\)

Question 5.
If A = (-2, -1, 0, 1, 2) and f : A → B is a surjection defined by f(x) = x² + x + 1, then find B. (May 2014)
Answer:
A = {-2,-1,0,1,2} and f: A → B is a surjection and f(x) = x² + x + 1;
∴ f(-2) = (-2)² + (-2) + 1=3,
f(-1) = (-1)² + (-1) + 1 = 1
f(0) = 0² + 0 + 1 = 1
f(1) =1² + 1 + 1 = 3
f(2) = 2² + 2 + 1 = 7
∴ B = f(A) = (1, 3, 7)

Question 6.
If A = {1, 2, 3, 4} and f: A → R is a function defined by f(x) = \(\frac{x^2-x+1}{x+1}\), then find the range of f.
Answer:
Given A = {1, 2, 3, 4} and f(x) = \(\frac{x^2-x+1}{x+1}\)

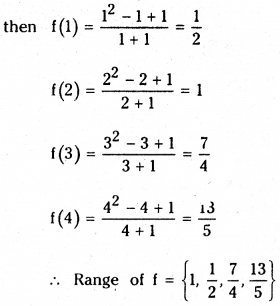
Question 7.
If f (x + y) = f (xy) ∀ x, y ∈ R, then prove that f is a constant function.
Answer:
Given f (x + y) = f(x y) ∀ x, y ∈ R Suppose x = y = 0 then
f(0 + 0) = f(0 x 0)
⇒ f(0) = f(0) ………………..(1)
Suppose x = 1, y = 0 then then f (1 + 0) = f(1 x 0)
⇒ f(D = f (0) ……………(2)
Suppose x = 1, y = 1 then f (1 + 1) = f(1 x 1)
⇒ f(2) = f(1) …………….. (3)
f(0) = f(1) = f(2)
= f(0) = f(2)
Similarly f(3) = f(0), f(4) = f(0) …………. f(n) = f(0)
∴ f is a constant function.

II.
Question 1.
If A = {x / – 1 ≤ x ≤ 11, f(x) = x², g(x) = x³ Which of the following are surjections
(i) f : A → A
(ii) g : A → A.
Answer:
i) Given A {x / – 1 ≤ x ≤ 1}, f(x) = x²
and f : A → A
Suppose y ∈ A
then x² = y ⇒ x = ± √y
If x = √y and if y = – 1 then x = √-1 ∈ A
f : A → A is not a surjection.

ii) Given A = {x/-1 ≤ x ≤ 1), g(x) = x³
and g : A → A
Suppose ye A then x² = y ⇒ x = \(\sqrt[3]{y}\) ∈ A
If y = -1 then x = -1 ∈ A
y = 0 then x = 0 ∈ A
y = 1 then x = 1 ∈ A
g : A → A is a surjection.

Question 2.
Which of the following are injections or surjections or Bisections ? Justify your answers.
i) f : R → R defined by f(x) = \(\frac{2 x+1}{3}\)
Answer:
Given f(x) = \(\frac{2 x+1}{3}\)
Let a₁, a₂ ∈ R
∴ f(a₁) = f(a₂)
⇒ \(\frac{2 \mathrm{a}_1+1}{3}=\frac{2 \mathrm{a}_2+1}{3}\)
⇒ 2a₁ + 1 = 2a₂ + 1
⇒ a₁ = a₂
f(a₁) = f(a₂) ⇒ a₁ = a₂ ∀ a₁, a₂ ∈ R
f(x) = \(\frac{2 x+1}{3}\) is an injection.

Suppose y ∈ R (codomain of f) then
y = \(\frac{2 x+1}{3}\) ⇒ x = \(\frac{3 y-1}{2}\)
Then f(x) = f\(\left(\frac{3 y-1}{2}\right)=\frac{\frac{2(3 y-1)}{2}+1}{3}\) = y
f is a surjection f: R → R defined by f(x) = \(\frac{2 x+1}{3}\) is a bijection.

ii) f : R → (0, ∞) defined by f(x) = 2^x
Answer:
Let a₁, a₂ ∈ R then f(a₁) = f(a₂)
⇒ 2^a₁ = 2^a₂
⇒ a₁ = a₂ ∀ a₁, a₂ ∈ R
f(x) = 2x, f: R → (0, ∞) is injection.
Let y ∈ (0, ∞) and y = 2^x ⇒ x = log₂ y
then f(x) = 2^x = 2 log2^y = y
∴ f is a surjection.
Since f is injection and surjection, f is a bijection.

iii) f : (0, ∞) → R defined by f(x) = log_ex.
Answer:
Let x₁, x₂ ∈ (0, ∞)and = log_ex. then f(x₁) = f(x₂)
⇒ log_ex₁ = log_ex₂ ⇒ x₁ = x₂
∴ f(x₁) = f(x₂)
x₁ = x₂ and f is injection.

Let y ∈ R then y = log_ex ⇒ x = e^y
f(x) = log_ex = log_ee^y = y and f is a surjection.
Since f is both injective and surjective, f is a bijection.

iv) f : [0, ∞) → [0, ∞) defined by f(x) = x²
Answer:
Let x₁, x₁ ∈ [0, ∞) given f(x) = x²
f(x₁) = f(x₂)
⇒ x₁ = x₂
x₁ = x₂ (∵ x₁, x₂ > 0)
f(x) = x²,
∴ f: [0, ∞) → [0, ∞) is an injection.

Let y ∈ [0, ∞)then y = x² ⇒ x = √y (∵ y > 0)
f(x) = x² = (√y)² = y
and f is a surjection
∴ f is a bijection.

v) f : R → [0, ∞) defined by f(x) = x²
Answer:
Let x₁ x₂ ∈ R and f(x) = x²
∴ f(x₁) = f(x₂)
⇒ x₁² = x₂²
⇒ x₁ = ±x₂ (∵ x₁, x₂ ∈ R)
f is not an injection
Let y ∈ [0, ∞] then y = x² ⇒ x = ±√y
where y ∈ [0, ∞] then f(x) = x² = (√y )² = y.
∴ f is a surjection.
Since f is not injective and only surjective, we say that f is not a bijection.

vi) f : R → R defined by f(x) = x²
Answer:
Let x₁ x₂ ∈ R then f(x₁) = f(x₂)
⇒ x₁² = x₂²
⇒ x₁ = ± x₂ (∵ x₁, x₂ ∈ R)
f(x) is not an injection.
Let y ∈ R then y = x²
⇒ x = ±√y
For elements that belong to (-∞, 0).
codomain R of f has no pre-image in f.
∴ f is not a surjection.
Hence f is not a bijection.

Question 3.
If g = 1(1,1), (2, 3), (3, 5), (4, 7)) is a function from A = {1, 2, 3, 4} to B = {1, 3, 5, 7}. If this is given by the formula g(x) = ax + b then find a and b.
Answer:
A = {1, 2, 3, 4}, B = {1, 3, 5, 7}
g = {(1, 1), (2, 3), (3, 5), (4, 7)}
∵ g(1) = 1, g(2) = 3, g(3) = 5, g(4) = 7
Hence for an element a ∈ A f ∃ b ∈ B such that g : A → B is a function.
Given g(x) = ax + b ∀ x ∈ A
g(1) = a + b = 1
g(2) = 2a + b = 3
solving a = 2, b = -1

Question 4.
If the function f : R → R defined by f(x) = \(\frac{3^x+3^{-x}}{2}\), then show that f (x+y) + f (x-y) = 2 f(x) f(y).
Answer:

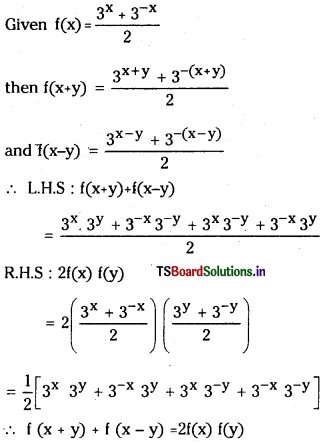
Question 5.
If the function f : R → R defined by f(x) = \(\frac{4^x}{4^x+2}\), then show that f (1 – x) = 1 – f(x) and hence reduce the value of f\(\left(\frac{1}{4}\right)\) + 2f\(\left(\frac{1}{2}\right)\) + f\(\left(\frac{3}{4}\right)\).
Answer:

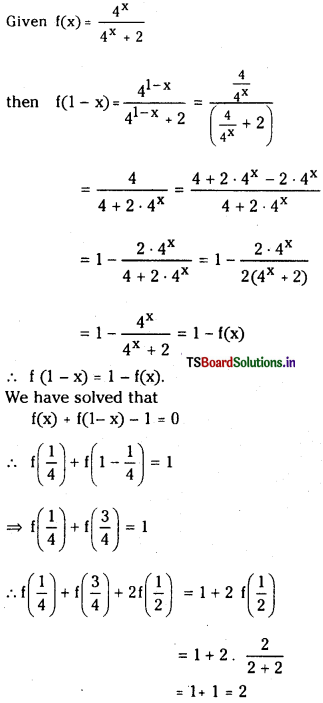
Question 6.
If the function f : {-1, 1} → {0, 2} defined by f(x) = ax + b is a suijection, then find a and b.
Answer:
Since f: {-1, 1} → {0, 2} and f(x) = ax + b is a surjection.
Given f (-1) = 0, f (1) = 2 (or) f (-1) = 2, f (1)=0
Case I : f (-1) = 0, f (1) = 2
∴ – a + b = 0, a + b = 2
Solving b =1 , a = 1

Case II : f (-1) = 2, and f (1) = 0
then – a + b = 2 and a + b = 0
Solving b = 1, a = -1
Hence a = + 1 and b = 1

Question 7.
If f(x) = cos (log x), then show that f\(\left(\frac{1}{x}\right)\) f\(\left(\frac{1}{y}\right)\) – \(\left(\frac{1}{2}\right)\)[f\(\left(\frac{x}{y}\right)\) + f(xy)] = 0
Answer:
Given f(x) = cos(log x)
then f\(\left(\frac{1}{x}\right)\) = cos(log\(\left(\frac{1}{x}\right)\))
= cos(-log x) = cos(log x) (∵ log 1 = 0)
Similarly f\(\left(\frac{1}{x}\right)\) = cos(log y)
f\(\left(\frac{x}{y}\right)\) = cos(log\(\left(\frac{x}{y}\right)\)) = cos(log x – log y)
f(xy) = cos (log xy) = cos [log x + log y]
f\(\left(\frac{x}{y}\right)\) + f(x y) = cos(log x – log y) + cos (log x + log y)
= 2 cos (log x) cos (log y) (∵ cos (A – B) + cos (A + B))
= 2 cos A cos B
f\(\left(\frac{1}{x}\right)\) f\(\left(\frac{1}{y}\right)\) – \(\left(\frac{1}{2}\right)\)[f\(\left(\frac{x}{y}\right)\) + f(xy)] = cos (log x) cos (log y) – \(\frac{1}{2}\) [2cos (log x) cos (logy)]
= 0