Students must practice this TS Intermediate Maths 2A Solutions Chapter 4 Theory of Equations Ex 4(d) to find a better approach to solving the problems.
TS Inter 2nd Year Maths 2A Solutions Chapter 4 Theory of Equations Ex 4(d)
I.
Question 1.
Find the algebraic equation whose roots are 3 times the roots of x3 + 2x2 – 4x + 1 = 0.
Solution:
Given equation is x3 – 2x2 – 4x + 1 = 0 ……………(1)
Let f(x) = x3 + 2x2 – 4x + 1
The equation whose roots are 3 times the roots of f(x) = 0 is given by f(\(\frac{x}{3}\)) = 0.
i.e., \(\left(\frac{x}{3}\right)^3+2\left(\frac{x}{3}\right)^2-4\left(\frac{x}{3}\right)\) + 1 = 0
⇒ \(\frac{x^3}{27}+\frac{2 x^2}{9}-\frac{4 x}{3}\) + 1 = 0
⇒ x3 + 6x2 – 36x + 27 = 0.
Question 2.
Find the algebraic equation whose roots are 2 times the roots of x5 – 2×4 + 3×3 – 2×2 + 4x + 3 = 0.
Solution:
Given equation is x5 – 2x4 + 3x3 – 2x2 + 4x + 3 = 0 ……..(1)
Let f(x) = x5 – 2x4 + 3x3 – 2x2 + 4x + 3
The equation whose roots are 2 times the roots of f(x) = 0 is given by f(\(\frac{x}{2}\)) = 0
i.e., \(\left(\frac{x}{2}\right)^5-2\left(\frac{x}{2}\right)^4+3\left(\frac{x}{2}\right)^3-2\left(\frac{x}{2}\right)^2+4\left(\frac{x}{2}\right)+3\) = 0
⇒ x5 – 4x4 + 12x3 – 16x2 + 64x + 96 = 0.
![]()
Question 3.
Find the transformed equation whose roots are the negatives of the roots of x4 + 5×3 + lix + = 0.
Solution:
Given equation is x4 + 5x3 + 11x + 3 = 0
Let f(x) = x4 + 5x3 + 11x + 3
The transformed equation whose roots are the negatives of the roots of f(x) = 0 is
f(- x) = 0.
i.e., (- x)4 + 5(- x)3 + 11 (- x) + 3 = 0
⇒ x4 – 5x3 – 11x + 3 = 0.
Question 4.
Find the transformed equation whose roots are the negatives of the root of x7 + 3x5 + x3 – x2 + 7x + 2 = 0.
Solution:
Given equation is
x7 + 3x5 + x3 – x2 + 7x + 2 = 0
Let f(x) = x7 + 3x5 + x3 – x2 + 7x + 2
The transformed equation whose roots are the negatives of the roots of f(x) = 0 is
f(- x) = 0.
i.e., (- x)2 + 3(- x)5 + (- x)3 – (- x)2 + 7(- x) + 2 = 0
x7 + 3x5 + x3 + x2 + 7x – 2 = 0.
Question 5.
Find the polynomial equation whose roots are the reciprocals of the roots of x4 – 3x3 + 7x2 + 5x – 2 = 0.
Solution:
Given equation is x4 – 3x3 + 7x2 + 5x – 2 = 0 ………….(1)
Let f(x) = x4 – 3x3 + 7x2 + 5x – 2
The polynomial equation whose roots are the reciprocals of the roots of (1) is given by
f(\(\frac{1}{x}\)) = 0
i.e., \(\left(\frac{1}{x}\right)^4-3\left(\frac{1}{x}\right)^3+7\left(\frac{1}{x}\right)^2+5\left(\frac{1}{x}\right)\) – 2 = 0
⇒ 1 – 3x + 7x2 + 5x3 – 2x4 = 0
⇒ 2x4 – 5x3 – 7x2 + 3x – 1 = 0.
![]()
Question 6.
Find the polynomial equation whose roots are the reciprocals of the roots of x5 + 11x4 + x3 + 4x2 – 13x + 6 = 0.
Solution:
Given equation is x5 + 11x4 + x3 + 4x2 – 13x + 6 = 0 …………(1)
Let f(x) = x5 + 11x4 + x3 + 4x2 – 13x + 6
The polynomial equation whose roots are the reciprocals of the roots of f(x) = 0 is
f(\(\frac{1}{x}\)) = 0
i.e., \(\left(\frac{1}{x}\right)^5+11\left(\frac{1}{x}\right)^4+\left(\frac{1}{x}\right)^3\) + 6 = 0
⇒ 6x5 – 13x4 + 4x3 + x2 + 11x + 1 = 0.
II.
Question 1.
Find the polynomial equation whose roots are the squares of the roots of x4 + x3 + 2x2 + x + 1 = 0.
Solution:
Given equation is x4 + x3 + 2x2 + x + 1 = 0
Let f(x) = x4 + x3 + 2x2 + x + 1
The polynomial equation whose roots are squares of the roots of f(x) = 0 is f (√x) = 0.
i.e.. (4√x)4 + (√x)3 + 2(√x)2 + √x + 1 = 0
⇒ x2 + 2x + 1 = √x (x + 1)
⇒ (x + 1)2 = √x (x + 1)
⇒ (x + 1)4 = x (x + 1)2
⇒ x4 + 4x3 + 6x2 + 4x + 1 = x(x2 +2x + 1)
⇒ x4 + 3x3 + 4x2 + 3x + 1 = 0 is the required equation.
![]()
Question 2.
Form the polynomial equation whose roots are the squares of the roots of x3 + 3x2 – 7x + 6 = 0.
Solution:
Given equation is x3 + 3x2 – 7x + 6 = 0 …… (1)
Let f(x) = x3 + 3x2 – 7x + 6
The polynomial equation whose roots are the squares of the roots of f(x) = 0 is f(√x) = 0.
i.e., (√x)3 + 3(√x)2 – 7(√x) + 6 = 0
⇒ x√x – 7√x = – 3x – 6
⇒ √x (x – 7) = – (3x + 6)
⇒ x (x2 + 49 – 14x) = 9x2 + 36 + 36x
⇒ x3 – 23x2 + 13x – 36 = 0 is the required equation.
Question 3.
Form the polynomial equation whose roots are the cubes of the roots of x3 + 3x2 + 2 = 0.
Solution:
Given equation is x3 + 3x2 + 2 = 0 …………. (1)
Let f(x) = x3 + 3x2 + 2
The polynomial equation whose roots are the cubes of the roots of f(x) = 0 is f(\(\sqrt[3]{x}\)) = 0.
i.e., \((\sqrt[3]{x})^3\) + 3 (\((\sqrt[3]{x})^2\)) + 2 = 0
⇒ x + 2 = – 3x2/3
⇒ (x + 2)3 = – 27 (x2)
⇒ x3 + 6x2 + 12x + 8 = – 27x2
⇒ x3 + 33x2 + 12x + 8 = 0 is the required equation.
![]()
III.
Question 1.
Find the polynomial equation whose roots are the translates of those of the equation – 5x3 + 7x2 – 17x + 11 = 0 by – 2.
Solution:
Given equation is
x4 – 5x3 + 7x2 – 17x + 11 = 0 ………(1)
Let f(x) = x4 – 5x3 + 7x2 – 17x + 11
The polynomial equation, whose roots are
the translates of those of the f(x) = 0 by – 2 is f(x + 2) = 0.
Suppose that
f(x + 2) = A0x4 + A1x3 + A2x2+ A3x + A4
By synthetic division, the coefficients A0, A1, A2, A3 and A4 are obtained as follows.

∴ The roots ol the equation x4 – 3x3 + x2 – 17x + 19 = 0 are the translates of the roots of the given equation by – 2.
Question 2.
Find the polynomial equation whose roots are the translates of those of the equation x5 – 4x4 + 3x2 – 4x + 6 = 0 by – 3.
Solution:
Given equation is x5 – 4x4 + 3x2 – 4x 4 6 = 0 ………….(1)
Let f(x) = x5 – 4x4 + 3x2 – 4x + 6
The polynomial equation, whose roots are the translates of those of the f(x) = 0 by – 3 is f(x + 3) = 0.
Suppose that f(x + 3) = A0x5 + A1x4 + A2x3 + A3x2 + A4x + A5
By synthetic division, the coefficients A0, A1, A2, A3, A4 and A5 are obtained as follows.
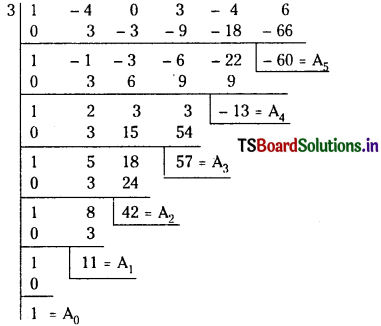
∴ The roots of the equation x5 + 11x4 + 42x3 + 57x2 – 13x – 60 = 0 are the translates of the roots of the given equation by – 3.
![]()
Question 3.
Find the polynomial equation whose roots are the translates of the roots of the equation x4 – x3 – 10x2 + 4x + 24 = 0 by 2.
Solution:
Given equation is x4 – x3 – 10x2 + 4x + 24 = 0
Let f(x) = x4 – x3 – 10x2 + 4x + 24
The polynomial equation whose roots are trans-lates of those of the f(x) = 0 by 2 is f(x – 2) = 0.
Suppose that f(x – 2) = A0x4 + A1x3 + A2x2 + A3x + A4
By synthetic division, the coefficients A0, A1, A2, A3, A4 are obtained as follows – 2.
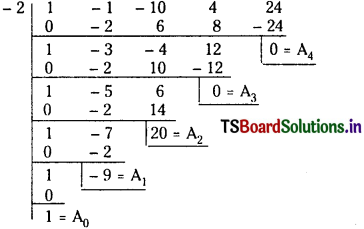
∴ The roots of the equation x4 – 9x3 + 20x2 = 0 are the translates of the roots of the given equati on by 2.
Question 4.
Find the polynomial equation whose roots are the translates of the roots of the equation 3x5 – 5x3 + 7 = 0 by 4.
Solution:
Given equation is 3x5 – 5x3 + 7 = 0
Let f(x) = 3x5 – 5x3 + 7
The polynomial equation whose roots are the translates of those of the f(x) = 0 by 4 is f(x – 4) = 0.
Suppose that
f(x – 4) = A0x5 + A1x4 + A2x3 + A3x2 + A4x + A5
By synthetic division, the coefficients A0, A1, A2, A3, A4, A5 are obtained as follows.
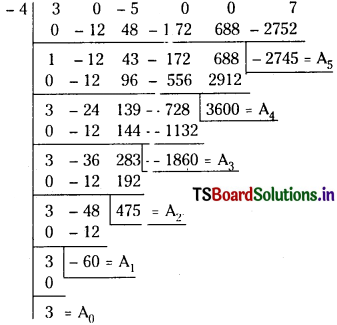
∴ The roots of the equation 3x5 – 60x4 + 475x2 – 1860x3 + 3600x – 2745 = 0 are the translates of the roots of the given equation by 4.
![]()
Question 5.
Transform e jach of the following equations into ones i n which the coefficients of the second hig >hest power of x is zero and also find their transformed equations.
i) x3 – 6x2 + 10x – 3 = 0
ii) x4 + 4x3 + 2x2 – 4x – 2 = 0
iii) x3 – 6x2 + 4x – 7 = 0
iv) x3 + 6x2+ 4x + 4 = 0
Solution:
i) Given equation is x3 – 6x + 10x – 3 = 0
Let f(x) = x3 – 6x2 + 10x – 3
We have to find ’h’ so that the coefficient of the Second highest power of x in f(x + h) is zero.
i.e., Coefficient of x2 in f(x + h) is zero,
f (x + h) = (x + h)3 – 6 (x + h)2 + 10 (x + h) – 3 Coefficients of x2 in f(x + h) is 3h – 6.
We choose ‘h‘ such that 3h – 6 = 0 i.e., h = 2
∴ f (x + 2) = (x + 2)3 – 6 (x + 2)2 + 10 (x + 2) – 3
= x3 + 6x2 + 12x + 8 – 6 (x2 + x + 4) + 10x + 20 – 3
= x3 – 2x + 1
∴ x3 – 2x + 1 = 0 is the required equation.
ii) Given equation is
x4 + 4x3 + 2x2 – 4x – 2 = 0
Let f(x) = x4 + 4x3 + 2x2 – 4x – 2
We have to find ‘h’ so that the coefficient of the second highest power of x in f(x + h) is zero.
i.e., coefficient of x2 in f(x + h) is zero,
f (x + h) = (x + h)4 + 4 (x + h)3 + 2 (x + h)2 , – 4 (x + h) – 2
Coefficient of x2 in f(x + h) is 4h + 4.
We choose ‘h‘ such that 4h + 4 = 0 i.e., h = – 1
∴ f(x – 1) = (x – 1)4 + 4 (x – 1)3 + 2 (x – 1)2 – 4 (x – 1) – 2
= (x4 – 4x3 + 6x2 – 4x + 1) + 4 (x3 – 3x2 + 3x – 1) + 2 (x2 – 2x + 1) – 4 (x – 1) – 2
= x4 – 4x2 + 1
∴ x4 – 4x2 + 1 = 0 is the required equation.
iii) Given equation is x3 – 6x2 + 4x – 7 = 0
Let f(x) = x3 – 6x2 + 4x – 7
We have to find ‘h’ so that the coefficient of the second highest power of x in f(x + h) is zero.
i.e., coefficient of x2 in f(x + h) is zero.
f(x + h) = (x + h)3 – 6 (x + h)2 + 4 (x + h) – 7
Coefficient of x2 in f(x + h) is 3h – 6
We choose ‘h’ such that 3h – 6 = 0 i.e., h = 2
∴ f(x + 2) = (x + 2)3 – 6 (x + 2)2 + 4 (x + 2) – 7
= (x3 + 6x2 + 12x + 8) – 6 (x2 + 4x + 4) + 4 (x + 2) – 7
= x3 – 8x – 15
∴ x3 – 8x – 15 = 0 is the required equation.
iv) Given equation is x3 + 6x2 + 4x + 4 = 0
Let f(x) = x3 + 6x2 + 4x + 4
We have to find ’h’ so that the coefficient of the second highest power of x in f(x + h) is zero, i.e., coefficient of x2 in f(x + h) is zero.
f(x + h) = (x + h)3 + 6(x + h)2 + 4(x + h) + 4
Coefficient of x2 in 1(x + h) is 3h + 6.
We have to choose ‘h’ such that 3h + 6 = 0 i.e., h = – 2
∴ f(x – 2)= (x – 2)3 + 6(x – 2)2 + 4(x – 2) + 4
= (x3 – 6x2 + 12x – 8) + 6 (x2 – 4x + 4) + 4 (x – 2) + 4
= x3 – 8x + 12
∴ x3 – 8x + 12 = is the required equation.
![]()
Question 6.
Transform each of the following equations into ones in which the coefficients of the third highest power of x is zero.
i) x4 + 2x<sup3 – 12x2 + 2x – 1 = 0
ii) x3 + 2x2 + x + 1 = 0
Solution:
i) Given equation is
x4 + 2x3 – 12x2 + 2x – 1 = 0
Let f(x) = x4 + 2x3 – 12x2 + 2x – 1
We have to find h’ so that the coefficient of the third highest power of ‘x’ in f(x + h) is zero.
i.e., Coefficient of x2 in [(x + h) is zero.
f(x + h) = (x + h)4 + 2 (x + h)3 – 12 (x + h)2 + 2 (x + h) – 1
Coefficient of x3 in 1(x + h) is 6h2 + 6h – 12
We have to choose ‘h’ such that
6h2 + 6h – 12 = 0
⇒ (h + 2) (h – 1) = 0
⇒ h = – 2 or 1.
Case – (I):
When h = – 2
f(x – 2) = (x – 2)4 + 2 (x – 2)3 – 12(x – 2)2 + 2 (x – 2) – 1
= x4 – 8x3 + 24x2 – 32x + 16 + 2(x3 – 6x2 + 12x – 8) – 12(x2 – 4x + 4) + 2(x – 2) – 1
= x4 – 6x3 + 42x – 53
∴ Tranformed equation is x4 – 6x3 + 42x – 53 = 0.
Case-(ii):
When h = 1
f(x + 1) = (x + 1)4 + 2(x + 1)3 – 12(x + 1)2 + 2 (x + 1) – 1
= (x4 + 4x3 + 6x2 + 4x + 1) + 2(x3 + 3x2 + 3x + 1) – 12 (x2 + 2x + 1) + 2(x + 1) – 1
= x4 + 6x3 – 12x – 8
∴ Tranformed equation is x4 + 6x3 – 12x – 8 = 0
∴ Required equation is x4 – 6x3 + 4x – 53 = 0
or x4 + 6x3 – 12x – 8 = 0.
ii) Given equation is x3 + 2x2 + x + 1 = 0
Let f(x) = x3 + 2x2 + x + 1
We have o find ‘h’ so that the coefficient of the third highest power of ‘x’ in f(x + h) is zero.
i.e., Coefficient of x in f(x + h) is zero.
f(x + h) = (x + h)3 + 2(x + h)2 + (x + h) + 1
Coefficient of ‘x3’ in f(x + h) is 3h2 + 4h + 1
We have to Choose ‘h’ such that 3h2 + 4h + 1 = 0
i.e., h = – 1 or h = – \(\frac{1}{3}\).
Case – (I):
When h = – 1
f(x – 1) = (x – 1)3 + 2(x – 1)2 +(x – 1) + 1
= (x3 – 3x2 + 3x – 1)2 + 2 (x2 – 2x + 1) + x – 1 + 1
= x3 – x2 + 1
∴ Transformed euluation is x3 – x2 + 1 = 0.
Case – (ii):
When h = – \(\frac{1}{3}\)
\(f\left(x-\frac{1}{3}\right)=\left(x-\frac{1}{3}\right)^3+2\left(x-\frac{1}{3}\right)^2+\left(x-\frac{1}{3}\right)\)
= \(\left(x^3-x^2+\frac{x}{3}-\frac{1}{27}\right)+2\left(x^2-\frac{2}{3} x+\frac{1}{9}\right)\) + x – \(\frac{1}{3}\) + 1
= x3 + x2 + \(\frac{23}{27}\)
∴ Transformed equation is x3 + x2 + \(\frac{23}{27}\)
⇒ 27x3 + 27x2 + 23 = 0
∴ Required equation is x3 – x2 + 1 = 0 or 27x3 + 27x2 + 23 = 0.
![]()
Question 7.
Solve the following equations.
i) x4 – 10x3 + 26x2 – 10x + 1 = 0.
ii) 2x5 + x4 – 12x3 – 12x2 + x + 2 = 0
Solution:
i) Given equatIon is
x4 – 10x3 + 26x2 – 10x + 1 = 0 (1)
This is even degree reciprocal equation of class one.
On dividing (1) by x2,
x2 – 10x + 26 – \(\frac{10}{x}+\frac{1}{x^2}\) = 0
⇒ \(\left(x^2+\frac{1}{x^2}\right)-10\left(x+\frac{1}{x}\right)\) + 26 = 0
⇒ \(\left(x^2+\frac{1}{x^2}\right)-10\left(x+\frac{1}{x}\right)\) + 24 = 0
[Put x + \(\frac{1}{x}\) = y)
⇒ y2 – 10y + 24 = 0
⇒ (y – 4) (y – 6) = 0
⇒ y = 4 or y = 6.
Case – (i):
When y = 4
⇒ x + \(\frac{1}{x}\) = 4
⇒ x2 – 4x + 1 = 0
⇒ x = 2 ± √3.
Case – (ii):
When y = 6
⇒ x + \(\frac{1}{x}\) = 4
⇒ x2 – 6x + 1 = 0
⇒ x = 3 ± 2√2
∴ The roots are 2 ± √3, 3 ± 2√2.
![]()
ii) Given equation is
2x5 + x4 – 12x3 – 12x2 + x + 2 = 0 ………………. (1)
Let f(x) = 2x5 + x4 – 12x3 – 12x2 + x + 2
(1) is an odd degree reciprocal equation of class one.
∴ x = – 1 is a root of (1)
x + 1 is a factor of f(x).
We divide f(x) with x + 1.
By synthetic division,
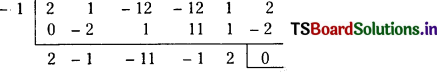
∴ f(x) = (x + 1) (2x4 – x3 – 11x2 – x + 2)
Let g(x) = 2x4 – x3 – 11x2 – x + 2
g(x) = 0 is an even degree reciprocal equation 0f class one.
Dividing g(x) = 0 by x2
We get 2x2 – x – 11 – \(\frac{1}{x}+\frac{2}{x^2}\) = 0
⇒ \(2\left(x^2+\frac{1}{x^2}\right)-\left(x+\frac{1}{x}\right)\) – 11 = 0
⇒ \(2\left(x+\frac{1}{x}\right)^2-\left(x+\frac{1}{x}\right)\) – 15 = 0
(Put x + \(\frac{1}{x}\) = y)
⇒ 2y2 – y – 15 = 0
⇒ (2y + 5) (y – 3) = 0
∴ y = 3 or y = – 1.
Case – (i) :
When y = 3
x + \(\frac{1}{x}\) = 3
⇒ x2 – 3x + 1 = 0
⇒ x = \(\frac{3 \pm \sqrt{5}}{2}\)
Case – (ii):
When y = \(\frac{-5}{2}\)
x + \(\frac{1}{x}\) = \(\frac{-5}{2}\)
⇒ 2x2 + 2 = – 5x
⇒ 2x2 + 5x + 2 = 0
⇒ (2x + 1) (x + 2) = 0
∴ x = – \(\frac{-1}{2}\) or x = – 2
∴ The roots are – 1, 2, \(\frac{3 \pm \sqrt{5}}{2}\).