Students can practice 10th Class Maths Textbook SSC Solutions Telangana Chapter 14 Statistics Ex 14.4 to get the best methods of solving problems.
TS 10th Class Maths Solutions Chapter 14 Statistics Exercise 14.4
Question 1.
The following distribution gives the daily income of 50 workers of a factory.
| Daily income (in Rupees) | 250-300 | 300-350 | 350-400 | 400-450 | 450-500 |
| Number of workers | 12 | 14 | 8 | 6 | 10 |
Convert the distribution above to a less than type cumulative frequency distribution and draw its ogive. (AS5)
Solution:
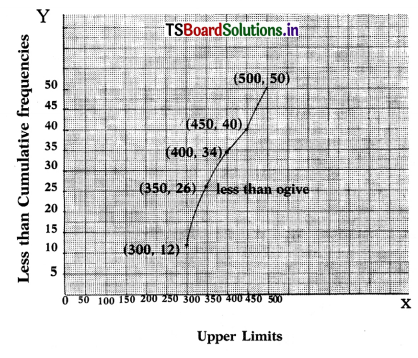
For less than ogive, we take the upper class limits on X-axis and their corresponding cumulative frequencies on Y – axis, choosing a convenient scale.
| Daily income (in Rupees) | 250-300 | 300-350 | 350-400 | 400-450 | 450-500 |
| Number of workers | 12 | 14 | 8 | 6 | 10 |
| Class Interval (Upper Limits) | frequency | cumulative frequency | Points |
| 300 | 12 | 12 | (300, 12) |
| 350 | 14 | 26 | (350, 26) |
| 400 | 8 | 34 | (400, 34) |
| 450 | 6 | 40 | (450, 40) |
| 500 | 10 | 50 | (500, 50) |
The points to be plotted are (300, 12) (350, 26) (400, 34) (450, 40) and (500, 50)
Scale :
On X-axis : 1 cm = 50 units
On Y-axis : 1 cm = 5 units
Question 2.
During the medical check-up of 35 students of a class, their weights were recorded as follows : (AS5)
| Weight (in kg) | Number of students |
| Less than 38 | 0 |
| Less than 40 | 3 |
| Less than 42 | 5 |
| Less than 44 | 9 |
| Less than 46 | 14 |
| Less than 48 | 28 |
| Less than 50 | 32 |
| Less than 52 | 35 |
Draw a less than type ogive for the given data. Hence obtain the median weight from the graph and verify the result by using the formula.
Solution:
The points to be plotted on a graph paper are : (38, 0), (40, 3), (42, 5), (44, 9), (46, 14), (48, 28), (50, 32), (52, 35)
Scale :
On X-axis : 1 cm = 2 units
On Y-axis : 1 cm = 2 units
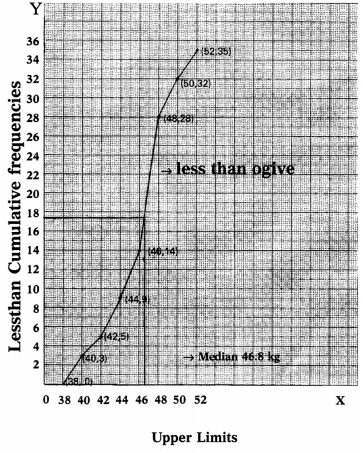
Number of observations = 35
Here \(\frac{\mathrm{n}}{2}\) = \(\frac{35}{2}\) = 17.5
Locate the point 17.5 on the Y – axis. From the point, draw a line parallel to the X – axis cutting the curve at a point. From the point, draw a perpendicular to the X – axis.
![]()
The point of intersection of this perpendicular with the X – axis determines the median of the given data as 46.8 kg.
| Weight | Number of Students (c.f) | Frequency (f) |
| Below 38 | 0 | 0 |
| 38-40 | 3 | 3 |
| 40-42 | 5 | 2 |
| 42-44 | 9 | 4 |
| 44-46 | 14 | 5 |
| 46-48 | 28 | 14 |
| 18-50 | 32 | 4 |
| 50-52 | 35 | 3 |
Number of observations = n = 35
\(\frac{\mathrm{n}}{2}\) = \(\frac{35}{2}\) = 17.5
17.5 belongs to the class 46 – 48
∴ Median class = 46 – 48
l – lower boundary of class = 46
f – frequency of the median class = 14
c.f. = 14
Class size = 2
Median = l + \(\frac{\left(\frac{n}{2}-c . f .\right)}{f}\) × h
= 46 + \(\frac{17.5-14}{14}\) × 2
= 46 + \(\frac{3.5}{14}\) × 2
= 46 + \(\frac{7}{14}\) = 46 + \(\frac{1}{2}\) = 46.5
Hence, median is 46.5 either ways.
![]()
Question 3.
The following table gives production yield per hectare of wheat of 100 farms of a village. (AS5)
| Production yield (Qui/Hec) | 50-55 | 55-60 | 60-65 | 65-70 | 70-75 | 75-80 |
| Number of farmers | 2 | 8 | 12 | 24 | 38 | 16 |
Change the distribution to a more than type distribution and draw its ogive.
Solution:
More than type distribution
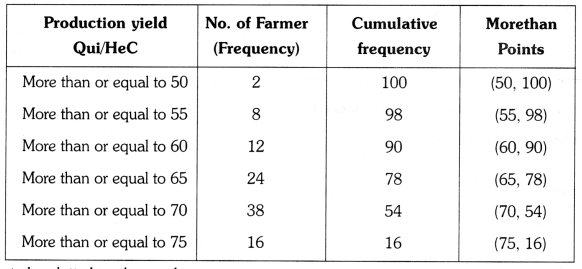
Points to be plotted on the graph paper are:
(50, 100), (55, 98), (60, 90), (65. 78), (70, 54) and (75, 16)
Scale:
On X-axis: 1 cm = 5 units
On Y-axis: 1 cm = 10 units
