TS 10th Class Maths Solutions Chapter 14 Statistics Ex 14.1

Students can practice 10th Class Maths Textbook SSC Solutions Telangana Chapter 14 Statistics Ex 14.1 to get the best methods of solving problems.

TS 10th Class Maths Solutions Chapter 14 Statistics Exercise 14.1

Question 1.
A survey was conducted by a group of students as a part of their environment awareness programme, in which they collected the following data regarding the number of plants in 20 houses in a locality. Find the mean number of plants per house.

Number of plants	0 – 2	2 – 4	4-6	6 – 8	8 – 10	10 – 12	12-14
Number of houses	1	2	1	5	6	2	3

Solution:

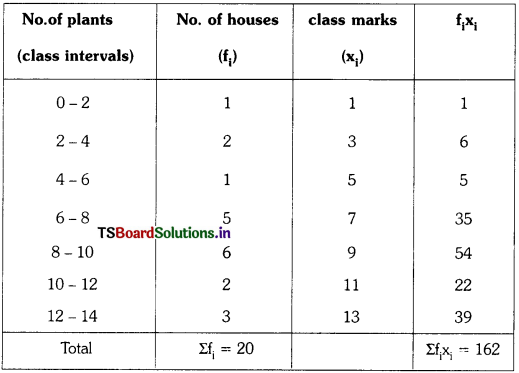
∴ \(\overline{\mathrm{x}}\) = \(\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{x}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\) = \(\frac{162}{20}\) = 8.1
!! Since f_i and x_i are of small values we use direct method.

Question 2.
Consider the following distribution of daily wages of 50 workers of a factory. (AS₄)

Daily wages in Rupees	200 – 250	250 – 300	300 – 350	350 – 400	400- 450
Number of workers	12	14	8	6	10

Find the mean daily wages of the workers of the factory by using an appropriate method.
Solution:

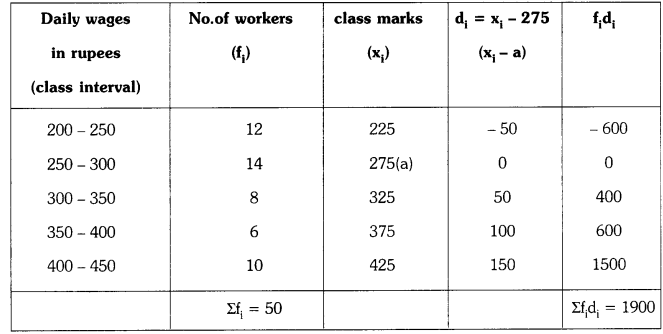
Here, the x_i are of large numerical values in 250 – 300. So, a = 275.
So we use Assumed Mean method then,
\(\overline{\mathrm{x}}\) = a + \(\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{d}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\)
Here, the Assumed mean is taken as 275.
∴ \(\overline{\mathrm{x}}\) = a + \(\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{d}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\) = 275 + \(\frac{1900}{50}\) = 275 + 38 = 313

Question 3.
The following distribution shows the daily pocket allowance of children of a locality. The mean pocket allowance is ₹ 18. Find the missing frequency f. (AS₄)

Daily pocket allowance(in Rupees)	11 – 13	13 – 15	15 – 17	17 – 19	19 – 21	21 – 23	23 – 25
Number of children	7	6	9	13	f	5	4

Solution:

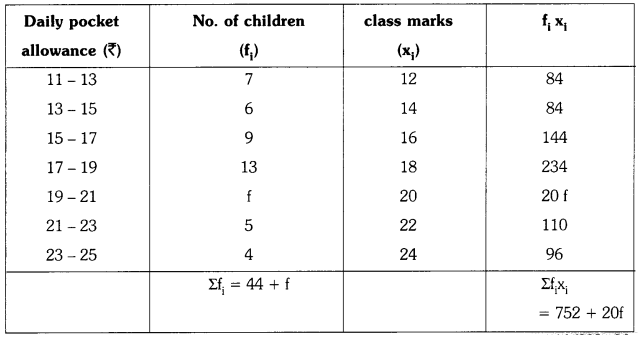
\(\overline{\mathrm{x}}\) = \(\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{d}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\)
\(\overline{\mathrm{x}}\) = 18 (given)
⇒ 18 = \(\frac{752+20 f}{(44+f)}\)
⇒ 18(44 + f) = 752 + 20f ⇒ 20f – 18f = 792 – 752
⇒ 2f = 40
∴ f = \(\frac{40}{2}\) = 20

Question 4.
Thirty women were examined in a hospital by a doctor and their of heart beats per minute were recorded and summarised as shown. Find the mean heart beats per minute for these women, choosing a suitable method. (AS₄)

Number of heart beats/minute	65-68	68-71	71-74	74-77	77-80	80-83	83-86
Number of women	2	4	3	8	7	4	2

Solution:

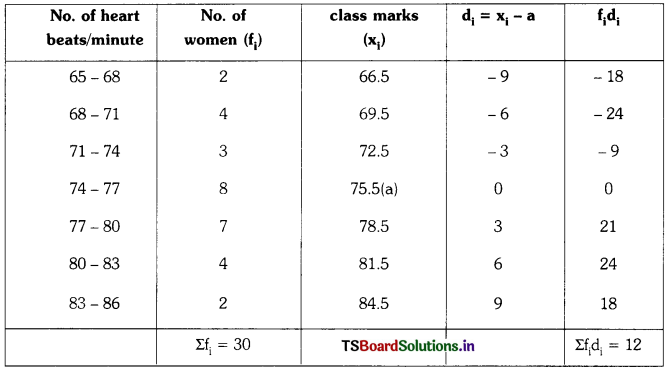
\(\overline{\mathrm{x}}\) = a + \(\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{d}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\)
= 75.5 + \(\frac{12}{30}\) = 75.5 + 0.4 = 75.9

Question 5.
In a retail market, fruit vendors were selling oranges kept in packing baskets. These baskets contained varying number of oranges. The following was the distribution of oranges. (AS₄)

Number of oranges	10-14	15-19	20-24	25-29	30-34
Number of baskets	15	110	135	115	25

Find the mean number of oranges kept in each basket. Which method of finding the mean did you choose ?
Solution:

Here, we use step division method where a = 22, h = 5
\(\overline{\mathrm{x}}\) = a + \(\left[\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{u}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\right]\) × h
⇒ 22 + \(\frac{25}{400}\) × 5
⇒ 22 + 0.31 = 22.31

Question 6.
The table below shows the daily expenditure on food of 25 households in a locality.

Daily expenditure (in Rupees)	100-150	150-200	200-250	250-300	300-350
Number of house holds	4	5	12	2	2

Find the mean daily expenditure on food by a suitable method. (AS₄)
Solution:

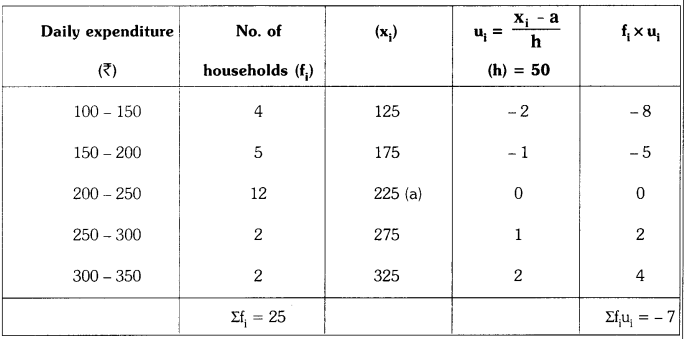
Here, a = 225, h = 50
\(\overline{\mathrm{x}}\) = a + \(\left[\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{u}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\right]\) × h
= 225 + \(\frac{(-7)}{25}\) × 50 = 225 – 14 = 211
The average daily expenditure on food = ₹ 211

Question 7.
To find out the concentration of SO₂ in the air (in parts per million, i.e., ppm), the data was collect for 30 localities in a certain city and is presented below.

Concentration of SO2 in ppm	0.00-0.04	0.04-0.08	0.08-0.12	0.12-0.16	0.16-0.20	0.20-0.24
Frequency	4	9	9	2	4	2

Find the mean concentration of SO₂ in the air. (AS₄)
Solution:

∴ \(\overline{\mathrm{x}}\) = \(\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{d}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\)
= \(\frac{2.96}{30}\) = 0.0986666 …….
≅ 0.099 ppm

Question 8.
A class teacher has the following attendence record of 40 students of a class for the whole term. Find the mean number of days a student was present out of 56 days in the term. (AS₄)

Number of days	35-38	38-41	41-44	44-47	47-50	50-53	53-56
Number of students	1	3	4	4	7	10	11

Solution:

Here, a = 51.5
∴ \(\overline{\mathrm{x}}\) = a + \(\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{d}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\)
= 51.5 – \(\frac{99}{40}\)
⇒ 51.5 – 2.475 = 49.025 ≅ 49 days

Question 9.
The following table gives the literacy rate (in percentage) of 35 cities. Find the mean literacy rate. (AS₄)

Literacy rate in %	45-55	55-65	65-75	75-85	85-95
Number of cities	3	10	11	8	3

Solution:

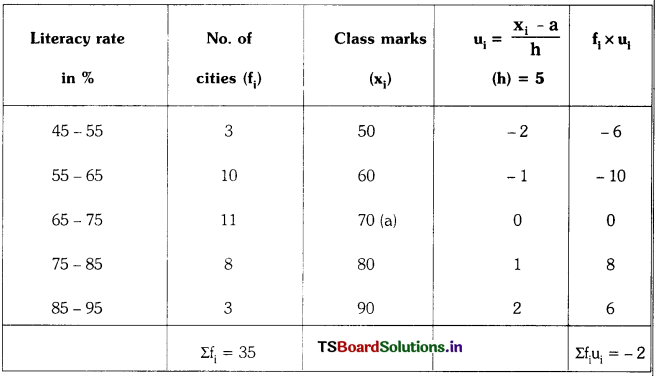
Here a = 70, h = 10
∴ \(\overline{\mathrm{x}}\) = a + \(\frac{\Sigma \mathrm{f}_{\mathrm{i}} \mathrm{d}_{\mathrm{i}}}{\Sigma \mathrm{f}_{\mathrm{i}}}\) × h
⇒ \(\overline{\mathrm{x}}\) = 70 – \(\frac{2}{35}\) × 10
= 70 – \(\frac{20}{35}\) = 70 – 0.5714
= 69.4285 ≅ 69.43%