TS 10th Class Maths Solutions Chapter 10 Mensuration Ex 10.3

Students can practice 10th Class Maths Study Material Telangana Chapter 10 Mensuration Ex 10.3 to get the best methods of solving problems.

TS 10th Class Maths Solutions Chapter 10 Mensuration Exercise 10.3

Question 1.
An iron pillar consists of a cylindrical portion of 2.8 m height and 20 cm in di-ameter and a cone of 42 cm height sur-mounting it. Find the weight of the pillar if 1cm3 of iron weighs 7.5g. (AS4)
Solution:
Volume of the iron pillar = Volume of the cylinder + volume of the cone
Cylinder : Radius = \(\frac{\text { diameter }}{2}\)
= \(\frac{20}{2}\) = 10 cm
Height = 2.8 m
= 280 cm
Volume = πr²h
= \(\frac{22}{7}\) × 10 × 10 × 280
= 88,000 cm³

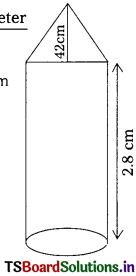
Cone : Radius ‘r’ = \(\frac{\text { diameter }}{2}\)
= \(\frac{20}{2}\) = 10 cm
Height ‘h’ = 42 cm
Volume = \(\frac{1}{3}\)πr²h
= \(\frac{1}{3}\) × \(\frac{22}{7}\) × 10 × 10 × 42
= 4,400 cm³.
Total volume = 88,000 + 4,400
= 92,400 cm³
∴ Total weight of the pillar at a weight of 7.5g per 1 cm³ = 92,400 × 7.5
= 6,93,000 gms
= \(\frac{6,93,000}{1,000}\) kg = 693 kg

Question 2.
A toy is made in the form of hemisphere surmounted by a right cone whose cicular, base is joined with the plane surface of hemisphere. The radius of the base of the cone is 7cm and its volume is \(\frac{3}{2}\) of hemisphere. Calculate the height of the cone and surface area of the toy correct to 2 places of decimal (take π = 3\(\frac{1}{7}\)) (AS4)
Solution:
Radius of the base of the cone(r) = 7 cm
Radius of hemisphere = 7cm
Volume of hemisphere = \(\frac{2}{3}\) πr³
= \(\frac{2}{3}\) × \(\frac{22}{7}\) × 7 × 7 × 7cm³
Volume of the cone
= \(\frac{3}{2}\) × volume of the hemisphere (given)
= \(\frac{3}{2}\) × \(\frac{2}{3}\) × \(\frac{22}{7}\) × 7 × 7 × 7
= 1078

Volume of cone = \(\frac{1}{3}\) πr²h = 1,078
= \(\frac{1}{3}\) × \(\frac{22}{7}\) × 7 × 7 × h = 1078
∴ h = 1,078 × \(\frac{3}{1}\) × \(\frac{7}{22}\) × \(\frac{1}{7}\) × \(\frac{1}{7}\) = 21cm
l² = h² + r² = 21² + 7²
= 441 + 49 = 490
l = \(\sqrt{490}\) = \(\sqrt{49 \times 10}\)
= 7\(\sqrt{10}\) = 7 × 3.16 = 22.12 cm
Surface area of the toy = curved surface area of the cone + curved surface area of the hemisphere = πrl + 2 πr²
= \(\frac{22}{7}\) × 7 × 7 × \(\sqrt{10}\) + 2 × \(\frac{22}{7}\) × 7 × 7
= \(\frac{22}{7}\) × 7 [7\(\sqrt{10}\) + 14]
= 22 × (22.12 + 14)
= 22 × 36.12 = 794.64 cm²

Question 3.
Find the volume of the largest right circular cone that can be cut out of a cube whose edge is 7cm. (AS4)
Solution:
The base of the largest right circular cone will be the circle inscribed in a face of the cube and its height will be equal to an edge of the cube.
∴ r = Radius of the base of the cone
= \(\frac{7}{2}\) cm (∴ edge = 7cm)
h = height of the cone = 7cm
Hence, volume of the cone
= \(\frac{1}{3}\) πr²h
= \(\frac{1}{3}\) × \(\frac{22}{7}\) × \(\frac{7}{2}\) × \(\frac{7}{2}\) × 7
= \(\frac{539}{6}\) cm³ = 89.83 cm³

Question 4.
A cylindrical tub of radius 5 cm and length 9.8 cm is full of water. A solid in the form of right circular cone mounted on a hemisphere is immersed into the tub. The radius of the hemisphere is 3.5 cm and height of cone outside the hemisphere is 5 cm. Find the volume of water left in tub (Take π = \(\frac{22}{7}\)) (AS4)
Solution:
Radius of the cylinder(r) = 5 cm
Height of the cylinder (h) = 9.8 cm
Volume of the cylinder = πr²h

= \(\frac{22}{7}\) × 5 × 5 × 9.8
= 770 cm³.
Radius of hemi-sphere(r) = 3.5 cm
Volume of the hemi-sphere = \(\frac{2}{3}\) πr³
= \(\frac{2}{3}\) × \(\frac{22}{7}\) × 35 × 35 × 35
= \(\frac{539}{6}\) cm³

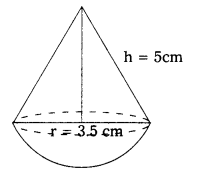
Radius of the base of the cone(r) = 3.5 cm
Height of the cone = 5 cm
∴ Volume of the cone = \(\frac{1}{3}\)πr²h
= \(\frac{1}{3}\) × \(\frac{22}{7}\) × 3.5 × 3.5 × 5
= \(\frac{385}{6}\) cm³
Total volume of the solid = volume of the hemisphere + volume of the cone
= \(\frac{539}{6}\) + \(\frac{385}{6}\) = \(\frac{924}{6}\) = 154 cm²
The volume of water left in the tub = volume of the cylindrical tube – volume of the solid immersed
= 770 – 154 = 616 cm³

Question 5.
In the adjacent figure, the height of a solid cylinder is 10 cm and diameter is 7 cm. Two equal conical holes of radius 3 cm and height 4 cm are cut off as shown in figure. Find the volume of the remaining solid. (AS4)

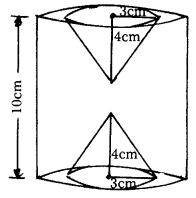
Solution:
Radius of the solid cylinder (r) = \(\frac{7}{2}\) cm
(∴ diameter = 7cm)
Height of the solid cylinder(h) = 10 cm
Volume of the solid cylinder = πr²h
= \(\frac{22}{7}\) × \(\frac{7}{2}\) × \(\frac{7}{2}\) × 10 = 385 cm³
Radius of concial hole(r) = 3 cm
Height(depth) of the conical hole(h) = 4 cm
Volume of the conical part = \(\frac{1}{3}\) pr²h
= \(\frac{1}{3}\) × \(\frac{22}{7}\) × 3 × 3 × 4
Total volume of two conical holes
= 2 × \(\frac{1}{3}\) × \(\frac{22}{7}\) × 3 × 3 × 4
= \(\frac{528}{7}\) = 75.43 cm³
Hence, volume of the remaining solid
= [Volume of the Cylindrical part] – [Total volume of two conical holes]
= 385 – 75.43
= 309.57cm³

Question 6.
Spherical Marbles of diameter 1.4 cm are dropped into a cylindrical beaker of diameter 7cm, which contains some water. Find the number of marbles that should be dropped into the beaker, so that water level rises by 5.6 cm. (AS4) Solution:
Rise in the water level is seem in cylinderial shape of Radius = Beaker radius

= \(\frac{\text { diameter }}{2}\) = \(\frac{7}{2}\) = 3.5 cm
Height ‘h’ of the rise = 5.6 cm
∴ Volume of the ‘water rise’ = πr²h
= \(\frac{22}{7}\) × 3.5 × 3.5 × 5.6
= \(\frac{22 \times 12.25 \times 5.6}{7}\) = 215.6
Volume of each marble dropped
= \(\frac{4 \pi r^3}{3}\)
Where radius r = \(\frac{\mathrm{d}}{2}\) = \(\frac{1.4}{2}\) = 0.7 cm
∴ V = \(\frac{4}{3}\) × \(\frac{22}{7}\) × 0.7 × 0.7 × 0.7
≈ 1.4373 cm³
∴ Volume of the ‘rise’ = Total volume of the marbles.
Let the number of marbles be ‘n’ then
n × volume of each marble = Volume of the rise
n × 1.4373 = 215.6
n = \(\frac{215.6}{1.4373}\) = 150
∴ Number of marbles = 150

Question 7.
A pen stand is made of wood in the shape of cuboid with three conical depression to hold the pens. The dimensions of the cuboid are 15 cm by 10cm by 3.5cm. The radius of each of the depression is 0.5 cm and the depth is 1.4 cm. Find the volume of wood in the entire stand. (AS4)

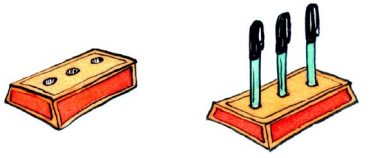
Solution:
The dimensions of the cuboid are
15 cm × 10 cm × 3.5 cm
∴ Volume of the ‘cuboid’ = \(\frac{15 \times 10 \times 7}{2}\)
= 525 cm³
Radius of the conical depression (r) = 0.5 cm
Depth of conical hole (h) = 1.4 cm
= \(\frac{14}{10}\) cm
Volume of one conical hole
= \(\frac{1}{3}\) × \(\frac{22}{7}\) × \(\frac{1}{2}\) × \(\frac{1}{2}\) ×\(\frac{14}{10}\)
Volume of three conical holes
= 3 × \(\frac{1}{3}\) × \(\frac{22}{7}\) × \(\frac{1}{2}\) × \(\frac{1}{2}\) × \(\frac{14}{10}\)
= \(\frac{11}{10}\) = 1.1 m³
∴ The volume of wood in the entire stand
= (Volume of the cuboid) – (volume of 3 conical holes)
= 525 – 1.1 = 523.9cm³.