TS Inter 2nd Year Maths 2B Solutions Chapter 7 Definite Integrals Ex 7(b)

Students must practice this TS Intermediate Maths 2B Solutions Chapter 7 Definite Integrals Ex 7(b) to find a better approach to solving the problems.

TS Inter 2nd Year Maths 2B Solutions Chapter 7 Definite Integrals Exercise 7(b)

I. Evaluate the following definite integrals.

Question 1.
\(\int_0^a\left(a^2 x-x^3\right) d x\)
Solution:

Question 2.
\(\int_2^3 \frac{2 x}{1+x^2} d x\) (Mar. ’12)
Solution:
Let 1 + x² = t, then 2x dx = dt
Upper limit t = 1 + 9 = 10 when x = 3
Lower limit t = 1 + 4 = 5 when x = 2

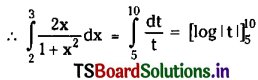
= log 10 – log 5
= log 2

Question 3.
\(\int_0^\pi \sqrt{2+2 \cos \theta} d \theta\)
Solution:

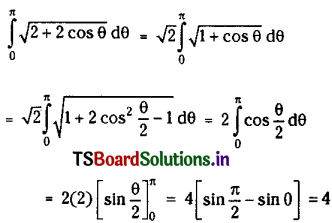
Question 4.
\(\int_0^\pi \sin ^3 x \cos ^3 x d x\)
Solution:
I = \(\int_0^\pi \sin ^3 x \cos ^3 x d x\)

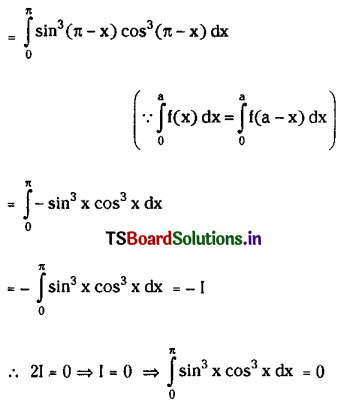
Question 5.
\(\int_0^2|1-x| d x\)
Solution:

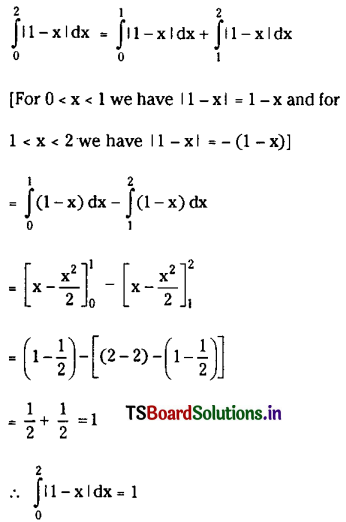
Question 6.
\(\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \frac{\cos x}{1+e^x} d x\)
Solution:

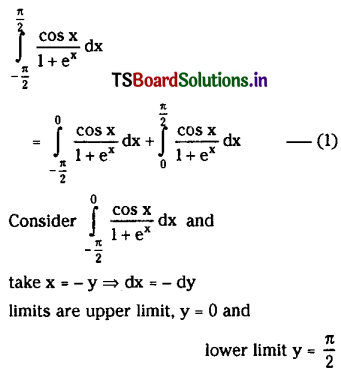
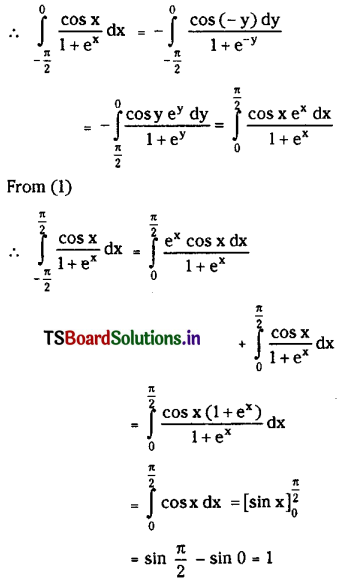
Question 7.
\(\int_0^1 \frac{d x}{\sqrt{3-2 x}}\)
Solution:
Let 3 – 2x = t² then -2 dx = 2t dt
∴ Upper limit t² = 1 ⇒ t = 1
and Lower limit t² = 3 ⇒ t = √3

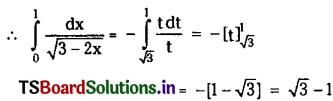
Question 8.
\(\int_0^a(\sqrt{a}-\sqrt{x})^2 d x\)
Solution:

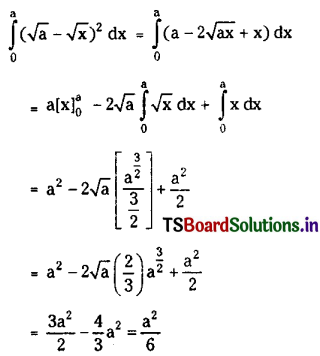
Question 9.
\(\int_0^{\frac{\pi}{4}} \sec ^4 \theta d \theta\)
Solution:

Question 10.
\(\int_0^3 \frac{x}{\sqrt{x^2+16}} d x\)
Solution:
Let x² + 16 = t2
Upper limit: x = 3
t² = 25
⇒ t = 5
⇒ 2x dx = 2t dt
Lower limit; x = 0 ⇒ t = 4
∴ \(\int_4^5 \frac{t d t}{t}=[t]_4^5\) = 1

Question 11.
\(\int_0^1 x e^{-x^2} d x\)
Solution:
Let x² = t then x dx = \(\frac{1}{2}\) dt
Upper limit x = 1 ⇒ t = 1
Lower limit x = 0 ⇒ t = 0

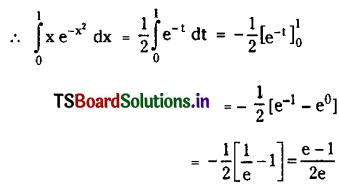
Question 12.
\(\int_1^5 \frac{d x}{\sqrt{2 x-1}}\)
Solution:
Let 2x – 1 = t² then 2 dx = 2t dt
⇒ dx = t dt
Upper limit when x = 5
⇒ t² = 9
⇒ t = 3
Lower limit when x = 1
⇒ t² = 1
⇒ t = 1 (taking positive values in [1, 5])

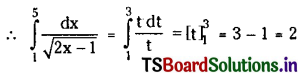
II. Evaluate the following integrals.

Question 1.
\(\int_0^4 \frac{x^2}{1+x} d x\)
Solution:

Question 2.
\(\int_{-1}^2 \frac{x^2}{x^2+2} d x\)
Solution:

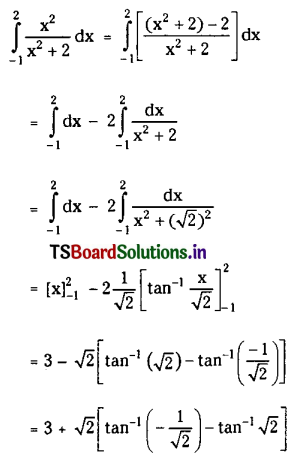
Question 3.
\(\int_0^1 \frac{x^2}{x^2+1} d x\) (New Model Paper, TET, Mar. ’11)
Solution:

Question 4.
\(\int_0^{\frac{\pi}{2}} x^2 \sin x d x\)
Solution:
Applying integration by parts
taking u = x² and v = sin x we get

Question 5.
\(\int_0^4|2-x| d x\) (May ’11)
Solution:
If x > 2 then |2 – x| = -(2 – x) = x – 2
If x < 2 then |2 – x| = 2 – x

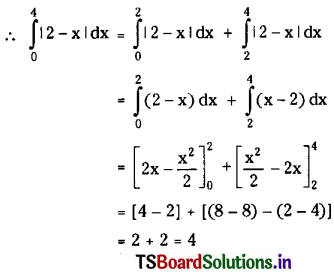
Question 6.
\(\int_0^{\frac{\pi}{2}} \frac{\sin ^5 x}{\sin ^5 x+\cos ^5 x} d x\)
Solution:

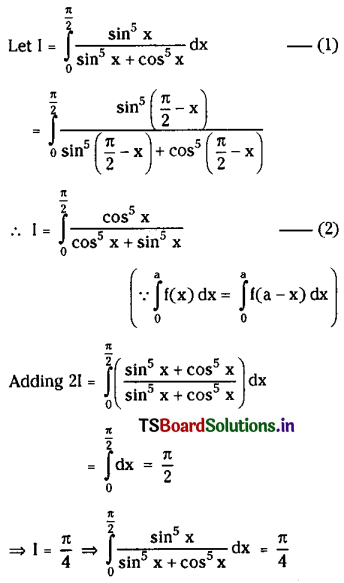
Question 7.
\(\int_0^{\frac{\pi}{2}} \frac{\sin ^2 x-\cos ^2 x}{\sin ^3 x+\cos ^3 x} d x\) (New Model Test Paper & Mar. ’12)
Solution:

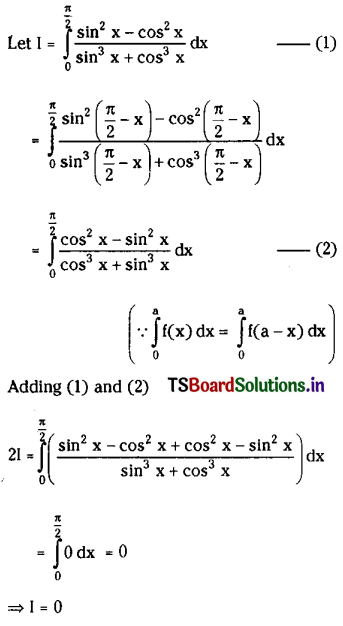
Evaluate the following limits.

Question 8.
\(\lim _{n \rightarrow \infty} \frac{\sqrt{n+1}+\sqrt{n+2}+\ldots+\sqrt{n+n}}{n \sqrt{n}}\)
Solution:
For determining the limit we use the result that if f is continuous on [0, 1] and

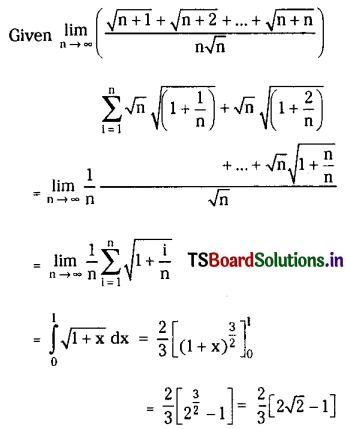
Question 9.
\(\lim _{n \rightarrow \infty}\left[\frac{1}{n+1}+\frac{1}{n+2}+\ldots .+\frac{1}{6 n}\right]\)
Solution:

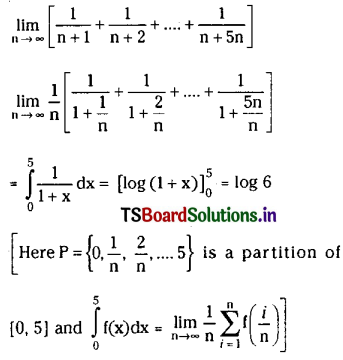
Question 10.
\(\lim _{n \rightarrow \infty} \frac{1}{n}\left[\tan \frac{\pi}{4 n}+\tan \frac{2 \pi}{4 n}+\ldots+\tan \frac{n \pi}{4 n}\right]\)
Solution:

Question 11.
\(\lim _{n \rightarrow \infty} \sum_{i=1}^n \frac{i^3}{i^4+n^4}\)
Solution:

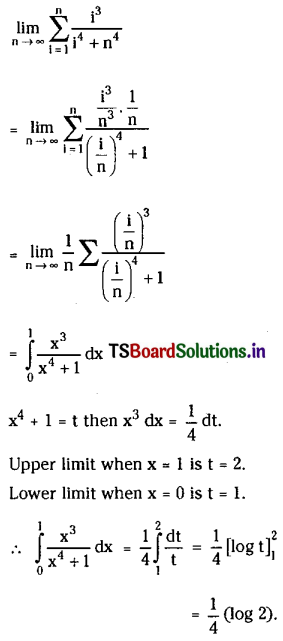
Question 12.
\(\lim _{n \rightarrow \infty} \sum_{i=1}^n \frac{i}{n^2+i^2}\)
Solution:
\(\lim _{n \rightarrow \infty} \sum_{i=1}^n \frac{1}{n^2+i^2}\)
Dividing the numerator and denominator by n² we get

Let 1 + x² = t then x dx = \(\frac{1}{2}\) dt
Upper limit when x = 1 is t = 2
Lower limit when x = 0 is t = 1

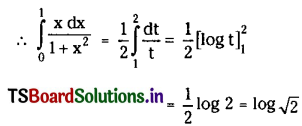
Question 13.
\(\lim _{n \rightarrow \infty}\left(\frac{1+2^4+3^4+\ldots+n^4}{n^5}\right)\)
Solution:

Question 14.
\(\lim _{n \rightarrow \infty}\left[\left(1+\frac{1}{n^2}\right)\left(1+\frac{2^2}{n^2}\right) \cdots \cdot\left(1+\frac{n^2}{n^2}\right)\right]^{\frac{1}{n}}\)
Solution:

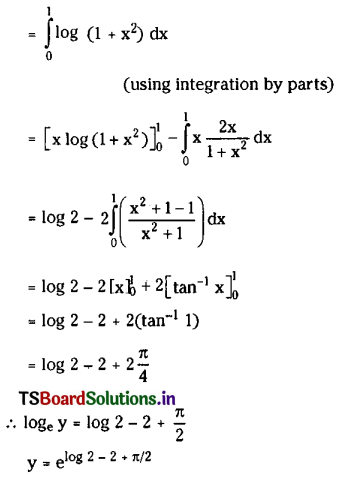
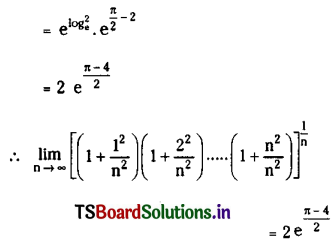
Question 15.
\(\lim _{n \rightarrow \infty}\left[\frac{(n)^{\frac{1}{n}}}{n}\right]\)
Solution:

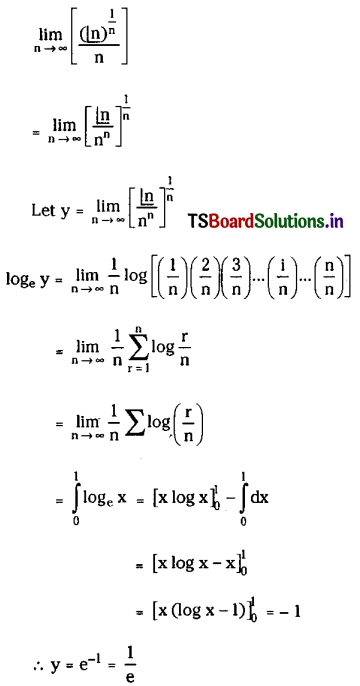
III. Evaluate the following integrals.

Question 1.
\(\int_0^{\frac{\pi}{2}} \frac{d x}{4+5 \cos x}\) (Mar. ’93)
Solution:

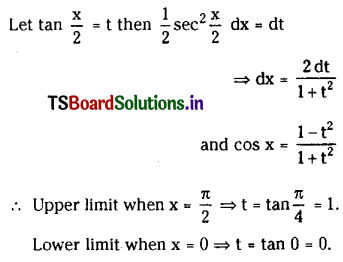
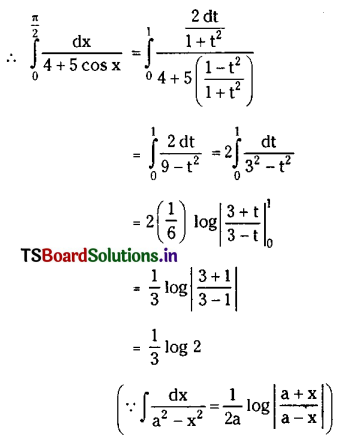
Question 2.
\(\int_a^b \sqrt{(x-a)(b-x)} d x\)
Solution:

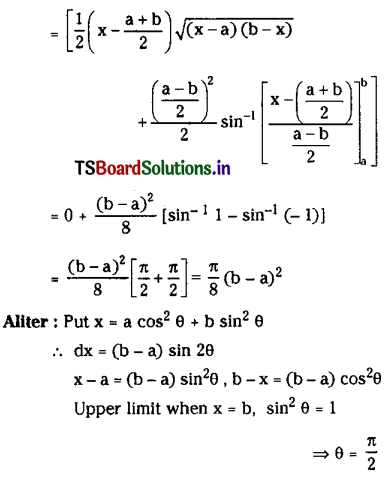
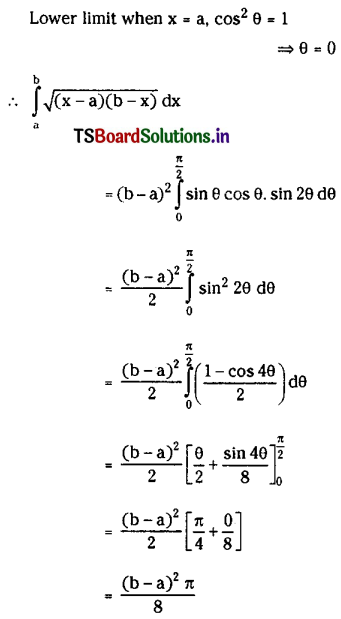
Question 3.
\(\int_0^{\frac{1}{2}} \frac{x \sin ^{-1} x}{\sqrt{1-x^2}} d x\)
Solution:
Let sin^-1x = θ then sin θ = x and dx = cos θ dθ
Upper limit, sin θ = \(\frac{1}{2}\) ⇒ θ = \(\frac{\pi}{6}\)
Lower limit, sin θ = 0 ⇒ θ = 0

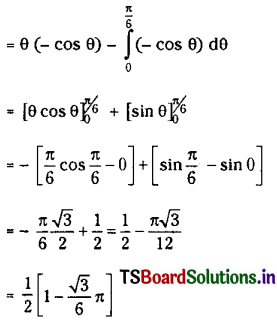
Question 4.
\(\int_0^{\frac{\pi}{4}} \frac{\sin x+\cos x}{9+16 \sin 2 x} d x\) (Apr. ’01)
Solution:

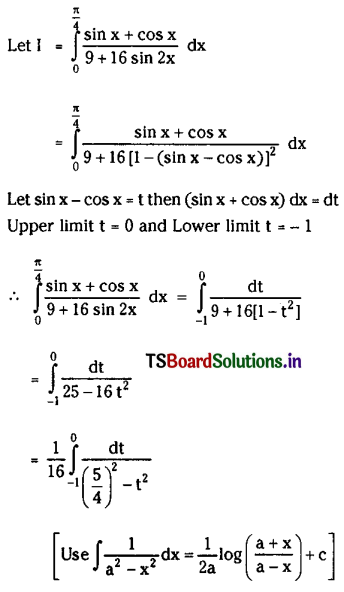
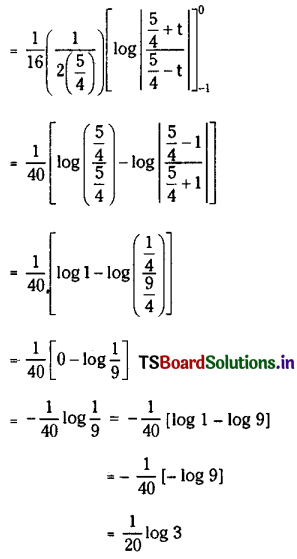
Question 5.
\(\int_0^{\frac{\pi}{2}} \frac{a \sin x+b \cos x}{\sin x+\cos x} d x\)
Solution:

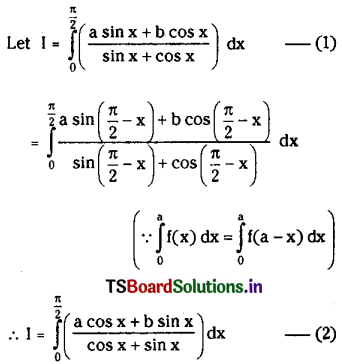
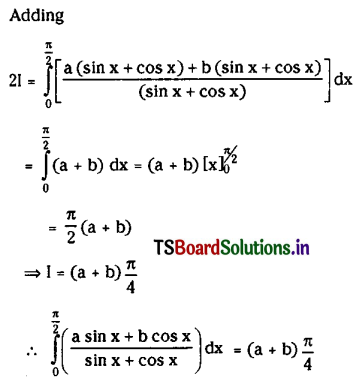
Question 6.
\(\int_0^a x(a-x)^n d x\)
Solution:

Question 7.
\(\int_0^2 x \sqrt{2-x} d x\)
Solution:

Question 8.
\(\int_0^\pi x \sin ^3 x d x\)
Solution:

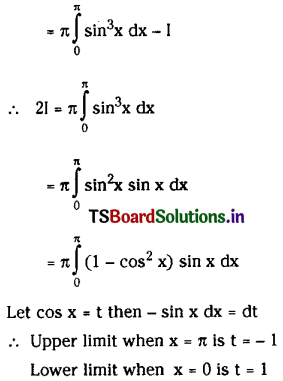
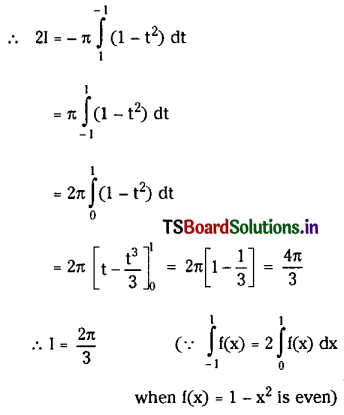
Question 9.
\(\int_0^\pi \frac{x}{1+\sin x} d x\) (May ’11)
Solution:

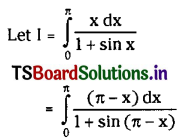
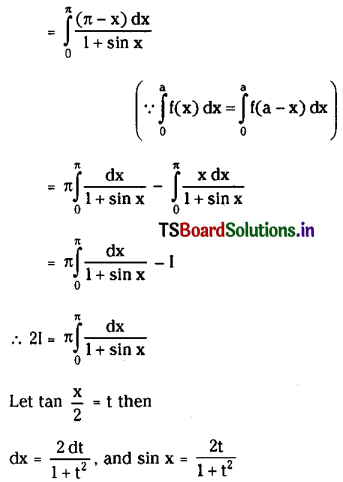
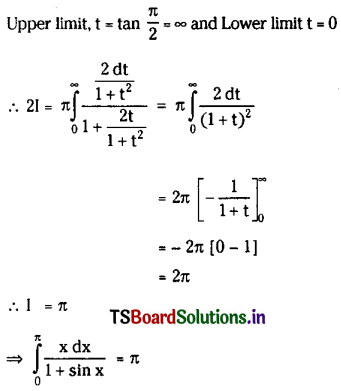
Question 10.
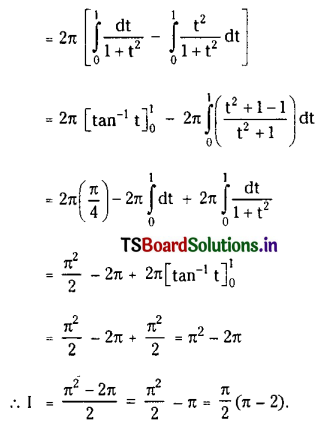
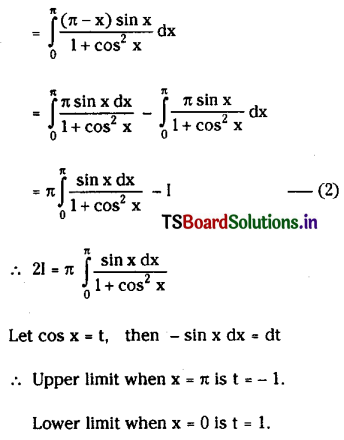
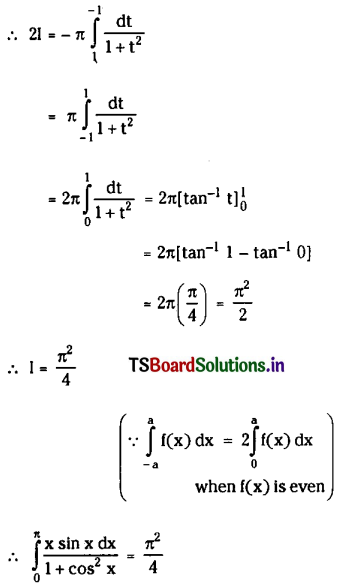
\(\int_0^\pi \frac{x \sin ^3 x}{1+\cos ^2 x} d x\) (Mar. ’11)
Solution:
Let I = \(\int_0^\pi \frac{x \sin ^3 x}{1+\cos ^2 x} d x\)

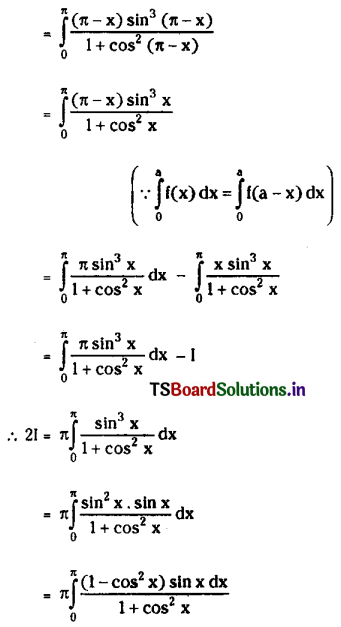
Question 11.
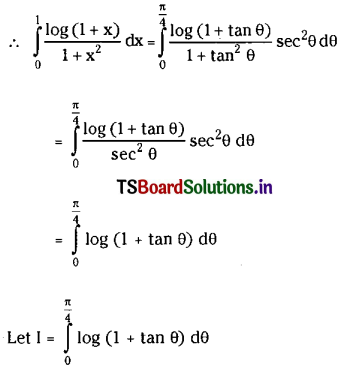
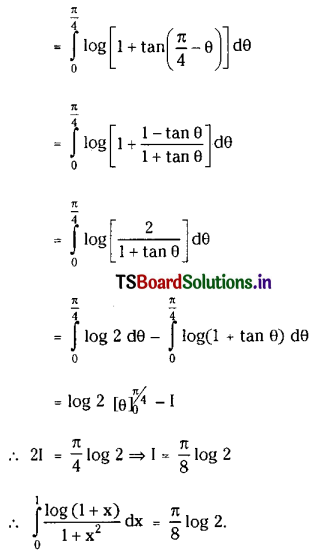
\(\int_0^1 \frac{\log (1+x)}{1+x^2} d x\) (New Model Paper & Mar. ’10)
Solution:
Put x = tan θ then dx = sec²θ dθ
Upper limit when x = 1 is θ = \(\frac{\pi}{4}\)
and Lower limit when x = 0 is θ = 0

Question 12.
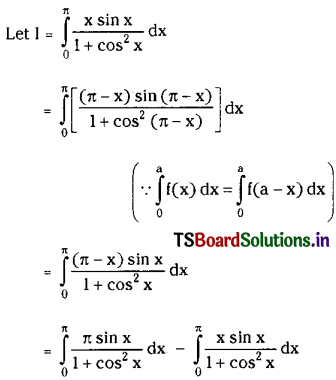
\(\int_0^\pi \frac{x \sin x}{1+\cos ^2 x} d x\) (Apr. ’99)
Solution:

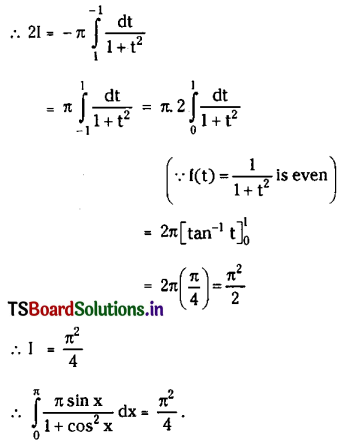
Question 13.
\(\int_0^{\frac{\pi}{2}} \frac{\sin ^2 x}{\cos x+\sin x} d x\)
Solution:

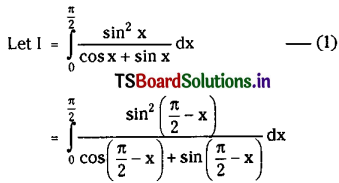
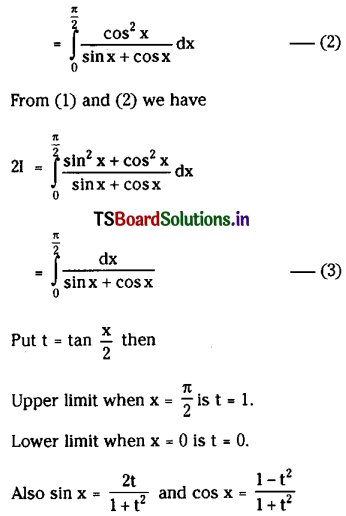
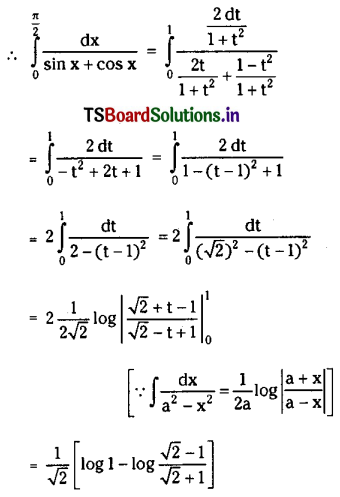
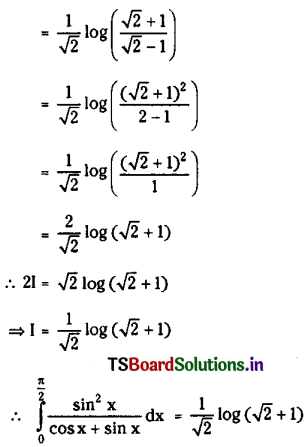
Question 14.
\(\int_0^\pi \frac{1}{3+2 \cos x} d x\)
Solution:

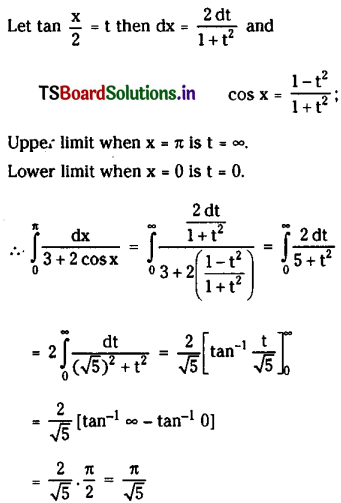
Question 15.
\(\int_0^{\frac{\pi}{4}} \log (1+\tan x) d x\)
Solution:

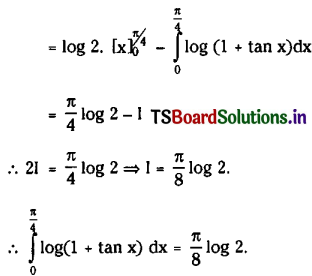
Question 16.
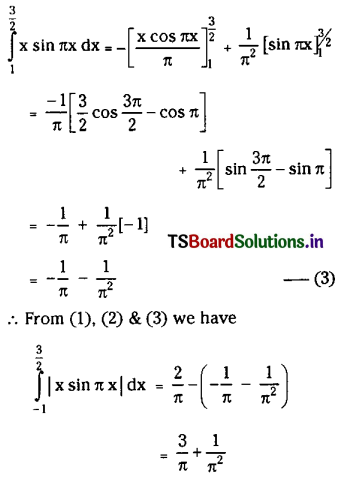
\(\int_{-1}^{\frac{3}{2}}|x \sin \pi x| d x\)
Solution:
We have |x sin πx| = x sin πx when -1 ≤ x ≤ 1
= -x sin πx when 1 < x ≤ \(\frac{3}{2}\)

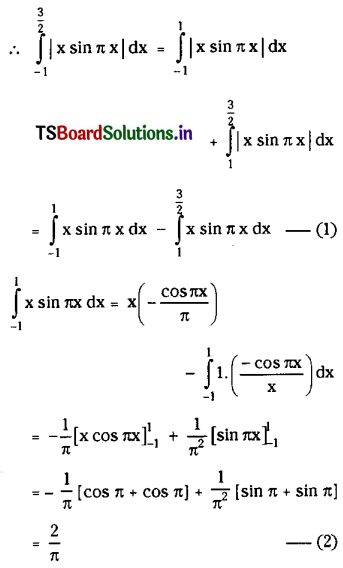
Question 17.
\(\int_0^1 \sin ^{-1}\left(\frac{2 x}{1+x^2}\right) d x\)
Solution:
Let x = tan θ then dx = sec²θ dθ
Upper limit when x = 1 is θ = \(\frac{\pi}{4}\)
and Lower limit when x = 0 is θ = 0

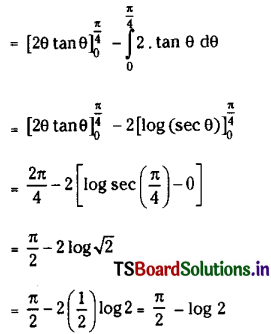
Question 18.
\(\int_0^1 x \tan ^{-1} x d x\)
Solution:
Let x = tan θ then dx = sec²θ dθ
Upper limit when x = 1 is θ = \(\frac{\pi}{4}\)
and Lower limit when x = 0 is θ = 0
∴ \(\int_0^1 x \tan ^{-1} x d x=\int_0^{\frac{\pi}{4}} \theta \tan \theta \sec ^2 \theta d \theta\)
using integration by parts by taking u = θ and v = tan θ sec²θ we get

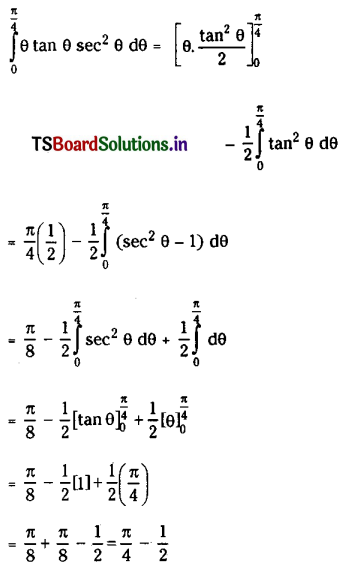
Question 19.
\(\int_0^\pi \frac{x \sin x}{1+\cos ^2 x} d x\)
Solution:

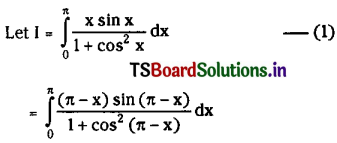
Question 20.
Suppose that f : R → R is a continuous periodic function and T is its period of it. Let a ∈ R. Then prove that for any positive integer n, \(\int_a^{a+n T} f(x) d x=n \int_a^{a+T} f(x) d x\).
Solution:

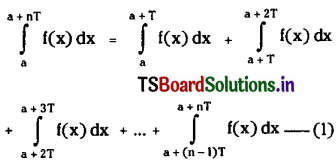
(∵ f is a continuous function with period T)
Consider \(\int_{a+r T}^{a+(r+1) T} f(x) d x\) and (1 < r < r+1 < n)
take x = y + rT and dx = dy
Upper limit when x = a + rt + T is y = a + T
The lower limit when x = a + rT is y = a

(∵ f is periodic ⇒ f(y + rT) = f(y)
Similarly, we can prove that each integral of (1) is equal to \(\int_a^{a+T} f(x) d x\)
Hence \(\int_a^{a+n T} f(x) d x=n \int_a^{a+n T} f(x) d x\)