TS 10th Class Maths Solutions Chapter 10 Mensuration Ex 10.1

Students can practice 10th Class Maths Study Material Telangana Chapter 10 Mensuration Ex 10.1 to get the best methods of solving problems.

TS 10th Class Maths Solutions Chapter 10 Mensuration Exercise 10.1

Question 1.
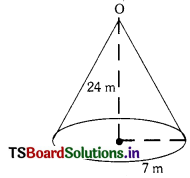
A Joker’s cap is in the form of right circular cone whose base radius is 7cm and height is 24 cm. Find the area of the sheet required to make 10 such caps. (AS4)
Solution:
Radius of the cap (r) = 7 cm
Height of the cap(h) = 24 cm
Slant height of the cap (l) = \(\sqrt{\mathrm{r}^2+\mathrm{h}^2}\)
= \(\sqrt{7^2+24^2}\)
= \(\sqrt{49+576}\)
= \(\sqrt{625}\) = 25
∴ l = 25 cm

Lateral surface area of the cap (cone) = πrl
L.S.A = \(\frac{22}{7}\) × 7 × 25 = 550 cm²
∴ Area of the sheet required for 10 caps
= 10 × 550 = 5500 cm².

Question 2.
A sports Company was ordered to prepare 100 paper cylinders for packing shuttle cocks. The required dimensions of the cylinder are 35 cm length/height and its radius is 7cm. Find the required area of thick paper sheet needed to make 100 cylinders. (AS4)
Solution:
Radius of the cylinder r = 7 cm
Height of the cylinder h = 35 cm
L.S.A of the cylinder with lids at both ends = 2πrh
= 2 × \(\frac{22}{7}\) × 7 × 35 = 1,540 cm²
∴ Area of thick paper required for 100 cylinders
= 100 × 1,540
= 1,54,000 cm²
= \(\frac{1,54,000}{100 \times 100}\) m² = 15.40 m².

Question 3.
Find the volume of right circular cone with radius 6 cm and height 7 cm. (AS1) (Mar. ’16 (A.P.))
Solution:
Radius of the cone (r) = 6 cm
Height of the cone (h) = 7 cm

Volume of the cone = \(\frac{1}{3}\) πr²h
= \(\frac{1}{3}\) × \(\frac{22}{7}\) × 6 × 6 × 7 = 264 cm³.

Question 4.
The lateral surface area of a cylinder is equal to the curved surface area of a cone. If their bases be the same, find the ratio of the height of the cylinder and slant height of the cone. (AS4)
Solution:
Lateral surface area of a cylinder = 2πrh
Curved surface area of the cone = πrl
Given that 2πrh = πrl
⇒ 2h = l
h = l/2
∴ The ratio of the height of the cylinder and slant height of the cone = h : l
⇒ h : 2h [∵ l = 2h]
⇒ 1 : 2.

Question 5.
A self help group wants to manufacture joker’s caps of 3 cm radius and 4 cm height. If the available paper sheet is 1000 cm² then how many caps can be manufactured from that paper sheet ?
Solution:
Radius of the cap (conical cap)(r) = 3 cm
Height of the cap(h) = 4 cm

Slant height l = \(\sqrt{\mathrm{r}^2+\mathrm{h}^2}\)
(by pythagoras theorem)
= \(\sqrt{3^2+4^2}\)
= \(\sqrt{9+16}\)
= \(\sqrt{25}\) = 5 cm
C.S.A of the cap = πrl = \(\frac{22}{7}\) × 3 × 5
= 47.14 cm²
Number of caps that can be made out of
1000 cm² = \(\frac{1000}{47.14}\) ≈ 21.21
∴ Number of caps = 21

Question 6.
A cylinder and cone have bases of equal radii and are of equal heights. Show that their volumes are in the ratio of 3 : 1 (AS4) (June ’15 (A.P.))
Solution:
Given that the bases of a cylinder and a cone are of equal radii.
The height are also equal (Given).
∴ Volume of the cylinder = πr²h
Volume of the cone = \(\frac{1}{3}\) πr²h

∴ The ratio of their volumes
= πr²h : \(\frac{1}{3}\) πr²h
= 1 : \(\frac{1}{3}\)
= 3 : 1
(cancelling the common factor πr²h)

Question 7.
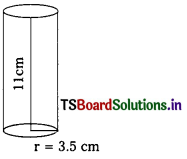
The shape of solid iron rod is a cylindrical. Its height is 11cm. and base diameter is 7 cm. Then find the total volume of 50 such rods. (AS4)
Solution:
Diameter of the cylinder (d) = 7 cm
Radius of the base (r) = \(\frac{7}{2}\) = 3.5 cm
Height of the cylinder (h) = 11 cm
Volume of the cylinder V = πr²h

= \(\frac{22}{7}\) × 3.5 × 3.5 × 11
= 423.5 cm³
∴ The total volume of 50 rods
= 50 × 423.5 cm³
= 21,175 cm³.

Question 8.
A heap of rice is in the form of a cone of diameter 12m and height 8m. Find its volume ? How much canvas cloth is required to cover the heap ? (AS4)
Solution:
Diameter of the heap (conical)

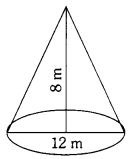
(d) = 12 m
∴ Radius = \(\frac{\mathrm{d}}{2}\) = \(\frac{12}{2}\) = 6 m
Height of the cone (h) = 8 m 1 2
Volume of the cone V = \(\frac{1}{3}\) πr²h
= \(\frac{1}{3}\) × \(\frac{22}{7}\) × 6 × 6 × 8
= 301.71 m³.

Question 9.
The curved surface area of a cone is 4070 cm² and its diameter is 70 cm. What is its slant height ? (AS1) (Mar ’15 (A.P.))
Solution:
Diameter of the base of the cone (d) = 70 cm
∴ Radius of the base(r) = \(\frac{\mathrm{d}}{2}\) = \(\frac{70}{2}\) = 35 cm
Curved surface area of the cone = πrl

By problem = πrl = 4070
= \(\frac{22}{7}\) × 35 × l = 4070
∴ l = 4070 × \(\frac{7}{22}\) × \(\frac{1}{35}\) = 37 cm
Hence, the slant height of the cone = 37cm.