We are offering TS 10th Class Maths Notes Chapter 12 Applications of Trigonometry to learn maths more effectively.
TS 10th Class Maths Notes Chapter 12 Applications of Trigonometry
→ Trigonometry is widely used in our daily life, geography and in navigation also.
→ If a person is looking at an object then the imaginary line joining the object and the eye of the observer is called the line of sight or ray of view.

→ An imaginary line parallel to earth surface and passing through the point of observation is called the horizontal.
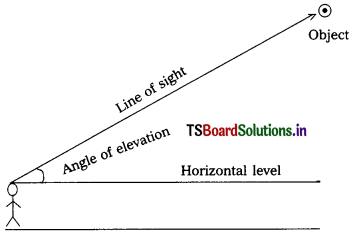
→ If the line of sight is above the horizontal then the angle between them is called “angle of elevation”.

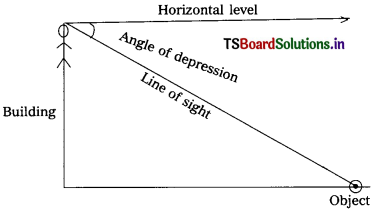
→ If the line of sight is below the horizontal then the angle between them is called the angle of depression.
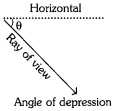
→ Useful hints to solve the problems :
- Draw a neat diagram of a right triangle or a combination of right triangles if necessary.
- Represent the data given on the triangle.
- Find the relation between known values and unknown values.
- Choose appropriate trigonometric ratio and solve for the unknown.
→ The height or length of an object or the distance between two distant objects can be determined with the help of trigonometric ratios.
→ To use this application of trigonometry, we should know the following terms.
→ The terms are Horizontal line, Line of Sight, Angle of Elevation and Angle of Depression.
→ Horizontal line : A line which is parallel to earth from observation point to object is called “horizontal line”.
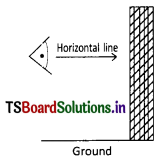
→ Line of sight: It is the line drawn from the eye of an observer to the object viewed.
![]()
→ Angle of elevation : It is the angle formed by the line of sight with the horizontal, when the object viewed is above the horizontal level. In this case, we have to raise our head to look at the object.
→ Angle of depression : It is the angle formed by the line of sight with the horizontal when the object viewed is below the horizontal level. In this case, we have to lower our head to look at the object.
The height or length of an object or the distance between two distant objects can be determined with the help of trigonometric ratios.
→ Trigonometric ratios in a right triangle:
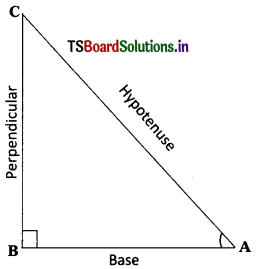
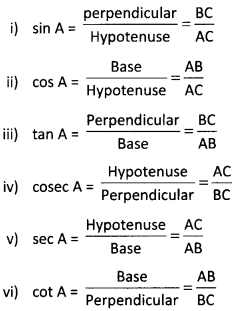
→ Trigonometric ratios of some specific angles :

Solving Procedure :
When we want to solve the problems of height and distances, we should consider the following :
- All the object such as tower, trees, buildings, ships mountains etc. shall be considered as linear for mathematical convenience.
- The angle of elevation or angle of depression is considered with referece to the horizontal line.
- The height of the observer is neglected, if it is not given in the problem.
- To find heights and distances, we need to draw figures and with the help of these figures we can solve the problems.
![]()
Leonard Euler:
- Euler is a Swiss Mathematical genius.
- Euler’s famous formula is F + C = E + 2.
- Euler’s equation is e’q = cos q + i sin q.
- He introduced the notations like S, f(x) etc.