TS 10th Class Maths Important Questions Chapter 6 Progressions

These TS 10th Class Maths Chapter Wise Important Questions Chapter 6 Progressions given here will help you to solve different types of questions.

TS 10th Class Maths Important Questions Chapter 6 Progressions

Previous Exams Questions

Question 1.
Check whether – 25 is a term in the pro-gression 5, 3, 1, … or not ? (A.P. June 15)
Solution:
The given 5, 3, 1, ……. is an arithmetic progression here
a = 5, d = a₂ – a₁ = 3 – 5 = -2
Let – 25 is some of ‘n’ th term
i.e. a_n = – 25
So a_n = a + (n – 1)d
-25 = 5 + (n – 1)(- 2)
– 25 – 5 = (n – 1)(- 2)
\(\frac{-30}{-2}\) = n – 1 = 15
⇒ n = -1 and n = 15 + 1 = 16
So -25 exist at 16^th term in above series.

Question 2.
Find out the common ratio in the GP 2, 2\(\sqrt{2}\), 4,………..
Solution:
The given GP is 2, 2\(\sqrt{2}\), 4, ……….
The common ratio = \(\frac{\mathrm{a}_2}{\mathrm{a}_1}\) = \(\frac{2 \sqrt{2}}{2}\) = \(\sqrt{2}\)

Question 3.
The hand borewell driller charges Rs. 200/- for the first one meter only and raises drilling charges @ 30/- for every subsequent meter. Write a progression for the above data. (T.S Mar. 15)
Solution:
Cost of first meter = Rs. 200/-
For every subsequent meter
= Rs. 30/- raised.
So the progression = 200, 230, 260,………

Question 4.
In a flower garden there are 23 plants in first row, 21 plants in second row, 19 plants in 3rd row and so on. If there are 10 rows in that garden, then find the to-tal number of plants in the last row with the help of the formula t_n = a + (n – 1) d. (T.S. Mar. 16)
Solution:
No. of plants in 1^st row = 23
No. of plants in 2^nd row = 21
No. of plants in 3^rd row = 19 and so on.
So the progression is 23, 21, 19, ………..
in this A.P a = 23, d = 21 – 23 = – 2
n = 10
t_n = a + (n – 1)
t₁₀ = 23 + (10 – 1) (-2)
= 23 + 9 (-2)
= 23 – 18 = 15
Number of plants in the last row = 5.

Question 5.
Find the sum of first 200 natural numbers. (T.S. Mar. 16)
Solution:
Formula for the sum of first n natural numbers is Σ_n = \(\frac{\mathrm{n}(\mathrm{n}+1)}{2}\)
Put n = 200 in above formula.
We get
Σ200 = \(\frac{200 \times(200+1)}{2}\) = \(\frac{200 \times 201}{2}\)
= 20,100

Question 6.
If the sides of a triangle are in AP. The perimeter of the triangle is 30 cm. the difference between the longer and shorter side is 4 cm. Then find all sides of the triangle. (T.S. Mar. 16)
Solution:
Let the 3 sides of given triangle = a – d, a, a + d
Then its perimeter
= a – d + a + a + d = 30 cm.
3a = 30 cm
⇒ a = \(\frac{30}{3}\) = 10 cm.
The larger side = a + d
The shorter side = a – d
The difference between the above two = (a + d) – (a – d) = 4 cm.
a + d – a + d = 4 cm.
2d = 4; d = \(\frac{4}{2}\) = 2 cm.
So the sides a – d = 10 – 2 = 8 cm
a = 10 cm
and a + d = 10 + 2 = 12 cm.
So 8, 10, 12 cm are the sides of the triangle.

Question 7.
Find the stun of all 3 digit numbers that are divisible by 4.
Solution:
The 3 digit numbers are 100, 101, 102,………. 999 among them the number divisible by 4 are 100, 104, 108, … 996 which is an A.P the first term a = 100
Common difference = a₂ – a₁
= 104 – 100 = 4
Let the number of terms = n
The n^th term a_n = 996
a_n = a + (n – 1) d
996 = 100 + (n – 1) 4
\(\frac{996-100}{4}\) = n – 1
⇒ \(\frac{896}{4}\) = n – 1 = 224
⇒ n = 224 + 1 = 225
Now formula for sum of ‘n’ terms in AP is
S_n = \(\frac{\mathrm{n}}{2}\)[a + l]
= \(\frac{225}{2}\)[100 + 996]
= \(\frac{225 \times 1096}{2}\) = 1, 23, 300

Additional Questions

Question 1.
For the following A.P’s write the first term and the common difference.

i) \(\frac{1}{4}\), \(\frac{1}{2}\), \(\frac{3}{4}\), \(\frac{5}{4}\)……….
ii) 5, 8, 11, 14, 17 …….
iii) \(\frac{1}{3}\), 1, \(\frac{5}{3}\), \(\frac{7}{3}\),………
Solution:
i) \(\frac{1}{4}\), \(\frac{1}{2}\), \(\frac{3}{4}\), \(\frac{5}{4}\)……….
Given \(\frac{1}{4}\), \(\frac{1}{2}\), \(\frac{3}{4}\), \(\frac{5}{4}\)……….
First term = \(\frac{1}{4}\) = a = t₁
Common difference = d
= t₂ – t₁ = \(\frac{1}{2}\) – \(\frac{1}{4}\) = \(\frac{1}{4}\)

ii) 5, 8, 11, 14, 17
Solution:
Given 5, 8, 11, 14, 17, ………
First term = 5 = a = t₁
Common difference = d
= t₂ – t₁ = 8 – 5 = 3

iii) \(\frac{1}{3}\), 1, \(\frac{5}{3}\), \(\frac{7}{3}\),……..
Solution:
Given \(\frac{1}{3}\), 1, \(\frac{5}{3}\), \(\frac{7}{3}\),……
Firstterm = \(\frac{1}{3}\) = a = t₁
Common difference = d
= t₂ – t₁
= 1 – \(\frac{1}{3}\) = \(\frac{3-1}{3}\) = \(\frac{2}{3}\)

Question 2.
Write the first four terms of the A.P. when the first term ‘a’ and the common’d’ are given as follows.
i) a = 6, d = – 2
ii) a = – 3, d = 4
iii) a = x + 2y, d = – y
iv) a = 8, d = 5
Solution:
i) a = 6, d = – 2
Given a = 6, d = – 2
First term = t₁ = a = 6
Second term = t₂ = a + d = 6 – 2 = 4
Third term = t₃ = a + 2d = 6 + 2(- 2)
= 6 – 4 = 2
Fourth term = t₄ = a + 3d
= 6 + 3 (-2)
= 6 – 6 = 0
∴First four terms are 6, 4, 2, 0

ii) a = – 3, d= 4
Solution:
Given a = – 3, d = 4
First term = t₁ = a = – 3
t₂ = a + d = -3 + 4 = 1
t₃ = a + 2d = -3 + 2 × 4
= -3 + 8 = 5
t₄ = a + 3d
= -3 + 3 × 4
= – 3 + 12 = 9
First four terms :
∴ First four terms are – 3, 1, 5, 9

iii) a = x + 2y, d = – y
Solution:
Given a = x + 2y, d = -y
First term = a = t₁ = x + 2y
t₂ = a + d
= x + 2y – y
= x + y
t₃ = a + 2d
= (x + 2y) + 2 × (- y)
= x + 2y – 2y
= x
t₄ = a + 3d
= (x + 2y) + 3(-y)
= x + 2y – 3y
= x – y
∴ First four terms are
x + 2y, x + y, x, x – y

iv) a = 8, d = 5
Solution:
Given a = 8, d = 5
First term = a = t₁ = 8
t₂ = a + d
= 8 + 5 = 13
t₃ = a + 2d
= 8 + 2 × 5
= 8 + 10
= 18
t₄ = a + 3d
= 8 + 3 × 5
= 8 + 15
= 23
∴ First four terms are 8, 13, 18, 23

Question 3.
Which of the following are APs ? If they form an AP, find the common difference and write three more terms.
i) 3, 5, 7, 9,……….
ii) 5, 9, 7, 3, ………..
iii) 3, \(\frac{10}{3}\), \(\frac{11}{3}\), 4,……….
iv) 0, – 3, – 6, – 9, -12
v) a, 4a, 7a, 10a …………
Solution:
i) 3, 5, 7, 9,………..
Given 3, 5, 7, 9, …….
Here a₁ = 3, a₂ = 5, a₃ = 7
a₂ – a₁ = 5 – 3 = 2
a₃ – a₂ = 7 – 5 = 2
Since d = a₂ – a₁ = a₃ – a₂ = 2 is equal.
∴ The series form an A.P.
Next three terms = 9 + 2 = 11, 11 + 2 = 13, 13 + 2 = 15
i.e., 11, 13, 15

ii) Given 5, 9, 13, 17
a₁ = 5, a₂ = 9, a₃ = 13
a₂ – a = 9 – 5 = 4
a₃ – a₂ = 13 – 9 = 4
Since d = a₂ – a₁ = a₃ – a₂ = 4 is equal.
∴The series form an A.P. next three terms
17 + 4 = 21
21 + 4 = 25
25 + 4 = 29
i.e., 21, 25, 29

iii) 3, \(\frac{10}{3}\), \(\frac{11}{3}\), 4
Solution:
Given 3, \(\frac{10}{3}\), \(\frac{11}{3}\), 4,………
here a₁ = 3, a₂ = \(\frac{10}{3}\), a₃ = \(\frac{11}{3}\)
a₂ – a₁ = \(\frac{10}{3}\) – 3 = \(\frac{10-9}{3}\) = \(\frac{11}{3}\)
a₃ – a₂ = \(\frac{11}{3}\) – \(\frac{10}{3}\) = \(\frac{11-10}{3}\) = \(\frac{1}{3}\)
Since a₂ – a₁ = a₃ – a₂ = \(\frac{1}{3}\) = d isequal.
∴ The series form an AP
Next three terms are = 4 + \(\frac{1}{3}\) = \(\frac{12-11}{3}\) = \(\frac{13}{3}\)
\(\frac{13}{3}\) + \(\frac{1}{3}\) = \(\frac{14}{3}\), \(\frac{14}{3}\) + \(\frac{1}{3}\) = \(\frac{14+1}{3}\) = \(\frac{15}{3}\) = 5
i.e., \(\frac{13}{3}\), \(\frac{14}{3}\), 5

iv) 0, -3, -6, -9, -12,……….
Solution:
Given 0, – 3, – 6, – 9, – 12,
Here a₁ = 0, a₂ = – 3, a₃ = – 6
a₂ – a₁ = -3 – 0 = -3
a₃ – a₂ = -6 – (-3)
= -6 + 3 = -3
Since a₂ – a₁ = a₃ – a₂ = – 3 = d is equal.
∴ The series form an A.P
Next three terms are = – 12 – 3
= -15
= -15 – 3
= -18
= -18 – 3
= -21
i.e.,-15,-18,-21

v) a, 4a, 7a, 10a ……….
Solution:
Given a, 4a, 7a, 10a, ……….
Here a₁ = a, a₂ = 4a, a₃ = 7a
a₂ – a₁ = 4a – a = 3a
a₃ – a₂ = 7a – 4a = 3a
Since a₂ – a₁ = a₃ – a₂ = 3a = d is equal.
∴ The series form an A.P
Next three terms are: 10a + 3a = 13a
13a + 3a = 16a, 16a + 3a = 19a
i.e., 13a, 16a, 19a.

Question 4.
Fill in the blanks in the following table.

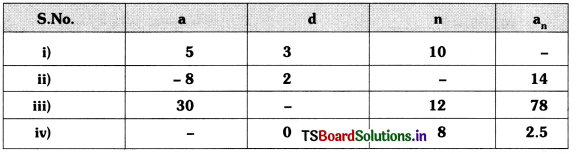
Solution:

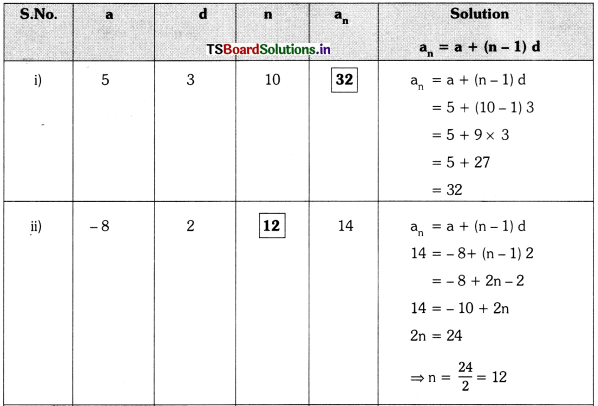
Question 5.
Find the
i) 25^th term of the A.P.: 8, 11, 14,………
ii) 10^th term of the A.P.
– 10, -6, -2,…….
Solution:
i) Given A.P: 8, 11, 14, ………
Here a₁ = 8, d = a₂ – a₁
= 11 – 8 = 3
a_n = a + (n – 1)d
a₂₅ = 8 + (25 – 1) × 3
= 8 + 24 × 3
= 8 + 72
= 80

ii) Given A.P : – 10, – 6, – 2, ………
Here a₁ = – 10,
d = a₂ – a₁
= – 6 – (-10)
= -6 + 10 = 4
a_n = a + (n – 1) d
a₁₀ = -10 + (10 – 1)4
= -10 + (10 – 1)4
= -10 + 9 × 4
= – 10 + 36
= 26

Question 6.
Which term of the A.P 5, 8, 11, 14, ….. is 47?
Solution:
Given A.P : 5, 8, 11, 14,……….
Here a = 5, d = a₂ – a₁ = 8 – 5 = 3
Let 47 be the nth term of the given A.P
∴ a_n = a + (n – 1) d
47 = 5 + (n – 1) 3
= 5 + 3n – 3
47 = 2 + 3n
⇒ 47 – 2
3n = 45
⇒ n = \(\frac{45}{3}\) = 15
∴ 47 is the 15th term of given A.P

Question 7.
Find the number of terms of the A.P.
7, 12, 17,…… 152.
Solution:
Given AP : 7, 12, 17,…….. 152
Here a = 7,
d = a₂ – a₁ = 12 – 7 = 5
Let 152 be the n^th term of the given A.P
Then a_n = a + (n – 1)d
152 = 7 + (n – 1)5
152 = 2 + 5n
⇒ 152 – 2 = 5n
⇒ 5n = 150
⇒ n = \(\frac{150}{5}\)
∴ 30 terms are there in the given AP

Question 8.
Find the 21^st term of an A.P Whose 11^th term is 92 and 16^th term is 122.
Solution:
Given an AP whose

d = \(\frac{30}{5}\) = 6
Substituting d = 6 in equation (1)
a + 10 × 6 = 92
a + 60 = 92
⇒ a = 92 – 60 = 32
Now the 21^st term = a + 20d
= 32 + 20 × 6
= 32 + 120 = 152

Question 9.
Find the sum of the following APs.
i) 4, 9, 14, …… to 14 terms
ii) – 32, – 28, – 24, …… to 12 terms.
Solution:
i) Given 4, 9, 14, …….. to 14 terms
Here a = 4, d = a₂ – a₁ = 9 – 4 = 5
n = 14
S_n = \(\frac{n}{2}\)[2a + (n – 1)d]
S₁₄ = \(\frac{14}{2}\)[2 × 4 + (14 – 1) × 5]
= 7 [8 + 13 × 5]
= 7 [8 + 65]
= 7 × 73
= 511

ii) – 32, – 28, – 24, ……. to 12 terms.
Solution:
Given -32, – 28, – 24, …… to 12 terms
Here a = – 32, d = a₂ – a₁
= -28 – (- 32)
= – 28 + 32 = 4
and n = 12
S_n = \(\frac{n}{2}\)[2a + (n – 1)d]
S₁₂ = \(\frac{12}{2}\) [2 × (-32) + (12 – 1) × 4]
= 6 [-64 + 11 × 4]
= 6[-20]
= -120

Question 10.
In an A.P. given a = 5, d = 6, a_n = 89, find n and S_n.
Solution:
Given a = 5, d = 6, a_n = 89
⇒ a + (n – 1) d = 89
⇒ 5 + (n – 1) 6 = 89
⇒ 5 + 6n – 6 = 89
⇒ 6n – 1 = 89
⇒ 6n = 89 + 1 = 90
S_n = \(\frac{n}{2}\)[a + l]
= \(\frac{15}{2}\)[5 + 89]
= 15 × 47 = 705
∴ S₁₅ = 705

Question 11.
In an A.P. given a = 6, a₁₃ = 12 = (l) find d and S₁₃.
Solution:
Given a = 6, a₁₃ = 12
a₁₃ = a + 12d = 12
6 + 12 × d = 12
⇒ 12d = 12 – 6 = 6
⇒ d = \(\frac{6}{12}\) = \(\frac{1}{2}\)
Now S_n = \(\frac{n}{2}\)[a + l]
S₁₃ = \(\frac{13}{2}\)[6+12]
S₁₃ = \(\frac{13}{2}\)[18] = 13 × 9 = 117

Question 12.
In an A.P. given a₁₄ = 57, d = 4. Find ‘a’ and S₁₀.
Solution:
Given a₁₄ = a + 13d = 57 (= l) and d = 4
a + 13 × 4 = 57
a + 52 = 57
⇒ a = 57 – 52 = 5
Now S_n = \(\frac{n}{2}\)[a + l]
S₁₀ = \(\frac{10}{2}\)[5+5]
= 5 [62]
S₁₀ = 310

Question 13.
In an A.P. a_n = 10, d = 3, S_n = 15 find n and ‘a’.
Solution:
Given a_n = a + (n – 1) d = 10,
d = 3, S_n = 15
⇒ a + (n – 1) 3 = 10
⇒ a + 3n – 3 = 10
⇒ a + 3n = 13
⇒ a = 13 – 3 n
S_n = \(\frac{n}{2}\)[a + a_n]
= \(\frac{n}{2}\)[13 – 3n + 10]
15 × 2 = n[23 – 3n]
30 = 23n – 3n²
⇒ 3n² – 23n + 30 = 0
⇒ 3n² – 18n – 5n + 30 = 0
⇒ 3n(n – 6) – 5(n – 6) = 0
⇒ (n – 6) (3n – 5) = 0
⇒ n – 6 = 0 or 3n – 5 = 0
∴ n = 6; a = \(\frac{15}{3}\) (n cannot be fraction)
a = 13 – 3n
= 13 – 3 × 6
a = 13 – 18 = – 5
∴ a = – 5, n = 6

Question 14.
If the sum of first 7 terms of an A.P is 77 and that of 17 terms is 442, find the sum of first “n” terms.
Solution:
Given A.P. such that
S₇ = 77
S₁₇ = 442
We know that S_n = \(\frac{n}{2}\)[2a + (n – 1) d]
S₇ = 77
\(\frac{7}{2}\)[2a + (7 – 1)d] = 77
⇒ 2a + 6d = \(\frac{77 \times 2}{7}\)
⇒ 2a + 6d = 22 (Dividing by 2)
⇒ a + 3d = 11 —– (1)
S₁₇ = 442
\(\frac{17}{2}\)[2a + (17 – 1)d] = 442
⇒ 2a + 16d = 52 (Dividing by 2)
⇒ a + 8d = 26 —— (2)

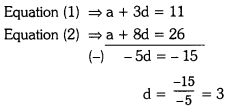
Substitute d = 3 in equation (1)
a + 3 × 3 = 11
⇒ a + 9 = 11
⇒ a = 11 – 9 = 2
∴ a = 2, d = 3
Now S_n = \(\frac{n}{2}\)[2a + (n – 1) d]
= \(\frac{n}{2}\)[2 × 2 + (n – 1)3]
= \(\frac{n}{2}\)[4 + 3n – 3]
S_n = \(\frac{n}{2}\)[3n + 1]
∴ Sum of first n terms = Sn = \(\frac{n}{2}\)(3n + 1)

Question 15.
Write the terms of the G.P. When the first term ‘a’ and the common ratio ‘r’ are given.
i) a = 5, r = 2
ii) a = \(\sqrt{3}\), r = \(\frac{1}{2}\)
iii) a = 16, r = –\(\frac{1}{2}\)
Solution:
i) a = 5, r = 2
The terms of G.P are a, ar, ar², ar³, ……….
i.e., 5, 5 × 2, 5 × 2², 5 × 2³,………..
⇒ 5, 10, 20, 40, ……..

ii) a = \(\sqrt{3}\), r = \(\frac{1}{2}\)
The terms of G.P are a, ar, ar², ar³, ……….
i.e., \(\sqrt{3}\), \(\sqrt{3}\) × \(\frac{1}{2}\), \(\sqrt{3}\) × \(\frac{1}{2^2}\), \(\sqrt{3}\) × \(\frac{1}{2^3}\)

iii) a = 16, r = –\(\frac{1}{2}\)
The terms of G.P are a, ar, ar², ar³, ………
i.e., 16, 16 × \(\left(\frac{-1}{2}\right)\), 16 × \(\left(\frac{-1}{2}\right)^2\), 16 × \(\left(\frac{-1}{2}\right)^3\) ………
⇒ 16, -18, 4, -2,……..

Question 16.
Which of the following are GP ? If them are G.P., write 3 more terms.

i) 3, 15, 75,………
ii) \(\frac{1}{2}\), –\(\frac{1}{6}\), \(\frac{1}{18}\),………
iii) a = \(\frac{1}{2}\), r = –\(\frac{1}{3}\)
iv) – 5 – 10, – 20,………
v) – 0.3, – 0.03, – 0.003,………..
Solution:
i) Given 3, 15, 75, ………
When a₁ = 3, a₂ = 15, a₃ = 75
\(\frac{\mathrm{a}_2}{\mathrm{a}_1}\) = \(\frac{15}{3}\) = 5, \(\frac{\mathrm{a}_3}{\mathrm{a}_2}\) = \(\frac{75}{15}\) = 5
∴ r = \(\frac{\mathrm{a}_2}{\mathrm{a}_1}\) = \(\frac{\mathrm{a}_3}{\mathrm{a}_2}\) = 5
Hence 3, 15, 75, ……. is a G.P
When a = 3, r = 5
a₄ = a.r³ = 3 × (5)³ = 3 × 125 = 375
a₅ = a.r⁴ = 3 × (5)⁴ = 3 × 625 = 1,875
a₆ = a.r⁵ = 3 × (5)⁵ = 3 × 3125 = 9,375

ii) \(\frac{1}{2}\), –\(\frac{1}{6}\), \(\frac{1}{18}\),………
Solution:
Given a₁ = \(\frac{1}{2}\), a₂ = –\(\frac{1}{6}\), a₃ = \(\frac{1}{18}\)
\(\frac{\mathrm{a}_2}{\mathrm{a}_1}\) = \(\frac{-1 / 6}{1 / 2}\) = \(\frac{-1}{6}\) × \(\frac{2}{1}\) = \(\frac{-1}{3}\)
\(\frac{\mathrm{a}_3}{\mathrm{a}_2}\) = \(\frac{1 / 18}{-1 / 6}\) = \(\frac{1}{18}\) × \(\frac{-6}{1}\) = \(\frac{-1}{3}\)
Here, \(\frac{a_2}{a_1}\) = \(\frac{a_3}{a_2}\) = r = \(\frac{-1}{3}\)
Given terms are in G.P.

iii) a = \(\frac{1}{2}\), r = –\(\frac{1}{3}\)
Solution:

iv) -5, -10, -20,………
Solution:
Given a₁ = -5, a₂ = -10, a₃ = -20
\(\frac{a_2}{a_1}\) = \(\frac{-10}{-5}\) = 2, \(\frac{a_3}{a_2}\) = \(\frac{-20}{-10}\) = -2
Since \(\frac{a_2}{a_1}\) = \(\frac{a_3}{a_2}\) = 2 = r
∴ Given is in G.P
a₄ = ar³ = -5 × (2)³ = -5 × 8 = -40
a₅ = ar⁴ = -5 × (2)⁴ = -5 × 16 = -80
a₆ = ar⁵ = -5 × (2)⁵ = -5 × 32 = -160

v) 0.3, 0.03, 0.003,……..
Solution:
Given a₁ = 0.3, a₂ = 0.03, a₃ = 0.003
Since \(\frac{a_2}{a_1}\) = \(\frac{0.03}{0.3}\) = 0.1, \(\frac{a_3}{a_2}\) = \(\frac{0.003}{0.03}\) = 0.1
\(\frac{a_2}{a_1}\) = \(\frac{a_3}{a_2}\) = 0.1 = r
∴ Given is in GP
a₄ = ar³ = 0.3 × (0.1)³ = 0.0003
a₅ = ar⁴ = 0.3 × (0.1)⁴ = 0.00003
a₆ = ar⁵ = 0.3 × (0.1)⁵ = 0.000003

Question 17.
For each geometric progression find the common ratio ‘r’ and then find a_n.

i) 2, \(\frac{2}{3}\), \(\frac{2}{9}\), \(\frac{2}{27}\),……..
ii) -3, -6, -12, -24,……..
Solution:
i) Given G.P. : 2, \(\frac{2}{3}\), \(\frac{2}{9}\), \(\frac{2}{27}\),………
Here a = 2, r = \(\frac{a_2}{a_1}\) = \(\frac{2}{\frac{3}{2}}\) = \(\frac{1}{3}\)
a_n = ar^n-1 = 2.\(\left(\frac{1}{3}\right)^{\mathrm{n}-1}\)

ii) -3, -6, -12, -24,……
Solution:
Given GP: -3, -6, -12, -24,……..
Here a₁ = -3, r = \(\frac{a_2}{a_1}\) = \(\frac{-6}{-3}\) = 2
a_n = ar,sup>n-1 = (-3) × (2),sup>n-1 = -3 × 2^n-1

Question 18.
Find the 8^th and n^th term of GP:
6, 18, 54,……….
Solution:
Given GP = 6, 18, 54,……….
Here a = 6. r = \(\frac{a_2}{a_1}\) = \(\frac{18}{6}\) = 3
We know that a = ar^n-1
∴ a₈ = ar⁷ = 6 × 3⁷
a_n = a^{n – 1} = 6.3^{n – 1}

Question 19.
Find the indicated term of each GR
i) a₁ = 8, r = \(\frac{1}{2}\), find a.
ii) a₁ = -10, r = \(\frac{1}{2}\); find a₅
Solution:
i) a₁ = 8, r = \(\frac{18}{6}\), find a₈.
a_n = a.r^{n – 1} (∴ a₁ = a = 8)
a₈ = a.r^{n – 1}
= 8.\(\left(\frac{1}{2}\right)^{8-1}\) = 8.\(\left(\frac{1}{2}\right)^7\) = 2³ × \(\frac{1}{27}\)
= \(\frac{1}{2^{7-3}}\) = \(\frac{1}{2^4}\)
∴ a₈ = \(\frac{1}{2^4}\)

ii) a₁ = -10, r = \(\frac{1}{5}\) ; find a₅
Solution:
a_n = ar^n-1
a₅ = (-10) × \(\left(\frac{1}{5}\right)^{5-1}\)
= (-2 × 5) × \(\frac{1}{5^4}\) = -2 × \(\frac{5}{5^4}\)
∴ a₅ = \(\frac{-2}{5^3}\)

Question 20.
Which term of the G.P.

i) 3,9,27, …… is 729.
ii) \(\sqrt{2}\), 2, 2\(\sqrt{2}\), ….. is 256.
iii) \(\frac{1}{5}\), \(\frac{1}{25}\), \(\frac{1}{125}\),……. is \(\frac{1}{3125}\)
Solution:
i) 3, 9, 27, ……. is 729.
Here a = 3, r = \(\frac{\mathrm{a}_2}{\mathrm{a}_1}\) = \(\frac{9}{3}\) = 3
Let the n^th term of GP be 729.
a_n = ar^n-1
729 = 3. (3)^n-1 = 3. \(\frac{3^{\mathrm{n}}}{3}\)
729 = 3ⁿ
⇒ 3ⁿ = 3ⁿ = 729 = 3⁶
[∵ bases are equal, exponents are also equal]
∴ n = 6
∴ 729 is the 6th term of given G.P

ii) \(\sqrt{2}\), 2, 2\(\sqrt{2}\), …… is 256.
Solution:
Given \(\sqrt{2}\), 2, 2\(\sqrt{2}\), ……… is 256
Here a = \(\sqrt{2}\), r = \(\frac{2}{\sqrt{2}}\) = \(\frac{\sqrt{2} \times \sqrt{2}}{\sqrt{2}}\) = \(\sqrt{2}\)
Let the n^th term of G.P be 256.
a_n = ar^n-1
⇒ 256 = \(\sqrt{2}\). (\(\sqrt{2}\))^{n – 1} = \(\sqrt{2}\). \(\frac{(\sqrt{2})^{\mathrm{n}}}{\sqrt{2}}\).
⇒ 2⁸ = (\(\sqrt{2}\))ⁿ = (2^1/2)ⁿ = \(2^{\frac{n}{2}}\)
⇒ \(\frac{\mathrm{n}}{2}\) = 8 [∵ Bases are equal, exponents are also equal.]
⇒ n = 2 × 8 = 16
∴ 256 is the 16^th term of given G.P

iii) \(\frac{1}{5}\), \(\frac{1}{25}\), \(\frac{1}{125}\),……. is \(\frac{1}{3125}\)
Solution:

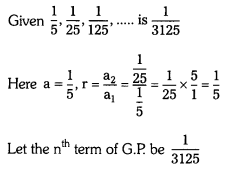
a_n = ar^n-1

[∵ Bases are equal, exponents are equal.]
∴ \(\frac{1}{3125}\) is the 5^th term of given G.P

Question 21.
Find the 10^th term of a G.P. Whose 8^th term is 384 and the common ratio is 2.
Solution:
Given a G.P such that a₈ = 384 and r = 2
We know that a_n = ar^n-1
⇒ a₈ = ar⁷
⇒ 384 = a. 2⁷
⇒ a = \(\frac{384}{2^7}\) = \(\frac{384}{128}\) = 3
∴ a = 3
Now a₁₀ = ar⁹ = 3.2⁹
= 3 × 512 = 1536
∴ 10^th term of GP is 1536

Question 22.
A contractor construction job specifies a penalty for delay of completion beyond a certain date as follows. ₹ 200 for the first day. The penalty for each succeeding day being Rs. 50 more than the preceding day. How much money does the contractor pay as penalty if he has delayed the work by 30 days. (AP SCERT Model Paper-2016)
Solution:
From the data we have
a = 200, d = 50, n = 30
S_n = \(\frac{n}{2}\)[2a + (n – 1)d]
S₃₀ = \(\frac{n}{2}\)[2(200) + (30 – 1) 50]
= 15 [400 + 1,450]
= 15 [1,850] = 27,750
The amount he has to pay as penalty ₹ 27,750