TS Inter 2nd Year Maths 2B Solutions Chapter 8 Differential Equations Ex 8(a)

Students must practice this TS Intermediate Maths 2B Solutions Chapter 8 Differential Equations Ex 8(a) to find a better approach to solving the problems.

TS Inter 2nd Year Maths 2B Solutions Chapter 8 Differential Equations Ex 8(a)

I.
Question 1.
Find the order of the differential equation obtained by eliminating the arbitrary constants b and c from xy = ce^x+ be^-x + x².
Solution:
Given equation is xy = ce^x + be^-x + x² ………….(1)
Differentiating (1) w.r.t x, we get
xy₁ + y = ce^x – be^-x + x²
Again differentiating w.r.t x, we get
xy₂ + y₁ + y₁ = ce^x + be^-x + 2
= (xy – x²) + 2
∴ xy₂ + 2y₁ – (xy – x²) – 2 = 0 ………………(2)
Arbitrary constants a and b are eliminated in the differential equation (2).
The order of the differential equation (2) is 2.

Question 2.
Find the order of the differential equation of the family of all circles with their cen¬tres at the origin.
Solution:
The equation of circle with centre 0 is given by x² + y² = a² where a is any constant.
Differentiating w.r.t x we get 2x + 2yy₁ = 0
⇒ x + yy₁ = 0
Which is the required differential equation of family of all circles with their centres at origin.
The order of the above differential equation is 1.

II.
Question 1.
Form the differential equations of the following family of curves where parameters are given in brackets.
(i) y = c (x – c)²; (c)
Given y = c(x – c)² ……………..(1)
Differentiating w.r.t ‘x’ we have
y₁ = 2c(x – c) ………….(2)

∴ y . y₁³ = (xy₁ – 2y) 4y²
⇒ y₁³ = (xy₁ – 2y) 4y
⇒ y₁³ = 4xyy₁ – 8y²
⇒ y₁³ – 4xyy₁ + 8y² = 0
⇒ \(\left(\frac{d y}{d x}\right)^3\) – 4xy \(\frac{d y}{d x}\) + 8y² = 0
This the differential equation in which c is eliminated.

ii) xy = ae^x + be^-x ; (a, b)
Solution:
Given xy = ae^x + be^-x and ………….(1)
Differentiating (1) w.r.t x
xy₁ + y = ae^x – be^-x
Again differentiating w.r.t x,
xy₂ + y₁ + y₁ = ae_x + be_-x = xy
= xy₂ + 2y₁ – xy = 0
which is the required equation obtained on the elimination of a and b.

(iii) y = (a + bx) e^kx; (a, b)
Solution:
Given y = (a + bx) e^kx …………(1)
and Differentiating (1) w.r.t x, we get
y₁ = (a + bx) ke^kx + e^kx . b
= ky + e^kx . b
∴ y₁ – ky = be^kx ………….(2)
Again differentiating w.r.t x,
y₂ – ky₁ = kbe^kx
= k(y₁ – ky)
⇒ y₂ – 2ky₁ + k²y = 0
⇒ \(\frac{d^2 y}{d x^2}-2 \mathrm{k} \frac{d y}{d x}\) + k²y = 0
is the required equation obtained on the elimination of a, b.

v) y = a cos (nx + b); (a, b)
Solution:
Given equation is y = a cos (nx + b)
∴ y₁ = – an sin (nx + b)
= – an² cos (nx + b)
= – n²y
∴ y₁ + n²y = 0
⇒ \(\frac{d^2 \mathrm{y}}{d x^2}\) + n²y = 0
is the required differential equation obtained on elimination of a and b.

Question 2.
Obtain the differential equation which corresponds to each of the following family of curves.
(i) The rectangular hyperbolas which have the coordinate axes as asymptotes.
Solution:
Equation of rectangular hyperbolas which have the coordinate axes as asymptotes is
xy = c².
Differentiating w.r.t x,
xy₁ + y = 0
⇒ x\(\frac{d y}{d x}\) + y = 0 is the required equation.

(ii) The ellipses with centres at the origin and having coordinate axes as axes.
Solution:
Equation of ellipse is \(\frac{x^2}{a^2}+\frac{y^2}{b^2}\) = 1
Differentiating w.r.t x’ we get,

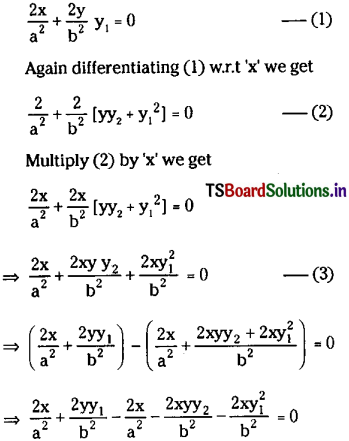
⇒ \(\frac{2}{\mathrm{~b}^2}\) [yy₁ – xyy₂ – xy₁²] = 0
⇒ yy₁ – xyy₂ – xy₁² = 0
⇒ xyy₂ + xy₁ – yy₁ = 0
⇒ \(x y \frac{d^2 y}{d x^2}+x\left(\frac{d y}{d x}\right)^2-y \frac{d y}{d x}=0\) is the required differential equation.

III.
Question 1.
Form the differential equations of the following family of curves whose parameters are given in brackets.
(i) y = ae^3x + be^4x; (a, b)
Solution:
Given y = ae^3x + be^4x
Differentiating w.r.t. ‘X’
y₁ = 3ae^3x + 4be^4x
⇒ y₁ – 3ae^3x = 4be^4x
= 4 [y – ae^3x]
⇒ y₁ – 4y = ae^3x ………….(1)
Again differentiating w.r.t. x,
y₂ – 4y₁ = – 3ae^3x
⇒ y₂ – 4y₁ = 3 (y₁ – 4y)
⇒ y₂ – 7y₁ + 12y = 0 is the required differential equation.

(ii) y = ax² + bx, (a, b)
Solution:
Given equation is
y = ax² + bx …………..(1)
and dill erentiating w.r.t. x
y₁ = 2ax + b …………(2)
Again differentiating w.r.t. x,
y₂ = 2a
⇒ x²y₂ = 2ax² …………..(3)
Also from (2)
– 2xy₁ = – 4x²a – 2bx …………..(4)
From (1)
2y = 2ax² + 2bx …………(5)
Adding (3), (4), (5) we get
x²y₂ – 2xy₁ + 2y = 2ax² – 4ax² – 2bx + 2ax² + 2bx = 0
∴ \(x^2 \frac{d^2 y}{d x^2}-2 x \frac{d y}{d x}+2 y=0\) is the required differential equation in which a, b are eliminated.

(iii) ax² + by² = 1; (a, b)
Solution:
Given equation of the curve is
ax² + by² = 1 ……………(1)
Differentiating (1) w.r.t. ‘x we get
2ax + 2by \(\frac{d y}{d x}\) = 0 and
by² = 1 – ax² from (1)
⇒ 2ax + 2byy₁ = 0 ……………(2)
⇒ b(2yy₁) = – 2ax …………….(3)
From (3) + (2) we get

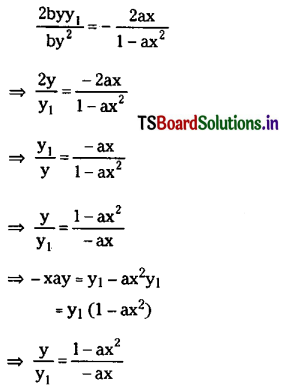
⇒ – xay = y₁ (1 – ax²)
⇒ – axy = y₁ – ax²y₁
⇒ y₁ = ax (xy₁ – y)
⇒ a = \(\frac{y_1}{x\left(x y_1-y\right)}\)
Differentiating w.r.t x,
0 = \(\frac{d}{d x}\left[\frac{y_1}{x\left(x y_1-y\right)}\right]\)
= \(\frac{y_2\left(x^2 y_1-x y\right)-y_1\left(\frac{d}{d x}\left(x^2 y_1-x y\right)\right)}{x^2\left(x y_1-y\right)^2}\)
⇒ (x²y₁ – xy)y₂ – y₁(x²y₂ + 2xy₁ – xy₁ – y) = 0
⇒ x²y₁y₂ – xyy₁ – x²y₁y₂ – 2xy₁² + xy₁² + yy₁ = 0
⇒ xyy₂ + xy₁² – yy₁ = 0
⇒ \(x y \frac{d^2 y}{d x^2}+x\left(\frac{d y}{d x}\right)^2-y\left(\frac{d y}{d x}\right)\) = 0 is the required differential equation obtained on elimination of constants a and b.

(iv) xy = ax² + \(\frac{b}{x}\); (a, b)
Solution:
Given equation is x²y = ax³ + b ………….(1)
Differentiating (1) w.r.t. ‘x’
2xy + x²y₁ = 3ax² ………….(2)
Again differentiating w.r.t x,
x²y₂ + 2xy₁ + 2xy₁ + 2y = 6ax
⇒ x²y₂ + 4xy₁ + 2y = 6ax
⇒ x³y₂ + 4x2y₁ + 2xy = 6ax²
= 2(3ax²)
= 2 [2xy + x²y₁]
= 2x²y₁ + 4xy
⇒ x³y² + 2x²y₁ – 2xy = 0
⇒ x²y₂ + 2xy₁ – 2y = 0
⇒ \(x^2 \frac{d^2 y}{d x^2}+2 x \frac{d y}{d x}\) – 2y = 0 which is the required differential equation on elimination of constants a and b from (1).

Question 2.
Obtain the differential equation which corresponds to each of the following family of curves.
(i) The circles which touch the Y – axis at the origin.
Solution:
The cquation of circle which touch the Y-axis at the origin is x² + y² + 2gx = 0 ………..(1)
Differentiating wr.t. x we get
2x + 2yy₁ + 2g = 0
⇒ g = – (x + yy₁)
Hence from (1)
x² + y² + 2x [- (x + yy₁)] = 0]
x² + y² – 2x² – 2xyy₁ = 0
⇒ – y² – x² = 2xy . \(\frac{d y}{d x}\) which is the required differential equation obtained on elimination of ‘g’ from (1).

(ii) The parabola each of which has a laws rectum 4a and whose axis are parallel to X- axis.
Solution:
Equation of parabola which has latus rectum 4a and whose axes are parallel to X-axis is
(y – k)² = 4a(x – h) ………….(1)
Differentiating w.r.t ‘x’
2 (y – k) y₁ = 4a
⇒ (y – k) y₁ = 2a ……………(2)
Differentiating again w.r.t ‘x’
(y – k) y₂ + y₁² = 0
From (2)
y – k = \(\frac{2 a}{y_1}\)
∴ From (3)
\(\frac{2 a}{y_1}\) y₂ + y₁² = 0
⇒ 2ay₂ + y₁³ = 0
⇒ 2a \(\frac{d^2 y}{d x^2}+\left(\frac{d y}{d x}\right)^3\) = 0 which is the required differential equation obtained on elimination of constants h, k from (1).

(iii) The parabolas having their focli at the origin and axis along the X-axis.
Solution:
Equation of parabola having focii at origin and axis is along X-axis is given by
y² = 4a(x + a) ……….(1)
Differentiating w.r.t x
2yy₁ = 4a
a = \(\frac{\mathrm{yy}_1}{2}\)
∴ From (1)
y² = 4a(x + a)
= 4 \(\frac{\mathrm{yy}_1}{2}\) (x + \(\frac{\mathrm{yy}_1}{2}\))
= 2yy₁ (x + \(\frac{\mathrm{yy}_1}{2}\))
= 2xyy₁ + y²y₁²
⇒ y = 2xy₁ + yy₁²
⇒ yy₁² + 2xy₁ – y = 0
⇒ \(y\left(\frac{d y}{d x}\right)^2+2 x\left(\frac{d y}{d x}\right)\) – y = 0
which is the required differential equation obtained on elimination of ‘a’ from (1).