TS Inter 2nd Year Maths 2B Solutions Chapter 3 Parabola Ex 3(b)

Students must practice this TS Intermediate Maths 2B Solutions Chapter 3 Parabola Ex 3(b) to find a better approach to solving the problems.

TS Inter 2nd Year Maths 2B Solutions Chapter 3 Parabola Exercise 3(b)

I.

Question 1.
Find the equations of the tangent and normal to the parabola y² = 6x at the positive end of the latus rectum.
Solution:
Given equation of parabola is y² = 6x ……..(1)
Comparing with y² = 4ax we get 4a = 6
⇒ a = \(\frac{3}{2}\)
Let L be the positive end of the latus rectum of the parabola y² = 6x
∴ L = (a, 2a) = (\(\frac{3}{2}\), 3)
The equation of tangent to the parabola (1) at (\(\frac{3}{2}\), 3) is yy₁ – 2a(x + x₁) = 0
y(3) = 3(x + \(\frac{3}{2}\))
⇒ 3y = 3x + \(\frac{9}{2}\)
⇒ 6y = 6x + 9
⇒ 2x – 2y + 3 = 0 ……(2)
The equation of normal to the parabola (1) at (\(\frac{3}{2}\), 3) is

Question 2.
Find the equation of tangent and normal to the parabola x² – 4x – 8y + 12 = 0 at (4, \(\frac{3}{2}\)).
Solution:
Given equation of parabola is x² – 4x – 8y + 12 = 0 ……..(1)
Let P(x₁, y₁) = P(4, \(\frac{3}{2}\)) be the given point.
Differentiating (1) w.r.t x we get
2x – 4 – 8 \(\frac{d y}{d x}\) = 0
⇒ \(\frac{d y}{d x}=\frac{x-2}{4}\)
∴ The slope of the tangent at (4, \(\frac{3}{2}\)) is m = \(\frac{4-2}{4}=\frac{2}{4}=\frac{1}{2}\)
and slope of the normal at (4, \(\frac{3}{2}\)) is -2
The equation of a tangent to the parabola (1) at (4, \(\frac{3}{2}\)) is y – y₁ = m(x – x₁)
⇒ y – \(\frac{3}{2}\) = \(\frac{1}{2}\) (x – 4)
⇒ 2y – 3 = x – 4
⇒ x – 2y – 1 = 0 ………(2)
The equation of normal to the parabola (1) at (4, \(\frac{3}{2}\)) is y – y₁ = \(-\frac{1}{m}\)(x – x₁)
⇒ y – \(\frac{3}{2}\) = -2(x – 4)
⇒ 2y – 3 = -4(x – 4)
⇒ 4x + 2y – 19 = 0

Question 3.
Find the value of k if the line 2y = 5x + k is a tangent to the parabola y² = 6x.
Solution:
Given the equation of the line is 2y = 5x + k.
⇒ y = \(\frac{5}{2} x+\frac{k}{2}\) which is of the form y = mx + c where m = \(\frac{5}{2}\) and c = \(\frac{k}{2}\)
Comparing y² = 6x with y² = 4ax we get
4a = 6 ⇒ a = \(\frac{3}{2}\)
The condition for the line y = mx + c to be a tangent to the parabola y² = 4ax is y = mx + \(\frac{a}{m}\)
Where c = \(\frac{a}{m}\)
∴ \(\frac{k}{2}=\frac{3 / 2}{5 / 2}=\frac{3}{5}\)
⇒ k = \(\frac{6}{5}\)

Question 4.
Find the equation of normal to the parabola y² = 4x which is parallel to y – 2x + 5 = 0.
Solution:
Given equation of parabola is y² = 4x ………(1)
Comparing with y² = 4ax we get a = 1
Given y – 2x + 5 = 0, the slope, m = 2
∴ The slope of the line parallel to the above line is ‘2’.
The equation of normal to the parabola which is parallel to the line is having slope m = 2 is
y = mx – 2am – am³
= 2x – 4 – 8
= 2x – 12
⇒ 2x – y – 12 = 0
∴ The equation of the required normal is 2x – y – 12 = 0.

Question 5.
Show that the line 2x – y + 2 = 0 is a tangent to the parabola y² = 16x. Find also the point of contact.
Solution:
Given line is 2x – y + 2 = 0
and slope m = 2, and c = 2.
Comparing y² = 16x with y² = 4ax
we have a = 4
Condition for a line y = mx + c to be a tangent to the parabola y² = 4ax is c = \(\frac{a}{m}\)
∴ c = 2 and \(\frac{\mathrm{a}}{\mathrm{m}}=\frac{4}{2}\) = 2
∴ c = \(\frac{a}{m}\)
The point of contact = \(\left(\frac{a}{m^2}, \frac{2 a}{m}\right)\) = (1, 4)

Question 6.
Find the equation of a tangent to the parabola y² = 16x inclined at an angle of 60° with its axis and also find the point of contact.
Solution:
Given parabola is y² = 16x ……..(1)
Comparing with y² = 4ax we get 4a = 16
⇒ a = 4
Given that inclination of the tangent line is 60°.
∴ Slope of the tangent is m = tan 60° = √3
∴ The equation of the tangent to the parabola (1) having slope √3 is y = mx + \(\frac{a}{m}\)
⇒ y = √3x + \(\frac{4}{\sqrt{3}}=\frac{3 x+4}{\sqrt{3}}\)
⇒ √3y = 3x + 5
⇒ 3x – √3y + 4 = 0
∴ Point of contact = \(\left(\frac{a}{m^2}, \frac{2 a}{m}\right)=\left(\frac{4}{3}, \frac{8}{\sqrt{3}}\right)\)

II.

Question 1.
Find the equations of tangents to the parabola y² = 16x which are parallel and perpendicular respectively to the line 2x – y + 5 = 0, also find the coordinates of their points of contact.
Solution:
Given equation of parabola y² = 16x …….(1)
and comparing with y² = 4ax we have 4a = 16
⇒ a = 4
Equation of any line parallel to the given line 2x – y + 5 = 0 is 2x – y + k = 0 …….(1)
⇒ y = 2x + k
∴ m = 2 and c = k
if (1) is a tangent to the parabola, then c = \(\frac{a}{m}\)
⇒ k = 2
∴ The equation of the required tangent is y = 2x + 2
⇒ 2x – y + 2 = 0
Point of contact = \(\left(\frac{a}{m^2}, \frac{2 a}{m}\right)\) = (1, 4)
Equation of any line perpendicular at 2x – y + 5 = 0 is x + 2y + k = 0
⇒ 2y = -x – k ………(2)
⇒ y = \(-\frac{\mathrm{x}}{2}-\frac{\mathrm{k}}{2}\)
Here c = \(\frac{k}{2}\) and m = \(-\frac{1}{2}\)
If (2) is a tangent to the parabola then c = \(\frac{a}{m}\)
⇒ \(-\frac{k}{2}=\frac{4}{-1 / 2}=-8\)
⇒ k = 16
∴ The equation of the required tangent is x + 2y + 16 = 0 ……..(3)
Point of contact = \(\left(\frac{a}{m^2}, \frac{2 a}{m}\right)\)
= \(\left(\frac{4}{(1 / 4)}, \frac{8}{-1 / 2}\right)\)
= (16, -16)

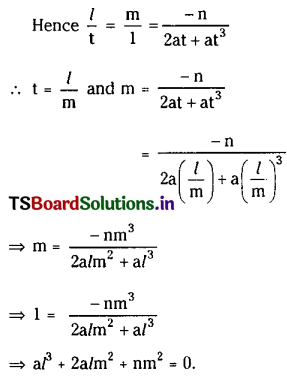
Question 2.
If lx + my + n = 0 is a normal to the parabola y² = 4ax, then show that al³ + 2alm² + nm² = 0.
Solution:
Given equation of parabola is y² = 4ax ……..(1) and given lx + my + n = 0 is a normal to the parabola y² = 4ax ……(2)
Equation of normal at a point P(t) on the parabola y² = 4ax is y + xt = 2at + at³
⇒ xt + y = 2at + at³ …….(3)
Equations (2) and (3) represent the same line.
Eliminating ‘t’ from (2) and (3) we get,

Question 3.
Show that the equation of the common tangents to the circle x² + y² = 2a² and the parabola y² = 8ax are y = ±(x + 2a). (Mar. ’10, ’09)
Solution:
Given equation of parabola is y² = 8ax …….(1) and circle is x² + y² = 2a² ……..(2)
The Centre of the circle is C = (0, 0) and the radius of the circle is r = √2a
Equation of the tangent to the parabola (1) having slope ‘m’ is y = mx + \(\frac{2a}{m}\)
⇒ my = m²x + 2a (∵ y² = 8ax is the parabola)
⇒ m²x – my + 2a = 0 ………(3)
Line (3) is also a tangent to circle (2).
∴ The perpendicular distance from C(0, 0) to line (3) is equal to radius √2a.
∴ \(\left|\frac{2 a}{\sqrt{m^4+m^2}}\right|=\sqrt{2 a}\)
⇒ \(\frac{4 a^2}{m^4+m^2}\) = 2a²
⇒ m⁴ + m² – 2 = 0
⇒ m⁴ + 2m² – m² – 2 = 0
⇒ (m² – 1) (m² + 2) = 0
⇒ m² = 1 or m² = -2
⇒ m² = 1 (∵ m² ≠ -2)
⇒ m = ±1
∴ The equations of the required tangents are y = \(\pm x+\frac{2 a}{\pm 1}\)
⇒ y = ±(x + 2a)

Question 4.
Prove that the tangents at the extremities of a focal chord of a parabola intersect at right angles on the directrix.
Solution:

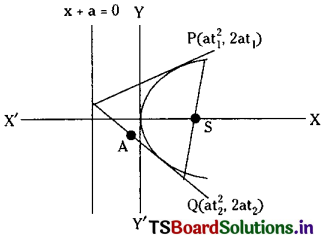
Let y² = 4ax be the equation of a parabola and
Let P\(\left(a t_1^2, 2 a t_1\right)\), Q\(\left(a t_2^2, 2 a t_2\right)\) be two extremeties of the focal chord.
Since \(\overline{\mathrm{PQ}}\) is the focal chord we have t₁t₂ = -1 (standard result)
The equation of tangent to the parabola y² = 4ax at P(t₁) is x = \(\mathrm{yt}_1+\mathrm{at}{ }_1^2\) ………(1)
Slope of the tangent (m₁) = \(\frac{1}{t_1}\)
The equation of the tangent Q(t₂) to the parabola y² = 4ax is x = \(\mathrm{yt}_2+\mathrm{at}_2^2\) ……..(2)
Slope of the tangent (m₂) = \(\frac{1}{t_2}\)
Now m₁m₂ = \(\frac{1}{t_1 t_2}\) = -1 (∵ t₁t₂ = -1)
∴ The tangents drawn at P, Q are perpendicular to each other.
The point of intersection of tangents (1) and (2) = [at₁t₂, a(t₁ + t₂)]
= [-a, a(t₁ + t₂)]
Substituting this point of intersection on the directrix of the parabola y² = 4ax i.e., x + a = 0.
We have -a + a = 0 and hence the point of intersection of tangents lie on the directrix.
∴ The tangents drawn at the extremities of the focal chord of a parabola intersect at right angles on the directrix.

Question 5.
Find the condition for the line y = mx + c to be a tangent to the parabola x² = 4ay. (Mar. ’12)
Solution:
Given equation of parabola as x² = 4ay …….(1)
and given line is mx – y + c = 0 ……..(2)
Suppose (2) is a tangent to the parabola (1).
Let P(x₁, y₁) be the point of contact.
Then the equation of the tangent to the parabola (1) at (x₁, y₁) is xx₁ – 2a(y + y₁) = 0
⇒ xx₁ – 2ay – 2ay₁ = 0 ………(3)
Since equations (2) and (3) represent the same line, the corresponding coefficients are proportional.
∴ \(\frac{x_1}{m}=\frac{-2 a}{-1}=\frac{-2 a y_1}{c}\)
⇒ x₁ = 2am, y₁ = -c
Since P(x₁, y₁) is a point on x² = 4ay we have \(\mathbf{x}_1^2\) = 4ay₁
⇒ (2am)² = 4a(-c)
⇒ am² = -c
⇒ c = -am²
∴ The condition for the line y = mx + c to be a tangent to the parabola x² = 4ay is am² + c = 0.

Question 6.
Three normals are drawn from (k, 0) to the parabola y² = 8x one of the normal is the axis and the remaining two normals are perpendicular to each other, then find the value of k.
Solution:
Given equation of parabola is y² = 8x ………(1)
Comparing with y² = 4ax we have 4a = 8
⇒ a = 2
The equation of normal to the parabola (1) having slope ‘m’ is
y = mx – 2am – am²
⇒ y = mx – 4m – 2m³
Given that the normal passes through (k, 0).
Then 0 = mk – 4m – 2m³
⇒ m(2m² + 4 – k) = 0
⇒ m = 0 or 2m² – k + 4 = 0 ……(2)
Let m₁, m₂ be the roots of a quadratic equation (2) then
m₁ + m₂ = 0, m₁m₂ = \(\frac{4-k}{2}\)
Given that the two normals are perpendicular, we have m₁m₂ = -1
⇒ \(\frac{4-k}{2}\) = -1
⇒ k = 6

Question 7.
Show that the locus of the point of intersection of the perpendicular tangents to the parabola y² = 4ax is the directrix x + a = 0.
Solution:
Given y² = 4ay ………(1) and Let P(x₁, y₁) be the point of intersection of perpendicular tangents.
Any tangent to the parabola will be of the form y = mx + \(\frac{a}{m}\) of this passes through (x₁, y₁) then y₁ = mx₁ + \(\frac{a}{m}\)
⇒ m²x₁ – my₁ + a = 0 …….(2)
Let m₁, m₂ be the slopes of tangents drawn from P(x₁, y₁) to the parabola (1) which corresponds to the roots of a quadratic equation (2).
Then m₁ + m₂ = \(\frac{y_1}{x_1}\) and m₁m₂ = \(\frac{a}{x_1}\) …….(3)
Since the tangents are perpendicular we have m₁m₂ = -1 and hence from (3),
-1 = \(\frac{a}{x_1}\)
⇒ x₁ + a = 0
∴ The Locus of (x₁, y₁) is x + a = 0 which is the equation of the directrix of the parabola (1).

Question 8.
Two parabolas have the same vertex and equal length of latus rectum such that their axes are at the right angle. Prove that the common tangents touch each at the end of the latus rectum.
Solution:

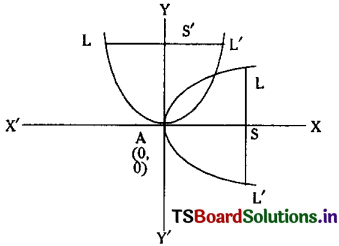
Let the equations of parabolas having the same vertex and equal length of latus rectum be
y² = 4ax …….(1)
x² = 4ay …….(2)
Let the equation of the tangent to the parabola (1) having slope ‘m’ is
y = mx + \(\frac{a}{m}\) …….(3)
But this is also a tangent to x² = 4ay
∴ c = -am²
∴ \(\frac{a}{m}\) = -am²
⇒ m³ = -1
⇒ m = 1
∴ From (3), we have y = -x – a
⇒ x + y + a = 0 ………(4)
L'(a, -2a) is the end of latus rectum of parabola (1).
∴ From (4), x + y + a = a – 2a + a = 0
∴ The common tangents to the parabolas y² = 4ax, x² = 4ay will touch the parabola at the end of the latus rectum.

Question 9.
Show that the foot of the perpendicular from focus to the tangent of parabola y² = 4ax lies on the tangent at the vertex.
Solution:
Given parabola is y² = 4ax ……….(1)
Equation of any tangent to (1) is of the form y = mx + \(\frac{a}{m}\)
⇒ my = m²x + a
⇒ m²x – my + a = 0 ……..(2)
Equation of the line perpendicular to the line (2) and passing through S(a, 0) is -m(x – a) + m²(y – 0) = 0
⇒ x + my – a = 0 ……..(3)
From (2) and (3)
(m² + 1)x = 0
⇒ x = 0
From (3), my – a = 0
⇒ y = \(\frac{a}{m}\)
∴ The point of intersection of (2) and (3) is (0, \(\frac{a}{m}\))
∴ The point (0, \(\frac{a}{m}\)) lies on the y-axis which is a tangent at the vertex.

Question 10.
Show that the tangent at one extremity of a focal chord of a parabola is parallel to the normal at the other extremity.
Solution:
Let y² = 4ax be the given parabola.
Let P = \(\left(a t_1{ }^2, 2 a t_1\right)\) and Q = \(\left(a t_2{ }^2, 2 a t_2\right)\) be the two extremities of a focal chord. then t₁t₂ = -1
The equation of the tangent to the parabola at P(t₁) is x – yt₁ + \(\mathrm{at}_1{ }^2\) = 0 …….(1)
then m₁ = \(\frac{1}{t_1}\)
The equation of the normal at Q(t₂) is xt₂ + y – 2at₂ – \(\mathrm{at}_2^3\) = 0 ……..(2)
The slope of the normal (m₂) = -t₂
= \(-\left(\frac{-1}{t_1}\right)=\frac{1}{t_1}\) (∵ t₁t₂ = -1)
∴ m₁ = m₂ and the tangent at P(t₁) is parallel to the normal at Q(t₂) to the parabola y² = 4ax.
∴ The tangent at one extremity of a focal chord is parallel to the normal at other extremities.

III.

Question 1.
The normal at a point t₁ on y² = 4ax meets the parabola again at the point t₂. Then prove that t₁t₂ + \(t_1{ }^2\) + 2 = 0.
Solution:
Given equation of parabola is y² = 4ax ……..(1)
Let P = \(\left(a t_1^2, 2 a t_1\right)\) = P(t₁) be any point on (1).
The equation of normal at P(t₁) on the parabola (1) is y + xt₁ = 2at₁ + \(\mathrm{at}_1{ }^3\)
⇒ t₁x + y = 2at₁ + \(\mathrm{at}_1{ }^3\) ……..(2)
The normal drawn at P(t₁) meets the parabola at Q(t₂).
∴ PQ is the chord of the parabola.
The equation of chord \(\overline{\mathrm{PQ}}\) of the parabola y² = 4ax is y(t₁ + t₂) = 2x + 2at₁t₂
⇒ 2x = y(t₁ + t₂) + 2at₁t₂ = 0 ……….(3)
Since equations (2) and (3) represent the same line, the coefficients of line turns are proportional.

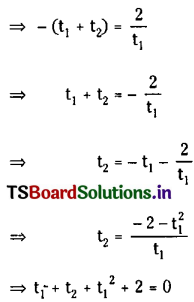
Question 2.
From an external point, P tangents are drawn to the parabola y² = 4ax and these tangents make angles θ₁, θ₂ with its axis, such that cot θ₁ + cot θ₂ is a constant ‘d’. Then show that all such P lies on a horizontal line.
Solution:

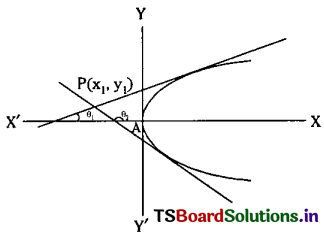
Given y² = 4ax ………(1) and Let P(x₁, y₁) be an external point.
Let the equation of a tangent to the parabola (1) having slope ‘m’ is y = mx + \(\frac{a}{m}\) …….(2)
if (2) passes through P(x₁, y₁) then y₁ = mx₁ + \(\frac{a}{m}\)
⇒ my₁ = m²x₁ + a
⇒ m²x₁ – my₁ + a = 0 …….(3)
Since this is a quadratic equation in ‘m’.
Let m₁ and m₂ be the roots. Then
m₁ + m₂ = \(\frac{y_1}{x_1}\) and m₁m₂ = \(\frac{\mathrm{a}}{\mathrm{x}_1}\)
Given that the tangents are making angles θ₁, θ₂ with its axis we have m₁ = tan θ₁ and m₂ = tan θ₂.
Also given that cot θ₁ + cot θ₂ = d

∴ The Locus of P(x₁, y₁) is y = ad which is a line parallel to X-axis.
∴ P(x₁, y₁) lies on a horizontal line.

Question 3.
Show that the common tangents to the circle 2x² + 2y² = a² and the parabola y² = 4ax intersect at the focus of the parabola y² = -4ax.
Solution:
Given equation of circle is x² + y² = \(\frac{a^2}{2}\) ……..(1)
and the given equation of parabola is y² = 4 ax ……..(2)
Let y = mx + c be a common tangent to (1) and (2).
If y = mx + c is a tangent to (1) then by the condition of tangency
c² = \(\frac{a^2}{2}\) (1 + m2) ………(3)
Also if y = mx + c is a tangent to the parabola y² = 4ax then
c = \(\frac{a}{m}\) …….(4)
∴ From (3) and (4) we have \(\frac{a^2}{m^2}=\frac{a^2}{2}\left(1+m^2\right)\)
⇒ m⁴ + m² = 2
⇒ m⁴ + m² – 2 = 0
⇒ m⁴ + 2m² – m² – 2 = 0
⇒ (m² + 2) (m² – 1) = 0
⇒ m² + 2 = 0 or m² – 1 = 0
m² + 2 = 0 is not admissible.
∴ m² – 1 = 0
⇒ m = ±1
From y = mx + c we have
= mx + \(\frac{a}{m}\)
= \(\pm x+\frac{a}{\pm 1}\)
= ± x ± a
Also y = ± x ± a satisfy the focus (-a, 0) of the parabola y² = -4ax.
Hence the common tangents to the circle (1) and parabola (2) intersect at the focus of the parabola y² = -4ax.

Question 4.
The sum of the ordinates of two points on y² = 4ax is equal to the sum of the ordinates of two other points on the same curve. Show that the chord joining the first two points is parallel to the chord joining the other two points.
Solution:
Given equation of parabola is y² = 4ax …..(1)
Let A = \(\left(\mathrm{at}_1{ }^2, 2 \mathrm{at}_1\right)\), B = \(\left(\mathrm{at}_2{ }^2, 2 \mathrm{at}_2\right)\), C = \(\left(\mathrm{at}_3{ }^2, 2 \mathrm{at}_3\right)\) and D = \(\left(\mathrm{at}_4{ }^2, 2 \mathrm{at}_4\right)\) be the four points on the parabola (1).
Given that 2at₁ + 2at₂ = 2at₃ + 2at₄
⇒ t₁ + t₂ = t₃ + t₄ ………(2)
The equation of the chord \(\overline{\mathrm{AB}}\) of the parabola y² = 4ax is y(t₁ + t₂) = 2x + 2at₁t₂
⇒ 2x – (t₁ + t₂) y + 2at₁t₂ = 0 ………(3)
Let m₁ be the slope of line (3) then m₁ = \(\frac{2}{t_1+t_2}\)
The equation of the chord \(\overline{\mathrm{CD}}\) of the parabola (1) is y(t₃ + t₄) = 2x + 2at₃t₄ and slope of this chord m₂ = \(\frac{2}{t_3+t_4}\)
∴ From (2) we have t₁ + t₂ = t₃ + t₄
Hence m₁ = m₂
∴ Chord \(\overline{\mathrm{AB}}\) is parallel to the chord \(\overline{\mathrm{CD}}\).

Question 5.
If a normal chord at a point ‘t’ on the parabola y² = 4ax subtends a right angle at the vertex then prove that t = ±√2.
Solution:
Given equation of parabola is y² = 4ax ……(1)
The equation of normal at a point P(t) on (1) is xt + y = (2at + at³) …….(2)
Let the normal at P(t) meet (1) again at Q(t₁) then PQ is the chord of the parabola (1)

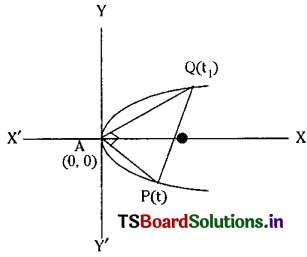
Equation of chord \(\overline{\mathrm{PQ}}\) is y(t₁ + t) – 2x – 2at₁t = 0
⇒ -2x + (t₁ + t)y – 2at₁t = 0 ………(3)
Equations (2) and (3) represent the same straight line.
∴ Coefficients of like terms are proportional