TS Inter 2nd Year Maths 2A Solutions Chapter 4 Theory of Equations Ex 4(a)

Students must practice this TS Intermediate Maths 2A Solutions Chapter 4 Theory of Equations Ex 4(a) to find a better approach to solving the problems.

TS Inter 2nd Year Maths 2A Solutions Chapter 4 Theory of Equations Ex 4(a)

I.
Question 1.
Form polynomial equations of the lowest degree, with roots as given below:
i) 1, – 1, 3
ii) 1 ± 2i, 4, 2
iii) 2 ± √3, 1 ± 2i
iv) 0, 0, 2, 2, – 2, – 2
v) 1 ± √3, 2, 5
vi) 0, 1, \(\frac{-3}{2}\), \(\frac{-5}{2}\)
Solution:
i) The polynomial equation of the lowest degree with roots as 1, – 1 and 3 is
(x – 1) (x + 1) (x – 3) = 0
⇒ (x² – 1) (x – 3) = 0
⇒ x³ – 3x² – x + 3 = 0

ii) The polynomial equation of the lowest degree with roots as 1 ± 2i, 4, 2 is
(x – (1 + 2i)) (x – (1 – 2i)) (x – 4) (x – 2) = 0
⇒ ((x – 1) – 2i) (x – 1 + 2i) (x – 4) (x – 2) = 0
⇒ ((x – 1)² + 4) (x² – 6x + 8) = 0
⇒ (x² – 2x + 1 + 4) (x² – 6x + 8)= 0
⇒ (x² – 2x + 5) (x² – 6x + 8) = 0
⇒ x⁴ – 6x³ + 8x² – 2x + 12x² – 16x + 5x² – 30 + 40 = 0
⇒ x⁴ – 8x³ + 25x² – 36x + 40 = 0.

iii)The required equation whose roots 2 ± √3, 1 ± 2i is
(x – (2 + √3)) (x – (2 – √3)) (x – (1 + 2i)) (x – (1 – 2i)) = 0
⇒ ((x – 2) – √3) (x – 2 + √3) (x – 1 – 2i) (x – 1 + 2i) = 0
⇒ [(x – 2)² – (√3)²] [(x – 1)² + 4] = 0
⇒ (x² – 4x + 4 – 3) (x² – 2x + 1 + 4) = 0
⇒ (x² – 4x + 1) (x² – 2x + 5) = 0
⇒ x⁴ – 2x³ + 5x² – 4x³ + 8x² – 20x + x² – 2x + 5 = 0
⇒ x⁴ – 6x³ + 14x² – 22x + 5 = 0.

iv) The required equation whose roots 0, 0, 2, 2, – 2, – 2 is
(x – 0) (x – 0) (x – 2) (x- 2) (x + 2) (x + 2) = 0
⇒ x² (x² – 4) (x² – 4) = 0
⇒ x² (x⁴ – 8x² + 16) = 0
⇒ x⁶ – 8x⁴ + 16x² = 0.

v) The required equation whose roots 1 ± √3, 2, 5 is
(x – (1 + √3)) (x – (1 – √3)) (x – 2) (x – 5) = 0
⇒ (x – 1 – √3) (x – 1 + √3) (x² – 7x + 10) = 0
⇒ ((x – 1)² – 3) (x² – 7x + 10) = 0
⇒ (x² – 2x – 2) (x² – 7x + 10) = 0
⇒ x⁴ – 7x³ + 10x² – 2x³ + 14x² – 20x – 2x² + 14x² – 20x – 2x² + 14x – 20 = 0
⇒ x⁴ – 9x³ + 34x² – 26x – 20 = 0.

vi) The required equation whose roots 0, 1, \(\frac{-3}{2}\), \(\frac{-5}{2}\)
(x – 0) (x – 1) (x + \(\frac{3}{2}\)) (x + \(\frac{5}{2}\)) = 0
⇒ (x² – x) (x² + \(\frac{5 x}{2}+\frac{5 x}{2}+\frac{15}{4}\)) = 0
⇒ (x² – x) (x² + 4x + \(\frac{15}{4}\)) = 0
⇒ x⁴ + 3x³ + \(\frac{15 x^2}{4}\) – x³ – 4x² – \(\frac{15 x{4}\) = 0
⇒ x⁴ + 3x³ – \(\frac{x^2}{4}-\frac{15 x}{4}\) = 0
⇒ 4x⁴ + 12x³ – x² – 15x = 0.

Question 2.
If α, β, γ are the roots of 4x³ – 6x² + 7x + 3 = 0, then find the value of a + 3y + ya.
Solution:
Given, α, β and γ are the roots of 4x³ – 6x² + 7x + 3 = 0
∴ αβ + βγ + γα = \(\frac{-\mathrm{p}_1}{\mathrm{p}_0}\)
= \(\frac{-(-6)}{4}=\frac{3}{2}\).

Question 3.
If 1, 1, α are the roots of x³ – 6x² + 9x – 4= 0, then find α.
Solution:
Given, 1, 1, α are the roots of x³ – 6x² + 9x – 4 = 0
∴ Sum of roots = \(\frac{-\mathrm{p}_1}{\mathrm{p}_0}\)
⇒ 1 + 1 + α = – (- 6)
⇒ 2 + α = 6
α = 4.

Question 4.
If – 1, 2 and are the roots of 2x³ + x² – 7x – 6 = 0, then find α.
Solution:
Given – 1, 2 and α are roots of
2x³ + x² – 7x – 6 = 0
∴ – 1 + 2 + α = \(\frac{-1}{2}\)
α = \(\frac{-1}{2}\) – 1 = \(\frac{-3}{2}\).

Question 5.
If 1, – 2 and 3 are the roots of x³ – 2x² + ax + 6 = 0, then find a.
Solution:
Given, 1, – 2 and 3 are the roots of x³ – 2x² + ax + 6 = 0
∴ αβ + βγ + γα = 1
⇒ 1 . (- 2) + (- 2) (3)i + 3(1) = a
⇒ a = – 2 – 6 + 3 = – 5.

Question 6.
If the product of the roots of 4x³ + 16x² – 9x – a = 0 is 9, then find a.
Solution:
Given equation is 4x³ + 16x² – 9x – a = 0
Given product of roots of above equation is 9.
⇒ – (- a) = 9 [∴ αβγ = \(\frac{-\mathrm{p}_3}{\mathrm{p}_0}\)]
⇒ a = 9.

Question 7.
Find s₁, s₂, s₃ and s₄ for each of the following equations.
i) x₄ – 16x₃ + 86x₂ – 176x + 105 = 0
ii) 8x₄ – 2x₃ – 27x₂ + 6x + 9 = 0
[Hint: s₁ = \(\sum_{\mathbf{1}=1}^4\) α_i, s₂ = \(\sum_{1 \leq 1i α_j, s₃ = [latex]\sum_{1 \leq 1<ji α_j α_k, s₄ = α₁ α₂ α₃ α₄].
Solution:
i) Given equation is
x⁴ – 16x³ + 86x² – 176x + 105 = 0 ……………..(1)
Compare (1) with
p₀x⁴ + p₁x³ + p₂x² + p₃x + p₄ = o
∴ p₀ = 1, p₁ = – 16, p₂ = 86, p₃ = 176, p₄ = 105.
∴ S₁ = Sum of roots
= [latex]\frac{-p_1}{p_0}=\frac{-(-16)}{1}\) = 16
S₂ = Sum of product of roots taken two at a time
= \(\frac{-\mathrm{p}_2}{\mathrm{p}_0}=\frac{86}{1}\) = 86
S₃ = Sum of product of roots taken three at a time = \(\frac{-p_3}{p_0}=\frac{-(-176)}{1}\) = 176
S4 = Product of four roots
= \(\frac{\mathrm{p}_4}{\mathrm{p}_0}=\frac{105}{1}\) = 105.

ii) Given equation is
8x⁴ – 2x³ – 27x² + 6x + 9 = 0 ……………… (1)
[Hint: same as above]
∴ S₁ = \(\frac{-(-2)}{8}=\frac{1}{4}\);
S₂ = \(\frac{-27}{8}\);
S₃ = \(\frac{-6}{8}=\frac{-3}{4}\);
S₄ = \(\frac{9}{8}\).

II.
Question 1.
If α, β and 1 are the roots of x³ – 2x² – 5x + 6 = 0, then find α and β.
Solution:
Given, α, β and 1 are the roots of x³ – 2x² – 5x + 6 = 0
∴ S₁ = \(\frac{-\mathrm{p}_1}{\mathrm{p}_0}\)
∴ α + β + 1 = – (- 2)
⇒ α + β = 1 ……………..(1)
S₃ = \(\frac{-\mathrm{p}_3}{\mathrm{p}_0}\)
αβ (1) = \(\frac{-6}{1}\)
αβ = – 6 ……………(2)
We know that
α – β = ± \(\sqrt{(\alpha+\beta)^2-4 \alpha \beta}\)
= ± \(\sqrt{1+24}\) = ± 5.

Case-(i):
If α – β = 5 …………(3) then
(1) + (3) ⇒ 2α = 6
α = 3.
From (1), β = – 2
∴ α = 3, β = – 2.

Case (ii):
If α – β = – 5 …………..(4) then
(1) + (5) ⇒ 2α = – 4
α = – 2
From (1), β = 3
∴ α = 3, β = – 2.

Question 2.
If α, β and γ are the roots of x³ – 2x² + 3x – 4 = 0 then find
i) Σ α²β²
ii) Σ αβ (α + β)
Solution:
Given, α, β and γ are the roots of
x³ – 2x² + 3x – 4 = 0 …………(1)
∴ α + β + γ = 2, αβ + βγ + γα = 3;
αβγ = 4 ………….(2)
i) ∴ Σ α²β² = α²β² + β²γ² + γ²α²
= (αβ + βγ + γα)² – 2αβγ (α + β + γ)
= 3² – 2 (4) (2) = 9 – 16 = – 7.

ii) Σ αβ (α + β)
= Σ α²β + Σ αβ²
= α²β + α²γ + β²α + β²γ + γ²α + γ²β
= (α + β + γ) (αβ + βγ + γα) – 3αβγ
= 2 (3) – 3 (4) = – 6.

Question 3.
If α, β and γ are the roots of x³ + px² + qx + r = 0, then find
i) Σ \(\frac{1}{\alpha^2 \beta^2}\)
ii) \(\frac{\beta^2+\gamma^2}{\beta \gamma}+\frac{\gamma^2+\alpha^2}{\gamma \alpha}+\frac{\alpha^2+\beta^2}{\alpha \beta}\)
iii)(β + γ – 3α) (γ + α – 3β) (α + β – 3γ)
iv) Σ α³β³
Solution:
Given α, β and γ are the roots of
x³ + px² + qx + r = 0
∴ α + β + γ = – p;
αβ + βγ + γα = q;
αβγ = – r

i) Σ \(\frac{1}{\alpha^2 \beta^2}\)

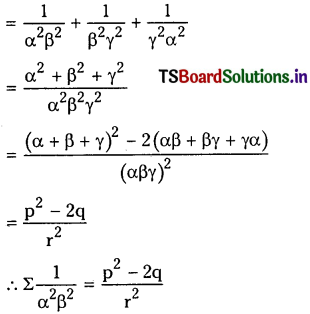
ii) \(\frac{\beta^2+\gamma^2}{\beta \gamma}+\frac{\gamma^2+\alpha^2}{\gamma \alpha}+\frac{\alpha^2+\beta^2}{\alpha \beta}\)

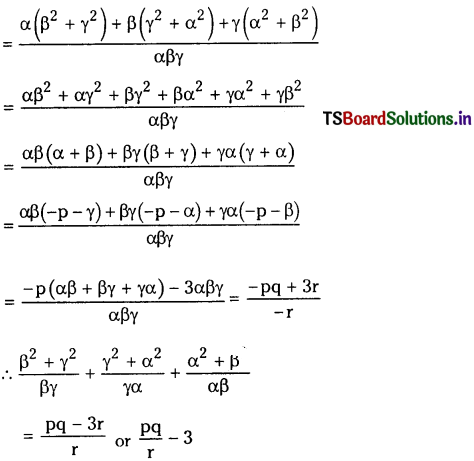
iii) (β + γ – 3α) (γ + α – 3β) (α + β – 3γ)
= (α + β + γ – 4α) (α + β + γ – 4β) (α + β + γ – 4γ)
= (- p – 4α) (- p – 4β) (- p – 4γ)
= – (p + 4α) (p + 4β) (p + 4γ)
= – [p³ + (4α + 4β + 4γ) p² + (16αβ + 16βγ + 16γα)p + 64 αβγ
= – [p³ + (α + β + γ)4p² + (αβ + βγ + γα) 16p + 64 αβγ]
= – [p³ – 4p³ + 16pq – 64r]
= 3p³ – 16pq + 64r.

iv) Σ α³β³ = α³β³ + β³γ³ + γ³α³
We know that
(αβ + βγ + γα)² = α²β² + β²γ² + γ²α² + 2αβγ (α + β + γ)
⇒ q² = α²β² + β²γ² + γ²α² + 2pr
⇒ α²β² + β²γ² + γ²α² = q² – 2pr ………….(1)
Consider
Σ α²β = α²β + β²α + γ²α + α²γ + γ²β + β²γ
= (αβ + βγ + γα) (α + β + γ) – 3 αβγ
⇒ Σ α²β = – pq + 3r ……………….(2)
Now, α³β³ + β³γ³ + γ³α³ = (α²β² + β²γ² + γ²α²) (αβ + βγ + γα) – αβγ Σ α²β
= (q² – 2pr) q + r (- pq + 3r)
(∵ from (1) and (2))
∴ α³β³ + β³γ³ + γ³α³ = q³ – 3pqr + 3r²

III.
Question 1.
If α, β, γ are the roots of x³ – 6x² + 11x – 6 = 0, then find the equation whose roots are α² + β², β² + γ², γ² + α².
Solution:
Let α, β, γ be the roots of the equation
x³ – 6x³ + 11x – 6 = 0 …………… (1)
∴ α + β + γ = 6; αβ + βγ + γα = 11
Let y = α² + β²
⇒ y = α² + β² + γ² – γ²
⇒ y = (α + β + γ)γ² – 2(αβ + βγ + γα) – γ²
⇒ y = 36 – 2(11) – γ²
⇒ y = 14 – γ²
⇒ γ = \(\sqrt{14-y}\)
∴ ‘γ’ is a root of equation (1),
we have γ³ – 6γ² + 11γ – 6 = 0.
⇒ \((\sqrt{14-y})^3-6(\sqrt{14-y})^2+11(\sqrt{14-y})\) – 6 = 0
⇒ \(\sqrt{14-y}\) (25 – y) = 6 (15 – y)
⇒ \(\sqrt{14-y}\) (25 – y)² = 36 (15 – y)²
⇒ \(\sqrt{14-y}\) (625 + y² – 50y) = 36 (225 + y² – 50y)
8750 + 64y² – 1325 y² – y³ = 36y² – 1080y + 8100
⇒ y³ – 28y² + 245y – 650 = 0
which represents a cubic equation with roots
α² + β², β² + γ² and γ² + α².
∴ Required equation is x³ – 28x² + 245x – 650 = 0.

Question 2.
If α, β, γ are the roots of x³ – 7x + 6 = 0, then find the equation whose roots are (α – β)², (β – γ)², (γ – α)².
Solution:
Given α, β, γy are the roots of equation
x³ – 7x + 6 = 0 ………….(1)
α + β + γ = 0; αβ + βγ + γα = – 7; αβγ = – 6.
Let y = (α – β)2 = (α + β)² – 4αβ
⇒ y = γ² – 4 \(\left(\frac{-6}{\gamma}\right)\)
⇒ y = γ² + \(\frac{24}{\gamma}\)
⇒ yγ = γ³ + 24
⇒ yγ = 7γ – 6 + 24 (∵ γ is a root of (1))
⇒ y(γ – 7) = 18
⇒ γ = \(\frac{18}{y-7}\)
Substituting in (1), we get
\(\left(\frac{18}{y-7}\right)^3-7\left(\frac{8}{y-7}\right)\) + 6 = 0
⇒ 18³ – 126 (y – 7)² + 6 (y – 7)³ = 0
⇒ 5832 – 126 (y² – 14y + 49) + 6 (y³ – 21y² + 147y – 343) = 0
⇒ y³ – 42y³ + 441y – 400 = 0
which represents cubic equation, with roots (α – β)², (β – γ)², (γ – α)².
∴ Required equation is x³ – 42x² + 441x – 400 = 0.

Question 3.
If α, β, γ are the roots of the equation x³ – 3ax + b = 0, then prove that Σ (α – β) (α – γ) = 9a.
Solution:
Given α, β, γ are the roots of x³ – 3ax + b = 0.
∴ α + β + γ = 0; αβ + βγ + γα = – 3a; αβγ = – b.
Now Σ (α – β) (α – γ))
= Σ [α² – αβ – αγ + βγ]
= (α + β + γ) – (αβ + βγ + γα)
= (α + β + γ)² – 3(αβ + βγ + γα)
= 0 – 3 (- 3a) = 9a
∴ Σ(α – β) (α – γ) = 9a.