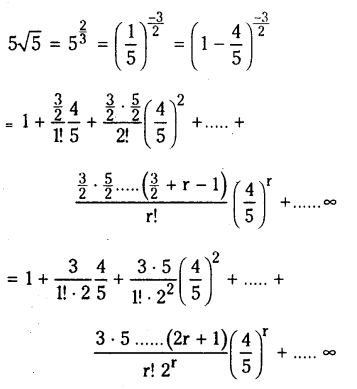TS Inter 2nd Year Maths 2A Binomial Theorem Important Questions

Students must practice these TS Inter 2nd Year Maths 2A Important Questions Chapter 6 Binomial Theorem to help strengthen their preparations for exams.

TS Inter 2nd Year Maths 2A Binomial Theorem Important Questions

Question 1.
Find the largest binomial coefficient(s) in the expansion of i) (1+x)¹⁹ (ii) (1 +x)²⁴
Solution:
i) Here n = 19, an odd integer. Therefore, by corollary 6.1.19. the largest binomial coefficients are \({ }^{\mathrm{n}} \mathrm{C}_{\left(\frac{\mathrm{n}-1}{2}\right)}\) and

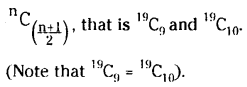
ii) Here n 24 ¡s an even integer. Hence there is only one largest binomial coefficient, that is \({ }^n C_{\left(\frac{n}{2}\right)}={ }^{24} C_{12}\)

Question 2.
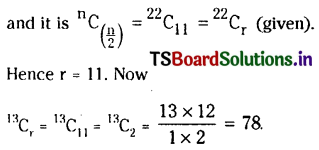
If ²²C_r is the largest bínomial coefficient in the expansion of (1+ x)²² find the value of ¹³C_r
Solution:
Here n = 22 is an even integer. Therefore, there is only one largest binomial coefficient

Question 3.
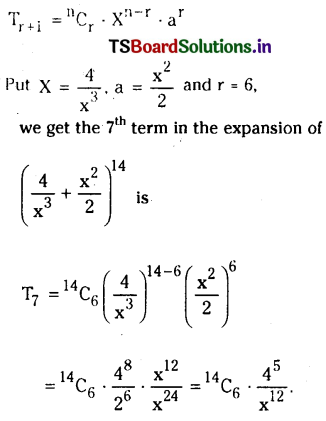
Find the 7th term in the expansion of
\(\left(\frac{4}{x^3}+\frac{x^2}{2}\right)^{14}\)
Solution:
The general term in the expansion of (X + a)ⁿ is given by

Question 4.
Find the 3rd term from the end in the expension of  \(\left(x^{\frac{-2}{3}}-\frac{3}{x^2}\right)^8\)
Solution:
Comparing the given expansion with (x + a), we get
\(X=x^{\frac{-2}{3}}, a=\frac{-3}{x^2}, n=8\)
The expansion has (n + 1) = 9 terms.
Hence the 3 term from the end is 7th term from the beginning and

Question 5.
Find the coefficients of x⁹ and x¹⁰ in the expansion of  \(\left(2 x^2-\frac{1}{x}\right)^{20}\)
Solution:

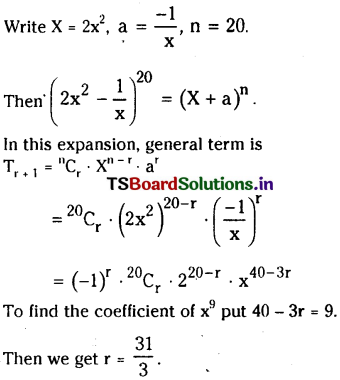
To find the coefficient of x⁹ put 40 – 3r = 9.
Then we get r = \(\frac{31}{3}\)
Since r is a positive integer this is not possible. This means that the expansion of \(\left(2 x^2-\frac{1}{x}\right)^{20}\) doesn’t posess x⁹ term. This means that the coefficient of x⁹ in the expansion of \(\left(2 x^2-\frac{1}{x}\right)^{20}\)  is 0.
To find the coefficient of x¹⁰ put 40 –  3r = 10.
We get r = 10
Now, on substituting r 10 in (1), we get that
\(T_{11}=(-1)^{10} \cdot{ }^{20} \mathrm{C}_{10} \cdot 2^{10} \cdot x^{10}\)
Hence, the coefficient of x¹⁰ in the expansion of \(\left(2 x^2-\frac{1}{x}\right)^{20} \text { is }{ }^{20} \mathrm{C}_{10} \cdot 2^{10}\)

Question 6.
Find the term independent of x (that is the constant term) in the expansion of \(\left(\sqrt{\frac{x}{3}}+\frac{3}{2 x^2}\right)^{10}\)
Solution:

Question 7.
If the coefficient of x¹⁰ in the expansion \(\left(a x^2+\frac{1}{b x}\right)^{11}\) is equal to the coefficient of
x^-10 In the expansion of \(\left(a x-\frac{1}{b x^2}\right)^{11}\) find the ration between a and b where a and b are real numbers.
Solution:
Step – 1: The general term in the expansion

To find the coefficient of x¹⁰ in this expansion, we should consider 22 – 3r = 10 or r = 4. Hence, the coefficient of x¹⁰ in the expansion of

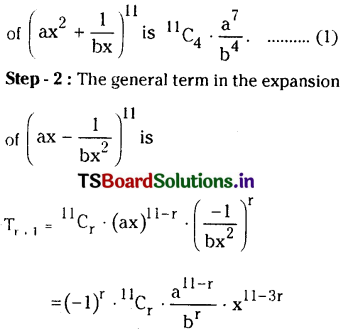
To find the coefficient of x^-10 in this expansion
we should consider 11 – 3r = – 10 or r = 7.
Thus the coefficient of x^-10 in the expansion

Question 8.
If the k^th term is the middle term in the expansion of \(\left(x^2-\frac{1}{2 x}\right)^{20}\), find T_k and T_k+3
Solution:
The general term in the expansion of \(\left(x^2-\frac{1}{2 x}\right)^{20}\) is given by

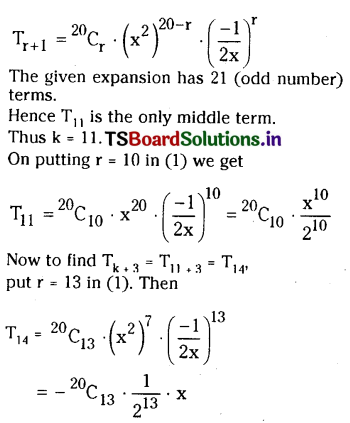
Question 9.
If the coefficients of (2r + 4)^th and (r – 2)^nd terms in the expansion of (1 + x)¹⁸ are equal, find r.
Solution:
The r^th term in the given expansion of
\((1+x)^{18} \text { is } \mathrm{T}_{\mathrm{r}}={ }^{18} \mathrm{C}_{(\mathrm{r}-1)} \cdot \mathrm{x}^{\mathrm{r}-1}\)
Thus, the coefficient of \({ }^{18} C_{r-1}\)
Given that the coefficient of (2r + 4)^th term = the coefficient of (r -2)^nd term.
That is \({ }^{18} C_{2 r+3}={ }^{18} C_{r-3}\)
⇒ 2r+ 3r – 3 or (2r + 3) +(r-3) 18
⇒ r = – 6 or r 6
Since r is a positive integer, we get r z 6

Question 10.
Prove that 2.C₀ +7.C₁ + 12C₂ + …. + (5n + Z)C_n (5n + 4)2^n-1
Solution:
First method:
The coefficieint of C₀, C₁, C₂, ………………. C_n in LH.S.
are 2, 7, 12 , (5n + 2) which are in A.P. with first term a = 2 and common difference d=5
Hence from example 6.1.14 (1), we get that
2C₀ + 7C₁ + 12C₂ + (5n+2).C_n
=(2a + nd) . 2^n-1
= (4 + 5n) 2^n-1

Second method:
The general term ((r +1)^th term) in LH.S (5r + 2) C_r Therefore,

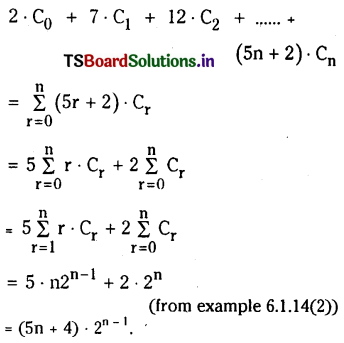
Question 11.
Prove that

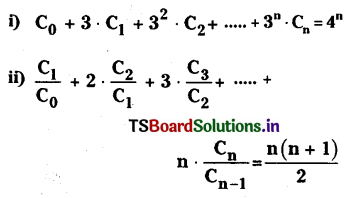
Solution:

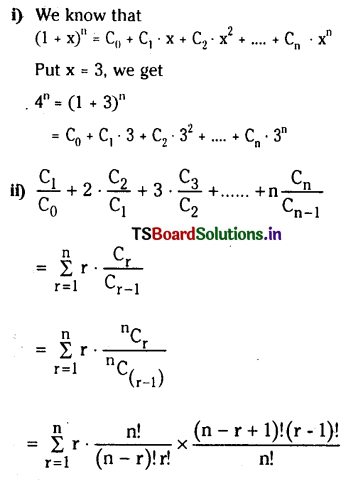
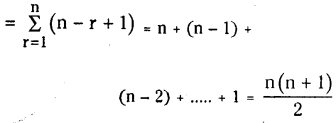
Question 12.
For r = 0, 1, 2 ……………….. n, prove that

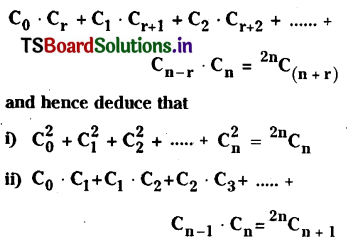
Solution:

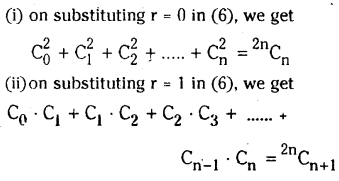
Question 13.
Prove that

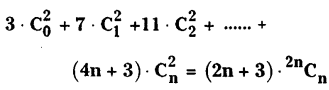
Solution:

Question 14.
Find the numerically greatest term(s) in the expansion of
(i) (2+3x)¹⁰ when x= \(\frac{11}{8}\)
(ii) (3 x-4y)¹⁴ when x=8, y=3
Solution:




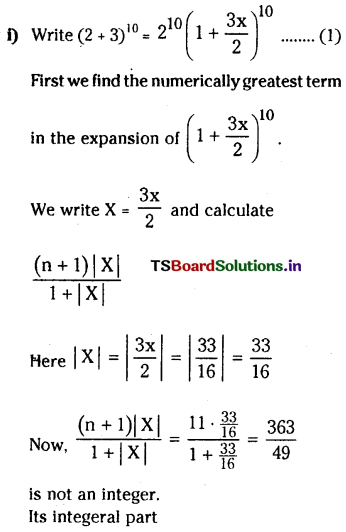
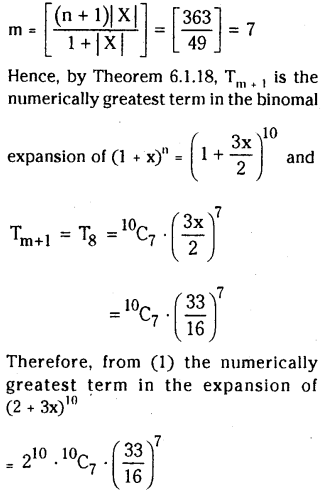
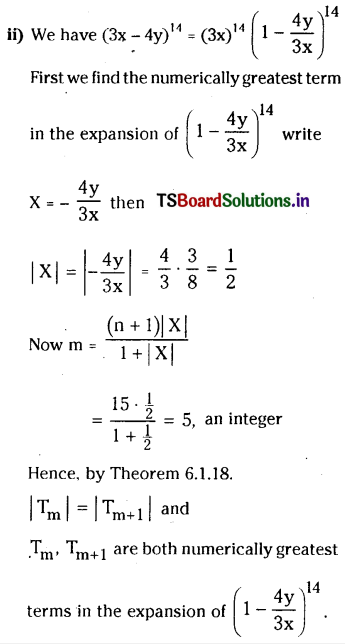
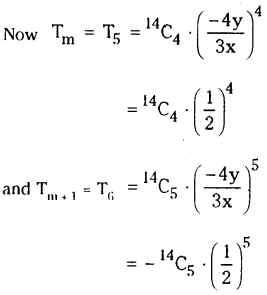
Therefore the numerically greatest terms in the expansion of (3x – 4y)¹⁴ are T₅ and T₆. They are

Question 15.
Prove that 6²ⁿ – 35n -1 is divisible by 1225 for all natural numbers n.
Solution:

i.e., 6²ⁿ-35ⁿ – 1 = 1225 (k) for sorne integer k(if n≥2)
If n = 1, then 6²ⁿ-35ⁿ – 1 = 6²-35-1 = 0, which is trivially divisible by 1225. Hence, for all natural numbers n, 6²I – 35n – 1 is divisible by 1225.
Note: The above problem can also be proved by induction.

Question 16.
Suppose that n is a natural number and I, F are respectively the Integral part and fractional part of \((7+4 \sqrt{3})^n\). Then show that
(i) I Is an odd integer
(ii) (1 + F)(1 – F) = 1.
Solution:

2k where k is a positive integer ………………. (1)
Thus, I + F + f is an even integer.
Since I is an integer, we get that
F + f is an integer. Also, since 0 < F < 1 and 0 < f < 1.
we get 0 < F + f < 2.
Since F + f is an integer, we get
F + f = 1 i.e., 1 – F = f

(i) From (1), I + F+ f= 2k
⇒ 1+ 1 = 2k ⇒ 1= 2k – I, an odd integer.

(ii) (1 +F) (1-F) (1 + F) f from (2)
= \((7+4 \sqrt{3})^n(7-4 \sqrt{3})^n=(49-48)^n=1\)

Question 17.
Find the coefficient of x⁶ in (3 + 2x + x²)⁶
Solution:

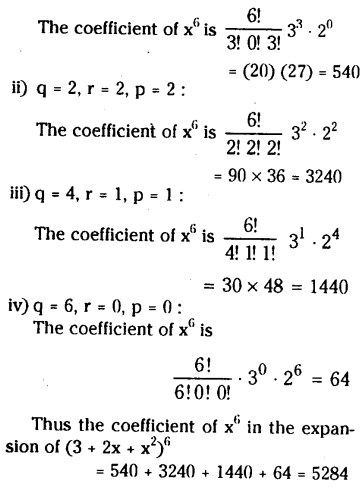
Question 18.
If n is a positive integer, then prove that
\(C_0+\frac{C_1}{2}+\frac{C_2}{3}+\ldots+\frac{C_n}{n+1}=\frac{2^{n+1}-1}{n+1}\)
Solution:

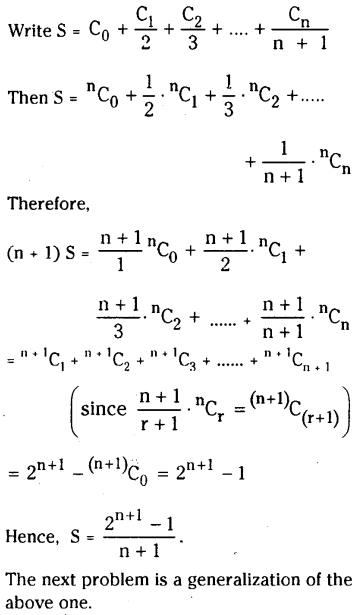
Question 19.
If n is a positive Integer and x is any non zero real number, then prove that

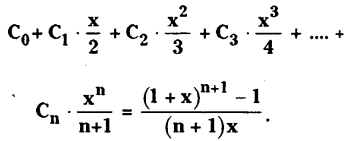
Solution:

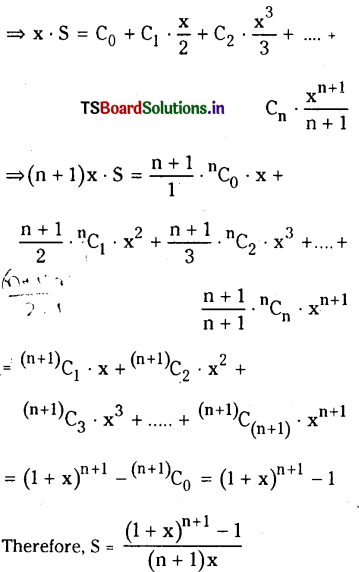
Question 20.
Prove that

Solution:

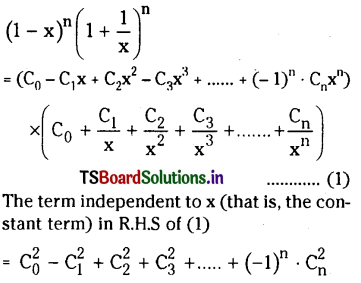
Now, we calculate the term independent of x in the L.H.S of equation (1). From (1)

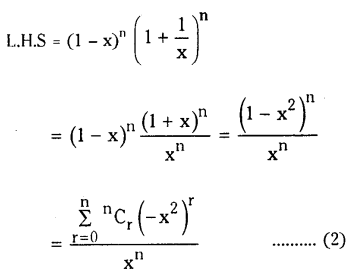
Observe that the expansion in the numerator of (2) contains only even powers of x. Therefore, if n is odd, then there is no constant term in (2). In other words, the term independent of x in \((1-x)^n\left(1+\frac{1}{x}\right)^n\) is zero. Now, suppose n is an even integer say n=2 k. Then, from (2) we get

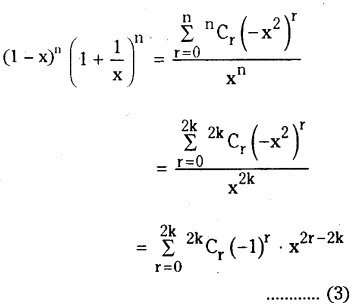
To get the term independent of x in (3), put 2 r-2 k=0. Then r=k and hence the term independent of x in
\((1-x)^n\left(1+\frac{1}{x}\right)^n\) is
\({ }^{2 \mathrm{k}} C_k \cdot(-1)^{\mathrm{k}}={ }^n C_{\frac{n}{2}}(-1)^{\frac{\mathrm{n}}{2}}\)

Question 21.
Find the set E of the values of x for which the binomial expansions for the following are valid.
(i) \((3-4 x)^{\frac{3}{4}}\)
(ii) \((2+5 x)^{\frac{-1}{2}}\)
(iii) (7 – 4x)^-5
(iv) \((4+9 x)^{\frac{-2}{3}}\)
(v) (a+bx)
Solution:

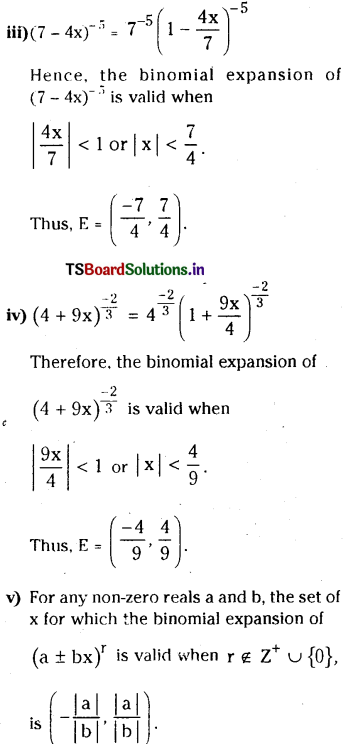
Question 22.
Find the


Solution:

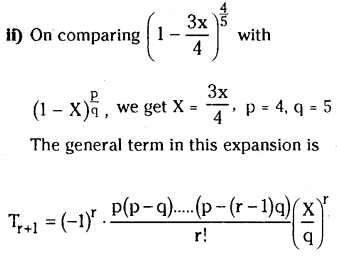
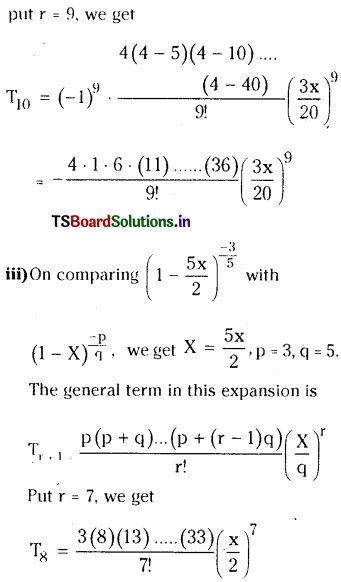
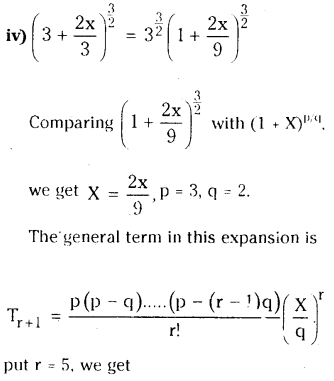
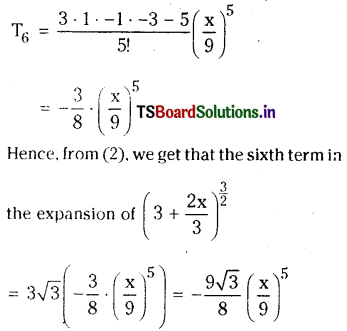
Question 23.
Write the first 3 terms in the expansion of
(i) \(\left(1+\frac{x}{2}\right)^{-5}\)
(ii) \((3+4 x)^{\frac{-2}{3}}\)
(iii) \((4-5 x)^{\frac{-1}{2}}\)
Solution:

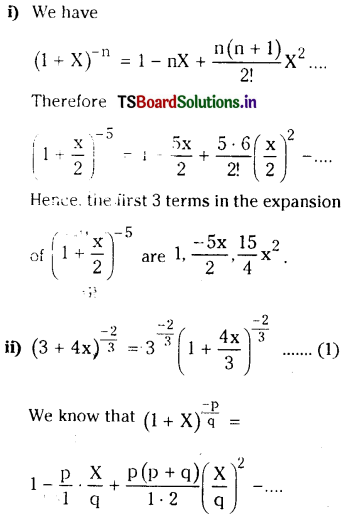
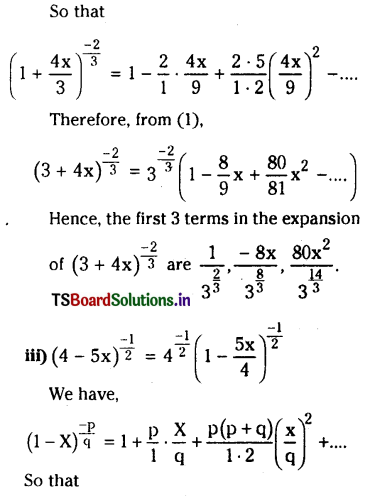
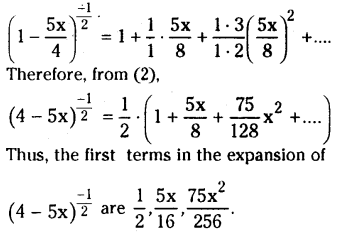
Question 24.
Write the general term in the expansion of
(i) \(\left(3+\frac{x}{2}\right)^{\frac{-1}{3}}\)
(ii) \(\left(2+\frac{3 x}{4}\right)^{\frac{4}{5}}\)
(iii) (1 – 4x)^-3
(iv) \((2-3 x)^{\frac{-1}{3}}\)
Solution:

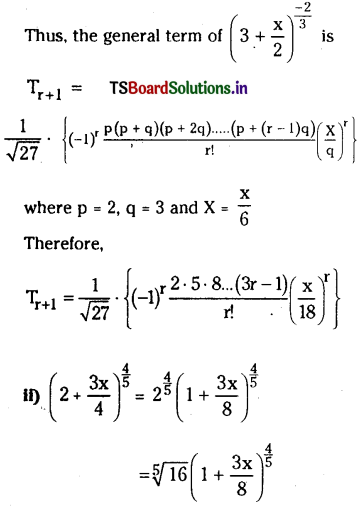
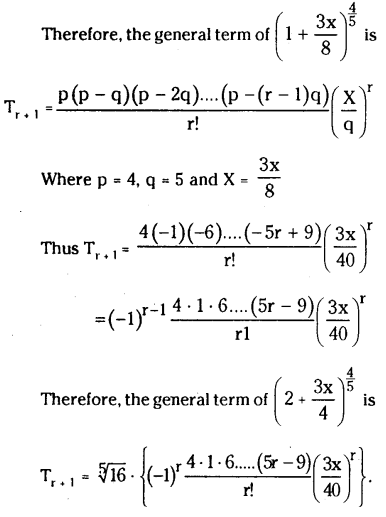
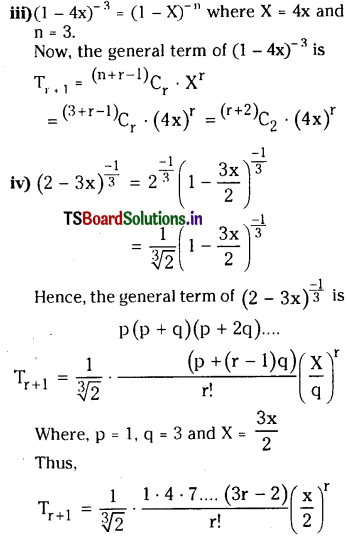
Question 25.
Find the coefficient of x¹² in \(\frac{(1+3 x)}{(1-4 x)^4}\)
Solution:

Question 26.
Find the coefficient of x⁶ in the expansion of \((1-3x)^{\frac{-2}{5}}\)
Solution:

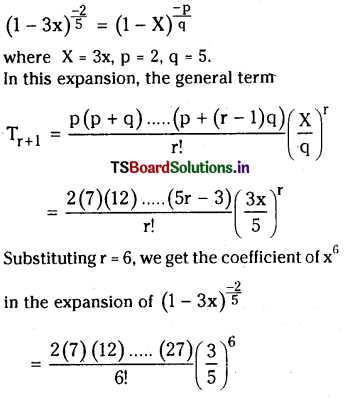
Question 27.
Find the sum of the infinite series
\(1+\frac{2}{3} \cdot \frac{1}{2}+\frac{2 \cdot 5}{3 \cdot 6}\left(\frac{1}{2}\right)^2+\frac{2 \cdot 5 \cdot 8}{3 \cdot 6 \cdot 9}\left(\frac{1}{2}\right)^3+\ldots \infty\)
Solution:

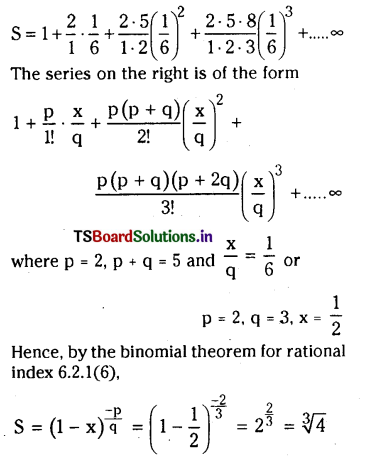
Question 28.
Find the sum of the series
\(\frac{3 \cdot 5}{5 \cdot 10}+\frac{3 \cdot 5 \cdot 7}{5 \cdot 10 \cdot 15}+\frac{3 \cdot 5 \cdot 7 \cdot 9}{5 \cdot 10 \cdot 15 \cdot 20}+\ldots ……….. \infty\)
Solution:

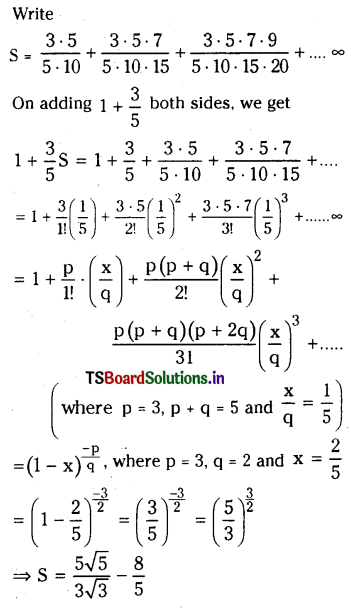
Question 29.
If x = \(\frac{1}{5}+\frac{1 \cdot 3}{5 \cdot 10}+\frac{1 \cdot 3 \cdot 5}{5 \cdot 10 \cdot 15}+\ldots \ldots \infty\)
Solution:

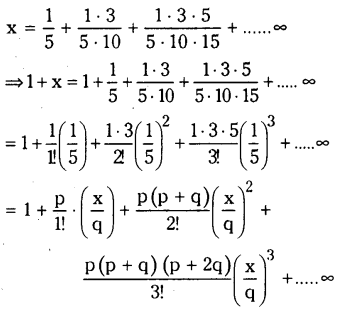
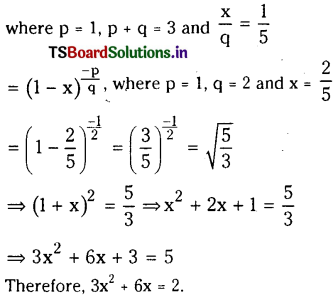
Question 30.
Find an approximate value of \(\sqrt[6]{63}\) correct to 4 decimal places.
Solution:

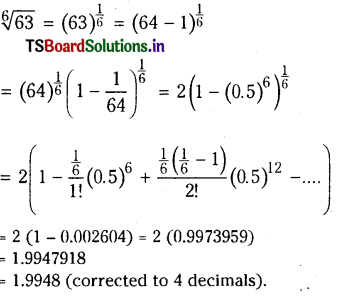
Question 31.
If \(|\mathbf{x}|\) is so small that x² and higher powers of x may be neglected, then find an approximate value of \(\frac{\left(1+\frac{3 x}{2}\right)^{-4}(8+9 x)^{\frac{1}{3}}}{(1+2 x)^2}\)
Solution:

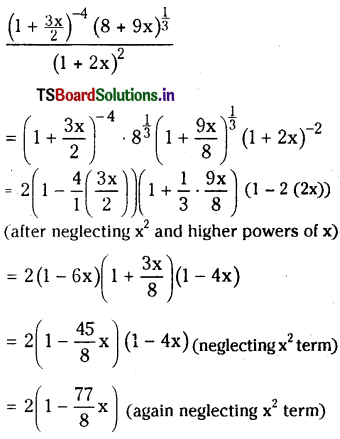
Question 32.
If |x| is so small that x⁴ and higher powers of x may be neglected, then find an approximate value of
\(\sqrt[4]{x^2+81}-\sqrt[4]{x^2+16}\)
Solution:

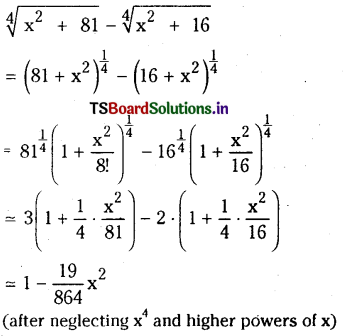
Question 33.
Suppose that x and y are positive and x is very small when compared to y. Then find an approximate value of
\(\left(\frac{y}{y+x}\right)^{\frac{3}{4}}-\left(\frac{y}{y+x}\right)^{\frac{4}{5}}\)
Solution:

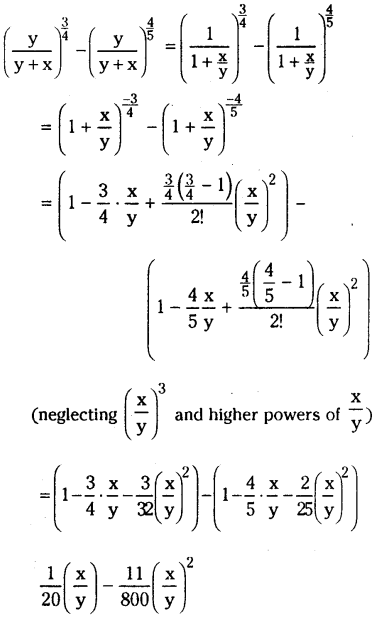
Question 34
Expand \(5 \sqrt{5}\) increasing powers of \(\frac{4}{5}\)
Solution: