TS Inter 1st Year Maths 1A Solutions Chapter 6 Trigonometric Ratios upto Transformations Ex 6(a)

Students must practice these TS Inter 1st Year Maths 1A Study Material Chapter 6 Trigonometric Ratios upto Transformations Ex 6(a) to find a better approach to solving the problems.

TS Inter 1st Year Maths 1A Trigonometric Ratios upto Transformations Solutions Exercise 6(a)

I.
Question 1.
Convert the following into simplest form
(i) tan (θ – 14π)
Answer:
tan (θ – 14π) = tan [- (14π – θ)]
= – tan (14π – θ)
= – tan [ 2(7π) – θ)
= – tan (-θ) = tan θ

(ii) cot (\(\frac{21 \pi}{2}\) – θ)
Answer:
cot (\(\frac{21 \pi}{2}\) – θ) = cot[10π + (\(\frac{\pi}{2}\) – θ)]
= cot (\(\frac{\pi}{2}\) – θ) = tan θ

(iii) cosec (5π + θ)
Answer:
cosec (5π + θ) = cosec [4π + (π + θ)]
= cosec(π + θ) = – cosec θ

(iv) sec (4π – θ)
Answer:
sec (4π – θ) = sec [2(2π) – θ]
= sec (- θ) = sec θ

Question 2.
Find the values of each of the following
(i) sin (-405°)
Answer:
sin (-405°) = -sin 405° = -sin (360°+45°)
= – sin 45° = \(-\frac{1}{\sqrt{2}}\)

(ii) cos \(\left(-\frac{7 \pi}{2}\right)\)
Answer:
cos \(\left(-\frac{7 \pi}{2}\right)\) = cos \(\frac{7 \pi}{2}\) = cos (630°)
= cos (360 + 270°) = cos 270°
= cos (180 + 90) = -cos 90 = 0
(or) cos \(\left(-\frac{7 \pi}{2}\right)\) = 0 (∵ cos(2n + 1)\(\frac{\pi}{2}\) = 0)

(iii) sec (2100°)
Sol. sec (2100°) = sec [5 × 360° + 300°]
= sec 300° = sec (360° – 60°)
= sec 60° = 2

(iv) cot (-315°)
Answer:
cot (-315°) = – cot 315° = – cot (270 + 45°)
= cot 45° = 1

Question 3.
Evaluate
(i) cos² 45° + cos² 135° + cos² 225° + cos² 315°
Answer:
cos 45° = \(\frac{1}{\sqrt{2}}\), cos 135° = cos (180 – 45°)
= – cos 45° = \(-\frac{1}{\sqrt{2}}\)

cos 225° = cos (180 + 45°)
= – cos 45° = –\(\frac{1}{\sqrt{2}}\)

cos 315° = cos(360 – 45°)
= cos 45° = \(\frac{1}{\sqrt{2}}\)

∴ cos² 45° + cos² 135° + cos² 225° + cos² 315°
= \(\frac{1}{2}+\frac{1}{2}+\frac{1}{2}+\frac{1}{2}\) = 2

(ii) sin²\(\frac{2 \pi}{3}\) + cos²\(\frac{5 \pi}{6}\) – tan²\(\frac{3 \pi}{4}\)
Answer:

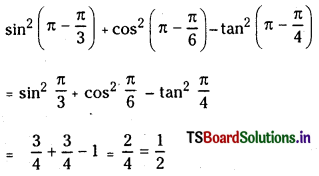
(iii) cos 225° – sin 225° + tan 495° – cot 495°
Answer:
cot (180 + 45) – sIn (180 + 45) + tan (360 + 135) – cot (360 + 135)
= – cot 45° + sin 45° + tan 135 – cot 135°
= – cos 45° + sin 45° +tan(180 – 45) – cot(180 – 45)
= – cos 45° + sin 45° – tan 45° + cot 45°
= \(-\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}\) – 1 + 1 = 0

(iv) (cos θ – sin θ) if
(a) θ = \(\frac{7 \pi}{4}\)
(b) θ = \(\frac{11 \pi}{4}\)
Answer:

Question 4.
(i) If sin θ = –\(\frac{1}{3}\) and 0 does not lie in the third 3 quadrant, find the values of (a) cos θ and (b) cot θ. (March 2013)
Answer:
sin θ = –\(\frac{1}{3}\) and sin θ is negative and does not lie in third quadrant,
⇒ θ lies in fourth quadrant. In IVth quadrant cos θ is positive and cot θ is negative.
a) cos θ = \(\sqrt{1-\sin ^2 \theta}=\sqrt{1-\frac{1}{9}}=\frac{2 \sqrt{2}}{3}\)
b) cot θ = \(\frac{\cos \theta}{\sin \theta}\) = -2√2

(ii) If cos θ = t (0 < t < 1) and θ does not lie in the first quadrant, find the values of a) sin θ b) tan θ
Answer:
cos θ = t, (0 < t < 1)
⇒ cos θ is positive and 0 does not lie in first quadrant
⇒ θ lies in IVth quadrant
a) sin θ = \(-\sqrt{1-\cos ^2 \theta}=-\sqrt{1-t^2}\)
b) tan θ = \(\frac{\sin \theta}{\cos \theta}=-\frac{\sqrt{1-t^2}}{t}\)

(iii) Find the value of sin 330°. cos 120° + cos 210°. sin 300°
Answer:
sin 330° cos 120° + cos 210° sin 300°
= sin (360 – 30) cos (180 – 60) + cos ( 180 + 30) sin (360 – 60)
= (-sin 30°) (-cos 60°) + (-cos 30°) (- sin 60°)
= sin 30 cos 60 + cos 30 sin 60 = sin (30 + 60)
= sin 90° = 1

(iv) If cosec θ + cot θ = \(\frac{1}{3}\), find cos θ and determine the quadrant in which θ lies.
Answer:
we have coses²θ – cot² θ = 1
⇒ (cosec θ + cot θ) (cosec θ – cot θ) = 1

∴ sin θ is positive and cos θ is negative,
⇒ θ lies in IInd quadrant.

Question 5.
(i) If sin α + cosec α= 2, find the value of sinⁿ α + cosecⁿ α; n ∈ Z.
Answer:
Given sin α + cosec α = 2
Squaring both sides
sin² α = cosec² α + 2 = 4
⇒ sin α + cosec α = 2
cubing on both sides
sin³ α + cosec³ α + 3 sin α cosec α (sin α + cosec α) = 8
sin³ α + cosec³ α + 3 (2) = 8
⇒ sin³ α + cosec³ α = 2
In the same way sinⁿ α + cosecⁿ α = 2 (n ∈ z)

(ii) If sec θ + tan θ = 5, find the quadrant in which θ lies and find the value of sin θ
Answer:
We have sec² θ – tan² θ = 1
⇒ (sec θ + tan θ) (sec θ – tan θ) = 1
Also given sec θ + tan θ = 5 ………….(2)

Adding (1) and (2)

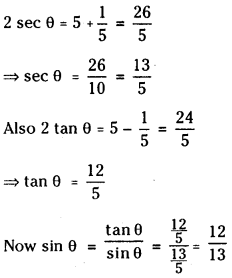
tan θ is +ve, sec θ is + ve
⇒ θ lies is first quadrant.

II.
Question 1.
Prove that
(i) \(\frac{\cos (\pi-A) \cot \left(\frac{\pi}{2}+A\right) \cos (-A)}{\tan (\pi+A) \tan \left(\frac{3 \pi}{2}+A\right) \sin (2 \pi-A)}\) = cos A
Answer:

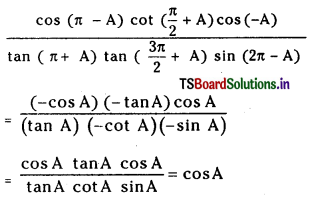
(ii) \(\frac{\sin (3 \pi-A) \cos \left(A-\frac{\pi}{2}\right) \tan \left(\frac{3 \pi}{2}-A\right)}{{cosec}\left(\frac{13 \pi}{2}+A\right) \sec (3 \pi+A) \cot \left(A-\frac{\pi}{2}\right)}\)
Answer:

(iii) sin 780°. sin 480° + cos 240°. cos 300° = \(\frac{1}{2}\)
Answer:
sin [2 × 360 + 60] sin [360 + 120] + cos [180 + 60] cos [360-60]
= sin 60 sin 120 – cos 60 cos 60
= sin 60 sin 60 – cos 60. cos 60
= \(\frac{\sqrt{3}}{2} \frac{\sqrt{3}}{2}-\frac{1}{2} \cdot \frac{1}{2}=\frac{3}{4}-\frac{1}{4}=\frac{1}{2}\)

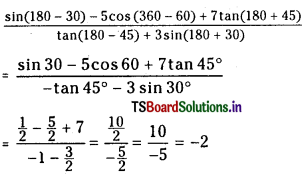
(iv) \(\frac{\sin 150^{\circ}-5 \cos 300^{\circ}+7 \tan 225^{\circ}}{\tan 135^{\circ}+3 \sin 210^{\circ}}\) = -2
Answer:

(v) cot\(\left(\frac{\pi}{20}\right)\). cot\(\left(\frac{3 \pi}{20}\right)\). cot\(\left(\frac{5 \pi}{20}\right)\). cot\(\left(\frac{7 \pi}{20}\right)\). cot\(\left(\frac{9 \pi}{20}\right)\) = 1
Answer:
cot\(\left(\frac{\pi}{20}\right)\). cot\(\left(\frac{3 \pi}{20}\right)\). cot\(\left(\frac{5 \pi}{20}\right)\). cot\(\left(\frac{7 \pi}{20}\right)\). cot\(\left(\frac{9 \pi}{20}\right)\)
= cot 9°. cot 27°. cot 45°. cot 63°. cot 81°
= cot 9°. cot 27°. 1.cot (90 – 27) . cot (90 -9)
= cot 9°. cot 27°. 1. tan 27°. tan 9°
= 1

Question 2.
(i) Simplify \(\frac{\sin \left(-\frac{11 \pi}{3}\right) \tan \left(\frac{35 \pi}{6}\right) \sec \left(-\frac{7 \pi}{3}\right)}{\cot \left(\frac{5 \pi}{4}\right) {cosec}\left(\frac{7 \pi}{4}\right) \cos \left(\frac{17 \pi}{6}\right)}\)
Answer:

(ii) If tan 20 ° = p, prove that
\(\frac{\tan 610^{\circ}+\tan 700^{\circ}}{\tan 560^{\circ}-\tan 470^{\circ}}=\frac{1-p^2}{1+p^2}\)
Answer:
Given that tan 20° = p then

(iii) If α, β are complementary angles such that b sin α = a, then find the value of (sin α cos β – cos α sin β)
Answer:
Given α, β are complementary angles α + β = 90°
⇒ β = 90° – α
∴ sin α cos β – cos α sin β
= sin (α – β)
= sin[α – (90 – α)]
= sin [2α – 90°]
= -sin[90 – 2α]
= -cos 2α
= -(1 – 2sin²α) = -1 + 2sin²α
= -1 + 2\(\left(\frac{a^2}{b^2}\right)\)
= \(\frac{2 a^2-b^2}{b^2}\)

Question 3.
(i) If cos A = cos B = – \(\frac{1}{2}\), A does not lie in the second quadrant and B does not lie in third quadrant, then find the value of \(\frac{4 \sin B-3 \tan A}{\tan B+\sin A}\)
Answer:
cos A = –\(\frac{1}{2}\) and A does not lie in second quadrant
⇒ A lies in third quadrant
cos B = –\(\frac{1}{2}\) and B does not lie in third quadrant
⇒ B lies in second quadrant
cos A = –\(\frac{1}{2}\) and A lie in third quadrant
⇒ A = 240°
cos B = –\(\frac{1}{2}\) and B lies in second quadrant.
⇒ B = 120°

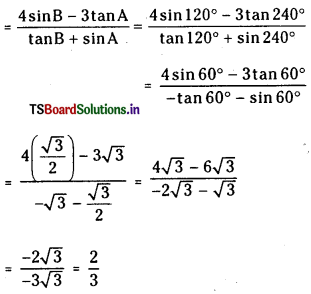
(ii) If 8 tan A = -15 and 25 sin B = -7 and neither A nor B is in the fourth quadrant, then show that sin A cos B + cos A sin B = \(\frac{-304}{425}\)
Answer:
8 tan A = -15 25 sin B = -7
⇒ tan A = \(\frac{-15}{8}\) ⇒ sin B = \(\frac{-7}{25}\)
Given neither A nor B is in the fourth quadrant, clearly A is in second quadrant and B is in third quadrant,
sin A cos B + cos A sin B

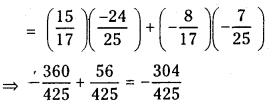
(iii) If A, B, C, D are angle of a cyclic quadrilateral then prove that
a) sin A – sin C = sin D – sin B
b) cos A + cos B + cos C + cos D = 0
Answer:
A, B, C, D are angles of a cyclic quadrilateral
⇒ A + C = 180°, and B + D = 180° ……………(1)
C = 180 – A and D = 180° – B
a) LHS = sin A – sin C
= Sin A – sin (180 – A) = 0
RHS = sin D – sin B
= sin (180 – B) – sin B
= sin B – sin B = 0
LHS = RHS

b) LHS cos A + cos B + cos C + cos D
= cos A + cos B + cos (180 – A) + cos (180 – B)
= cos A + cos B – cos A – cos B = 0
= RHS

Question 4.
If a cos θ – b sin θ = c, then show that a sin θ + b sin θ = ±\(\sqrt{a^2+b^2-c^2}\)
Answer:
Given a cos θ – b sin θ = c
and let a sin θ + b cos θ = x
squaring and adding, we get
⇒ (a cos θ – b sin θ)² + (a sin θ + b cos θ)²
= c² + x²
a² (cos² θ + sin² θ)² + b² (sin² θ + cos² θ)
= c² + x²
⇒ a² + b² + c² + x² ⇒ x² = a² + b² + c²
⇒ x = ±\(\sqrt{a^2+b^2-c^2}\)

(ii) If 3 sin A + 5 cos A = 5, then show that 5 sin A – 3 cos A = ± 3
Answer:
Given that 3 sin A + 5 cos A = 5
Let 5 sin A – 3 cos A = x
squaring and adding, we get
(3 sin A + 5 cos A)² + (5 sin A – 3 cos A)²
= 5² + x²
⇒ 9 (sin² A + cos² A) + 25 (cos² A + sin² A)
⇒ 34 = 25 + x² ± ⇒ x² = 9 ⇒ x = ±3
∴ 5 sin A – 3 cos A = ± 3

(iii) If tan² θ = (1 – e²), show that sec θ + tan³ θ. cosec θ = (2 – e²)^3/2.
Answer:
Given tan²θ = 1 – e²
⇒ sec² θ= 1 + tan² θ = 1
LHS = sec θ + tan³ θ. cosec θ
= sec θ + \(\frac{\sin ^3 \theta}{\cos ^3 \theta} \cdot \frac{1}{\sin \theta}\)
= sec θ + tan² θ sec θ = ( 1 + tan² θ) sec θ
= sec² θ sec θ
= (2 – e²) \(\sqrt{2-\mathrm{e}^2}\)
= (2 – e²)^3/2 = RHS

III.
Question 1.
Prove that following
(i) \(\frac{\tan \theta+\sec \theta-1}{\tan \theta-\sec \theta+1}=\frac{1+\sin \theta}{\cos \theta}\)
Answer:

(ii) prove that
(1 + cot θ – cosec θ) (1 + tan θ + sec θ) = 2
Answer:
(1 + cot θ – cosec θ) (1 + tan θ + sec θ)

(iii) 3 (sin θ – cos θ)⁴ + 6 (sin θ + cos θ)² + 4 (sin⁶ θ + cos⁶ θ) = 13
Answer:
(sin θ – cos θ)² = sin² θ + cos² θ – 2 sin θ cos θ
= 1 – 2sin θ cos θ (sin θ – cos θ)⁴
= (1 – 2 sin θ cos θ)²
= 1 – 4 sin θ cos θ + 4 sin² θ cos² θ (sin θ + cos θ)²
= sin² θ + cos² θ + 2 sin θ cos θ
= 1 + 2 sin θ cos θ
sin⁶ θ + cos⁶ θ = (sin² θ)³ + (cos² θ)³
= (sin² θ + cos² θ) ( sin⁴ θ + cos⁴ θ + sin² θ cos² θ)
= 1 [(sin²θ + cos²θ)² – 2 sin²θ cos²θ + sin²θ cos²θ]
= [1 – sin² θ cos² θ]
LHS = 3 (sin θ – cos θ)⁴ + 6 (sin θ + cos θ)² + 4(sin⁶θ + cos⁶θ)
= 3 [ 1 – 4 sin θ cos θ + 4 sin² θ cos² θ] + 6 [1 + 2sin θ cos θ] + 4 [ 1 – sin² θ cos² θ]
= 3 + 6 + 4 = 13 = RHS

Question 2.
(i) Prove that (sin θ + cosec θ)² + (cos θ + sec θ)² – (tan² θ + cot² θ) = 7
Answer:
(sin θ + cosec θ)² + (cos θ + sec θ)² – (tan²θ + cot²θ)
= sin² θ + 2 + cosec² θ + cos² θ + 2 + sec² θ – tan² θ cot² θ
= sin² θ + 2 + 1 + cot² θ + cos² θ+ 2 + 1 + tan² θ – tan² θ – cot² θ
= (sin² θ + cos² θ) + 2 + 1 + 2 + 1
= 1 + 2 + 1 + 2 + 1 = 7

(ii) cos⁴α + 2 cos²α(1 – \(\frac{1}{\sec ^2 \alpha}\)) = 1 – sin²α
Answer:
cos⁴α + 2 cos²α(1 – cos²α)
= cos⁴α + 2 cos²α sin²α
= cos²α[cos²α + 2sin²α]
= (1 – sin²α)[cos²α + sin²α + sin²α]
= (1 – sin²α)(1 + sin²α) = 1 – sin⁴α

(iii) \(\frac{(1+\sin \theta-\cos \theta)^2}{(1+\sin \theta+\cos \theta)^2}=\frac{1-\cos \theta}{1+\cos \theta}\)
Answer:

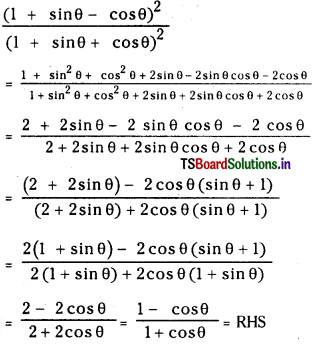
(iv) If \(\frac{2 \sin \theta}{(1+\cos \theta+\sin \theta)}\) = x, then find the value of \(\frac{1-\cos \theta+\sin \theta}{1+\sin \theta}\)
Answer:

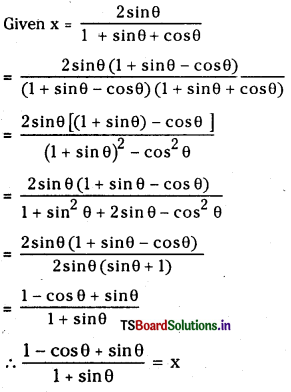
Question 3.
Eliminate θ from the following
(i) x = a cos³ θ, y = b sin³ θ
Answer:

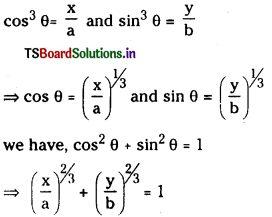
(ii) x = a cos⁴ θ, y = b sin⁴ θ
Answer:

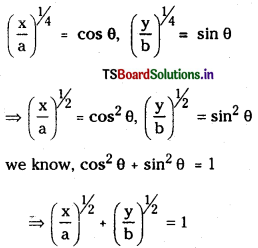
(iii) x = a (sec θ + tan θ), y = b(sec θ – tan θ)
Answer:
\(\frac{x}{a}\) = sec θ + tan θ, \(\frac{y}{b}\) = sec θ – tan θ
⇒ \(\left(\frac{x}{a}\right)\left(\frac{y}{b}\right)\) = (sec θ + tan θ)(sec θ – tan θ)
= sec²θ – tan²θ = 1
⇒ xy = ab

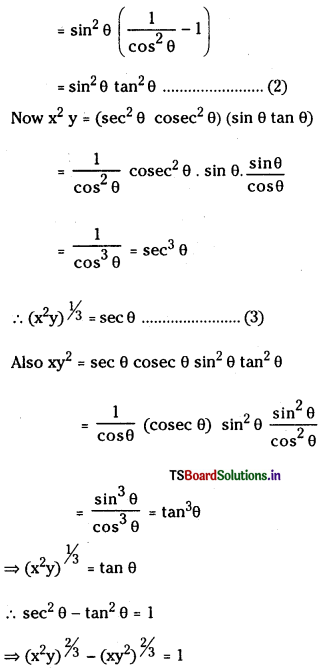
(iv) x = cot θ + tan θ, y = sec θ – cos θ
Answer:
x = cot θ + tan θ
⇒ x² = (cot θ + tan θ)²
= cot²θ + tan²θ + 2
= (1 + cot²θ) + (1 + tan²θ)
= cosec²θ + sec²θ
= \(\frac{1}{\sin ^2 \theta}+\frac{1}{\cos ^2 \theta}\)
= \(\frac{\sin ^2 \theta+\cos ^2 \theta}{\sin ^2 \theta \cos ^2 \theta}=\frac{1}{\sin ^2 \theta \cos ^2 \theta}\)
∴ x² = sec²θ cosec²θ …………..(1)
y = sec θ – cos θ
⇒ y² = sec² θ + cos² θ – 2
= -1 + sec² θ – 1 + cos² θ
= tan² θ – sin² θ