TS 10th Class Maths Solutions Chapter 7 Coordinate Geometry InText Questions

Students can practice TS Class 10 Maths Solutions Chapter 7 Coordinate Geometry InText Questions to get the best methods of solving problems.

TS 10th Class Maths Solutions Chapter 7 Coordinate Geometry InText Questions

Do This

Question 1.
i) From the figure write co-ordinates of the points A, B, C, D, E, F, G, H. (Page No. 159)

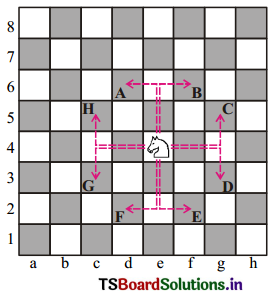
Solution:
Given : knight is at the origin (0, 0)
i.e., 36 + 25 + y² – 10y = 16 + 9 + y² – 6y
4y = 36
y = 9
So, the required point is (0, 9).
let us check our solution:
AP = \(\sqrt{(6-0)^2+(5-9)^2}\)
BP = \(\sqrt{(-4-0)^2+(3-9)^2}\)
= \(\sqrt{36+16}\) = \(\sqrt{52}\)
= \(\sqrt{16+36}\) = \(\sqrt{52}\)
So, (0, 9) is equidistant from (6, 5) and (-4, 3)

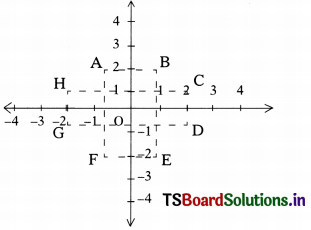
Therefore A(- 1, 2), B(l, 2), C(2, 1), D(2, -1), E(1, -2), F(-1, -2), G(-2, -1), H(-2, 1).

ii) Find the distance covered by the knight in each of its 8 moves i.e., find the distance of A, B, C, D, E, F, G, H and from the origin. (Page No. 159)
Solution:
Origin (0, 0)
Points A, B, C, D, E, E G, H
Distance of any point P(x, y) from the origin is

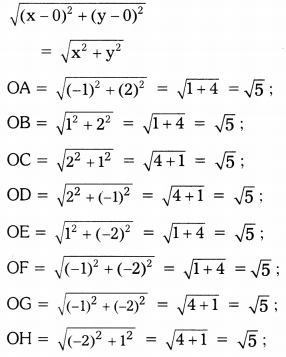
iii) What is the distance between two points H and C ? And also find the distance between two points A and B. (Page No. 159)
Solution:
Given : H(-2, 1), C(2, 1), A(-1, 2), B(1, 2)
Distance between any two points
P(x₁, y₁) Q(x₂, y₂) is
\(\overline{\mathrm{PQ}}\) = \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)
∴ Distance between H and C is
HC = \(\sqrt{[2-(-2)]^2+[1-1]^2}\)
= \(\sqrt{4^2+0}\) = \(\sqrt{16}\) = 4 units.
Distance between A and B is
AB = \(\sqrt{[1-(-1)]^2+(2-2)^2}\)
= \(\sqrt{2^2+0^2}\)
= \(\sqrt{4+0}\) = 2 units.

Question 2.
Where do these following points lie (-4, 0) (2, 0) (6, 0) (-8, 0) on co-ordinate plane ? (Page No. 160)
Solution:
Given points are (-4, 0), (2, 0), (6, 0), (-8, 0) these all points have their y-co-ordinate = 0
∴ These points lie on X – axis.

Question 3.
What is the distance between points (-4, 0) and (6, 0) on co-ordinate plane ? (Page No. 160)
Solution:
Given points = (- 4, 0) (6, 0).
These two points lie on the X – axis.
∴ Distance between them = | x₂ – x₁ |
= | 16 – (-4)| = |6 + 4| = 10

Question 4.
Find the distance between the following points.

i) (3, 8) (6, 8). (Page No. 162)
Solution:
Given points = A(3, 8), B(6, 8)
These two points lie on X – axis Distance between A(3, 8) & B(6, 8)
= | x₂ – x₁|
= |6 – 3| = 3 units.

ii) (-4, – 3) (-8,-3)
Solution:
Given points A(- 4, -3) & B(-8, -3)
These two points lie on X – axis
∴ Distance between
A(-4, -3) and B(-8, -3) = |x₂ – x₁|
= |-8 – (-4)|
= |-8 + 4|
= 4 units.

iii) (3, 4) (3, 8)
Solution:
Given points A(3, 4) & B(3, 8)
These two points lie on Y-axis
∴ Distance between A(3, 4) & B(3, 8) = |(y₂ – y₁)|
= | 8 – 4|
= 4 units.

iv) (-5, -8) (-5, -12)
Solution:
Given points A(-5, -8), B(-5, -12)
These two points lie on Y – axis
Distance between
A(-5, -8) & B(-5, -12) = | y₂ – y₁|
= |-12 + 8|
= 4 units.

Question 5.
Find the distance between the following points. (Page No. 162)

i) A = (2, 0) and B (0, 4)
Solution:
Given points = A(2, 0), B(0, 4)

ii) P(0, 5) and Q(12, 0)
Solution:
Given points P(0, 5), Q(12, 0)
PQ = \(\sqrt{(12-0)^2+(0-5)^2}\)
= \(\sqrt{144+25}\)
= \(\sqrt{169}\) = 13 units.

Question 6.
Find the distance between the following pair of points. (Page No. 164)
i) (7, 8) and (-2, 3)
Solution:
Given points = (7, 8) & (-2, 3)
distance formula

ii) (-8, 6) and (2, 0)
Solution:
Given points = (-8, 6) and (2, 0)

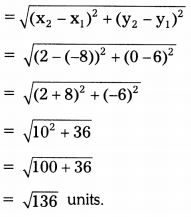
Try This

Question 1.
Where do these following points lie (0, -3), (0, -8), (0, 6), (0, 4) ? (Page No. 161)
Solution:
As the X-co-ordinate of all these points is zero, all points lie on Y-axis.

Question 2.
What is the distance between (0, -3), (0, -8) and justify that the distance between two points on Y- axis is | y₂ – y₁ | on Co-ordinate plane ? (Page No. 161)
Solution:
As the given two points lie on Y-axis, distance between them is | y₂ – y₁ | = | -3 + 8| = 5 units.
Let (0, y₁) and (0, y₂) be any two points on Y-axis, then distance between them
= \(\sqrt{(0-0)^2+\left(\mathrm{y}_2-\mathrm{y}_1\right)^2}\)
= \(\sqrt{0+\left(\mathrm{y}_2-\mathrm{y}_1\right)^2}\)
= \(\sqrt{\left(\mathrm{y}_2-\mathrm{y}_1\right)^2}\)
= |y₂ – y₁|
[∵ distance can’t be negative].

Question 3.
Find the distance between points “O (ori-gin)” and A(7, 4). (Page No. 162)
Solution:
Given : Origin and a point (7, 4)
Distance of a point (x, y) from the origin is = \(\sqrt{x^2+y^2}\)
= \(\sqrt{7^2+4^2}\) = \(\sqrt{49+16}\) = \(\sqrt{65}\) units.

Question 4.
Find the distance between A(1, -3), B(-4, 4) and rounded to two decimal. (Page No. 164)
Solution:
Given: A(1, -3), B(-4, 4)
AB = \(\sqrt{\left(\mathrm{x}_2-\mathrm{x}_1\right)^2+\left(\mathrm{y}_2-\mathrm{y}_1\right)^2}\)
= \(\sqrt{(-4-1)^2+(4+3)^2}\)
= \(\sqrt{25+49}\)
= \(\sqrt{74}\)
= 8.602 = 8.60

Think – Discuss

Question 1.
How will you find the distance between two points in which x or y co-ordinates are same but not zero ? (Page No. 161)
Solution:
Let the points be A(2, 3), B(2, 5)
Here the x-co-ordinates are same, then the dis-tance between the points, A and B is | y₂ – y₁ | = |5 – 3| = 2 units.
It the points P(4, 3), Q(-8, 3), Here the y-co- ordinates are same. In such a case, the distance is given by |x₂ – x₁| = |-8 – 4| = |-12| = 12 units.
i.e.,

Question 2.
Ramu says the distance of a point P(x₁, y₁) from the origin O(0, 0) is \(\sqrt{\mathbf{x}_1^2+\mathbf{y}_1^2}\). Do you agree with Ramu or not ? Why ? (Page No. 163)
Solution:
Yes, The distance between 0(0,0) and P(x₁, y₁) is \(\sqrt{\left(x_1-0\right)^2+\left(y_1-0\right)^2}\) ⇒ \(\sqrt{\mathrm{x}_1^2+\mathrm{y}_1^2}\)

Question 3.
Ramu also writes the distance formula as AB = \(\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2}\) why ? (Page No. 163)
Solution:
(x₁ – x₂)² is same as (x₂ – x₁)² and (y₁ – y₂)² is same as (y₂ – y₁)² i.e.,
= \(\sqrt{\left(\mathrm{x}_2-\mathrm{x}_1\right)^2+\left(\mathrm{y}_2-\mathrm{y}_1\right)^2}\)
= \(\sqrt{\left(\mathrm{x}_1-\mathrm{x}_2\right)^2+\left(\mathrm{y}_1-\mathrm{y}_2\right)^2}\)

Question 4.
Sridhar calculated the distance between T(5, 2) and R(-4, -1) to the nearest decimal is 9.5 units.
Now you find the distance between P(4, 1) and Q(-5, -2). Do you get the same answer that Sridhar got ? Why ? (Page No. 164)
Solution:
Distance between T(5, 2) and R(-4, -1) to the nearest decimal = 9.5 units.
(According to Sridhar’s calculation)
We find the distance between P(4, 1) and (-5, -2)
= \(\sqrt{(4+5)^2+(1+2)^2}\)
= \(\sqrt{(9)^2+(3)^2}\) = \(\sqrt{81+9}\)
= \(\sqrt{90}\) = 9.48 = 9.5
because the points P(4, 1), Q(-5, 2), R(-4, -1) and T(5, 2) are almost have same signs.

Do This

Question 1.
Find the point which divides the line seg¬ment joining the points (3, 5) and (8, 10) internally in the ratio 2 : 3. (Page No. 171)
Solution:
Let P(x, y) be the required point then

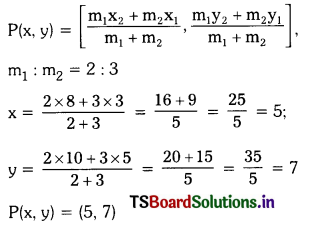
Question 2.
Find the midpoint of the line segment joining the points (2, 7) and (12, -7). (Page No. 171)
Solution:
Midpoint of the line joining the points (x₁, y₁), (x₂, y₂) is

Question 3.
Find the trisectional points of line joining (2, 6) and (-4, 8). (Page No. 172)
Solution:
A(2, 6), B(-4, 8) be the given points.
Let P Q divide the line joining of \(\overline{\mathrm{AB}}\) in the ratio 1: 2 & 2: 1
section formula (x, y)
= \(\left[\frac{\mathrm{m}_1 \mathrm{x}_2+\mathrm{m}_2 \mathrm{x}_1}{\mathrm{~m}_1+\mathrm{m}_2}, \frac{\mathrm{m}_1 \mathrm{y}_2+\mathrm{m}_2 \mathrm{y}_1}{\mathrm{~m}_1+\mathrm{m}_2}\right]\)
P(x, y) in the ratio 1 : 2

for Q(x, y) in the ratio 2 : 1

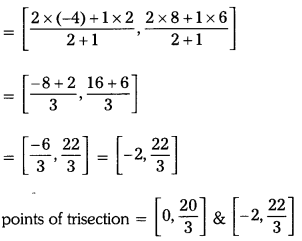
Question 4.
Find the trisectional points of line joining (-3, -5) and (-6, -8). (Page No. 172)
Solution:
Given : A(-3, -5) B(-6, -8). Let P, Q be the points of trisection of \(\overline{\mathrm{AB}}\), then P divides \(\overline{\mathrm{AB}}\) in the ratio 1: 2

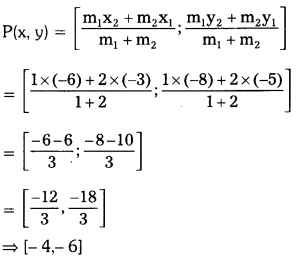
Q(x, y) divides AB in the ratio 2: 1
Q(x, y)

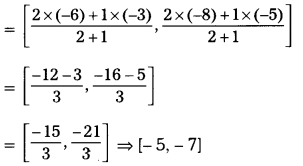
∴ The points of trisection are P(-4, -6), Q(-5, -7)

Question 5.
Find the centrold of the triangle whose vertices are (-4, 6), (2, -2) and (2, 5) respectively. (Page No. 174)
Solution:
Given points: (-4, 6), (2, -2), (2, 5)
The co-ordinates of the centroid

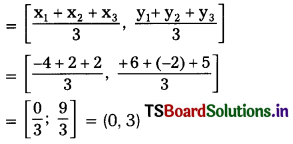
∴ The centroid is (0, 3)

Try This

Let A (4, 2) B (6, 5) C (1, 4) be the vertices of Δ ABC.

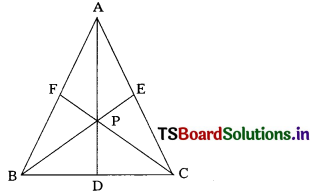
Question 1.
The median from A meets BC at D. Find the co-ordinates of the point D. (Page No. 173)
Solution:
D is the mid point of BC

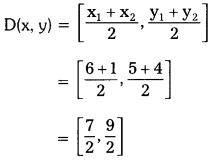
Question 2.
Find the co-ordinates of the point P on AD such that AP : PD = 2: 1 (Page No. 173)
Solution:
P is a point on AD which divides AD in the ratio 2 : 1
∴ P(x, y) = \(\left[\frac{m_1 x_2+m_2 x_1}{m_1+m_2}, \frac{m_1 y_2+m_2 y_1}{m_1+m_2}\right]\)

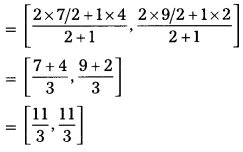
Question 3.
Find the co-ordinates of points E and F on medians BE and CF. (Page No. 173)
Solution:
E is the mid point of \(\overline{\mathrm{AC}}\)

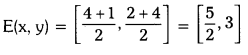
similarly, F is the mid point of \(\overline{\mathrm{AB}}\)

Question 4.
Find the points which divide the line segment BE in the ratio 2: 1 and also that divide the line segment CF in the ratio
2: 1. (Page No. 173)
Solution:
Given: B(6, 5), E[\(\frac{5}{2}\), 3], ratio 2: 1
Let it be P(x, y) =

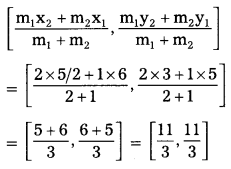
similarly, let P divide C(1, 4) and [5, \(\frac{7}{2}\)] in the ratio 2: 1 then

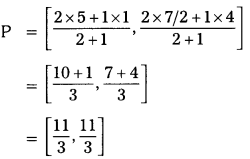
Question 5.
What do you observe? Justify the point that divides each median in the ratio 2: 1 is the centroid of a triangle. (Page No. 173)
Solution:
From the above problems, we conclude that the point “P” divides each median in the ratio 2 : 1 i.e., the three medians are concurrent at P, which is called centroid. A centroid divides each median in the ratio 2: 1.

Think – Discuss

Question 1.
The line joining points A(6, 9) and B(-6, -9) are given. (Page No. 175)

a) In which ratio does origin divide \(\overline{\mathbf{A B}}\) ? ‘And what it Is called for \(\overline{\mathbf{A B}}\) ?
Solution:
Given : A(6, 9) B(-6, -9)
Let origin 0(0, 0) divides AB in the ratio k : 1 internelly

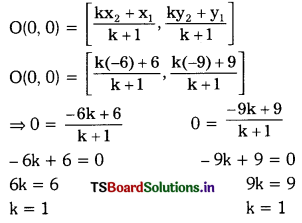
∴ Ratio is 1 : 1
Here the origin bisects \(\overline{\mathbf{A B}}\).
∴ Origin is called the mid point of \(\overline{\mathbf{A B}}\).

b) In which ratio does the point P(2, 3) divide \(\overline{\mathbf{A B}}\) ?
Solution:
Given : A(6, 9) B(-6, -9) and P(2, 3) divide
\(\overline{\mathbf{A B}}\) internally in the ratio say k : 1 then
P(2, 3) = \(\left[\frac{\mathrm{k}(-6)+6}{\mathrm{k}+1}, \frac{\mathrm{k}(-9)+9}{\mathrm{k}+1}\right]\)
⇒ 2 = \(\frac{-6 k+6}{k+1}\) and 3 = \(\frac{-9 k+9}{k+1}\)
⇒ 2k + 2 = -6k + 6 and 3k + 3
⇒ -9k + 9 = 8k ⇒ 6 – 2 and 3k + 9k
⇒ 9 – 3
⇒ 8k = 4 and 12k = 6;
k = \(\frac{4}{8}\) and k = \(\frac{6}{12}\)
k = \(\frac{1}{2}\) and k = \(\frac{1}{2}\)
∴ The ratio (k : 1) = [\(\frac{1}{2}\) : 1] = [1 : 2].

c) In which ratio does the point P(-2, -3) divide \(\overline{\mathbf{A B}}\) ?
Solution:
Let Q divide \(\overline{\mathbf{A B}}\) in the ratio say k : 1 internally, then,
P(-2, -3) = \(\left[\frac{\mathrm{kx}_2+\mathrm{x}_1}{\mathrm{k}+1}, \frac{\mathrm{ky}_2+\mathrm{y}_1}{\mathrm{k}+1}\right]\)
(-2, -3) = \(\left[\frac{\mathrm{k}(-6)+6}{\mathrm{k}+1}, \frac{\mathrm{k}(-9)+9}{\mathrm{k}+1}\right]\)
-2 = \(\frac{-6 \mathrm{k}+6}{\mathrm{k}+1}\) and \(\frac{-9 \mathrm{k}+9}{\mathrm{k}+1}\) = -3
-2 = \(\frac{-6 \mathrm{k}+6}{\mathrm{k}+1}\)
-6k + 6 = -2k – 2
-6k + 2k = -2 – 6
-4k = -8
k = \(\frac{-8}{-4}\) k = 2
and \(\frac{-9 \mathrm{k}+9}{\mathrm{k}+1}\) = -3
-9k + 9 = -3k – 3
-9k + 3k = -3 – 9
-6k = -12
k = \(\frac{-12}{-6}\) k = 2
∴ Ratio is k : 1 = 2 : 1

d. In how many equal parts is \(\overline{\mathbf{A B}}\) divided by P & Q ?
Solution:
Since P, Q divided \(\overline{\mathbf{A B}}\) in the ratio 1 : 2 & 2 : 1
\(\overline{\mathbf{A B}}\) is divided into 3 equal parts by P & Q.

e. What do we call P & Q for \(\overline{\mathbf{A B}}\) ?
Solution:
P & Q are the points of trisection of \(\overline{\mathbf{A B}}\).

Try This

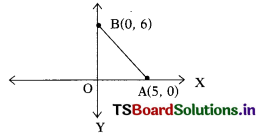
Question 1.
Take a point A on X—axis and B on Y—axis and find area of the triangle AOB. Discuss with your friends what did they
do. (Page No. 178)

Solution:
[∵ Axes are ⊥^er to each other].
consider the points A(5, 0), B(0, 6)
ΔAOB = \(\frac{1}{2}\) × b × h
= \(\frac{1}{2}\) × 6 × 5 = 15 sq. units.
Area of Δ^le A (x, 0), 0(0, 0), B(0, y) is \(\frac{1}{2}\)xy.

Question 2.
Find the area of the square formed by (0, -1) (2, 1) (0, 3) and (-2, 1) taken are as vertices. (Page No. 181)

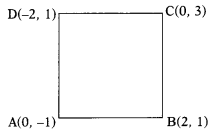
Solution:
Let A(0, -1), B(2, 1), C(0, 3) and D(-2, 1) are the vertices of square.
Area of the square ABCD = side² = AB²
But AB = \(\sqrt{\left(\mathrm{x}_2-\mathrm{x}_1\right)^2+\left(\mathrm{y}_2-\mathrm{y}_1\right)^2}\)
= \(\sqrt{(2-0)^2+(1+1)^2}\)
= \(\sqrt{2^2+2^2}\)
= \(\sqrt{4+4}\) = \(\sqrt{8}\)
∴ Area of square = \(\sqrt{8}\) × \(\sqrt{8}\)
= 8 sq. units

Think – Discuss

Question 1.
Let A(x₁, y₁), B(x₂, y₂) C(x₃, y₃). Then find the area of the following triangles in a plane. And discuss with your friends in groups about the area of that triangle. (Page No. 178)
i)

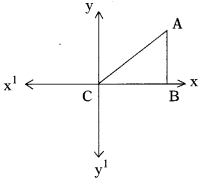
Solution:
Given ΔAOB where A(x₁, y₁), B(x₂, y₂), C(x₃, y₃) But we know that the origin O is (0, 0) which is given as C
Now \(\overline{\mathrm{CB}}\) = x₂ – x₃ = x₂ – 0 = x₂
\(\overline{\mathrm{AB}}\) = y₁ – y₂ = y₁ – 0 = y₁
∴ Area of ΔABC = \(\frac{1}{2}\)bh
Δ = \(\frac{1}{2}\) × b × h
= \(\frac{1}{2}\) × BC × AB
= \(\frac{1}{2}\) |(x₁ – x₃) × (y₂ – y₁)| sq. units

Question 2.
Find the area of the triangle formed by the following points. (Page No. 181)
i) (2, 0) (1, 2) (1, 6)
ii) (3, 1) (5, 0) (1, 2)
iii) (-1. 5, 3) (6, 2) (-3, 4).
What do you observe?
Solution:
i) (2, 0) (1, 2) (1, 6)
Take the third point as (-1, 6)
∆ = \(\frac{1}{2}\)|x₁(y₂ – y₃) + x₂(y₃ – y₁) + x₃(y₁ – y₂)|
∆ = \(\frac{1}{2}\) |2(2 – 6) + 1(6 – 0) – 1(0 – 2)|
= \(\frac{1}{2}\) |-8 + 6 + 2| = 0

ii) (3, 1) (5, 0) (1, 2)
Solution:
∆ = \(\frac{1}{2}\) | 3(0 – 2) + 5(2 – 1) + 1(1 – 0)|
= \(\frac{1}{2}\) |-6 + 5 + 1|
=0

iii) (-1.5, 3) (6, 2) (-3, 4)
Solution:
∆ = \(\frac{1}{5}\) | -1.5(2 – 4) + 6(4 – 3) – 3(3 – 2)|
= \(\frac{1}{2}\) | 3 + 6 – 3| = \(\frac{6}{2}\)
= 3 sq.units.

What do you observe?
Solution:
We observe that the area formed by above (i) & (ii) triangles is zero.

Plot these points on three different graphs. What do you observe?
Solution:

Solution:
We observe that the points are collinear.

Can we have a triangle with ‘0’ square units. (Page No. 181)
Solution:
No.

What does it mean?
Solution:
If the area of the triangle formed by any three points is zero, it means the points are collinear.

Do This

Question 1.
Find the area of the triangle whose vertices are
i) (5, 2), (3, -5), (-5, -1)
ii) (6, -6), (3, -7) and (3, 3) (Page No. 180)
Solution:
Given : The vertices of the triangle are (5, 2) (3, -5) (-5, -1)
Area of the triangle,
∆ = \(\frac{1}{2}\) | x₁(y₂ – y₃) + x₂(y₃ – y₁) + x₃(y₁ – y₂)
= \(\frac{1}{2}\) | 5(-5 – (-1)) + 3((-1) – 2) + (-5)(2 – (-5))|
= \(\frac{1}{2}\) |5 × (-4) + 3 × (-3) + (-5) × 7|
= \(\frac{1}{2}\) |-20 – 9 – 35|
= \(\frac{1}{2}\) |-64|
= \(\frac{64}{2}\)
= 32 sq. units.

ii) (6, -6), (3, -7) and (3, 3)
Solution:
Given : The vertices of a triangle are (6, -6), (3, -7), (3, 3)
Area of a triangle,
∆ = \(\frac{1}{2}\) | x₁(y₂ – y₃) + x₂(y₃ – y₁) + x₃(y₁ – y₂)
= \(\frac{1}{2}\) |6(-7 – 3) + 3(3 + 6) + 3(-6 + 7)|
= \(\frac{1}{2}\) |-60 + 27 + 3|
= \(\frac{1}{2}\) |-30|
= \(\frac{30}{2}\) = 15 sq. units

Question 2.
Verify whether the following points are collinear or not. (Page No. 182)

i) (1, -1), (4, 1), (-2, -3)
Solution:
Given : Three points
(1, -1), (4, 1), (-2, -3)
Area of ∆ = \(\frac{1}{2}\) | x₁(y₂ – y₃) + x₂(y₃ – y₁) + x₃(y₁ – y₂)
= \(\frac{1}{2}\) |1(1 + 3) + 4(-3 + 1) + (-2)(-1 – 1)|
= \(\frac{1}{2}\)|4 – 8 + 4| = 0
As the area of the triangle is “O”, the three points are collinear.

ii) (1, -1) (2, 3) (2, 0)
Solution:
Given points are (1, -1) (2, 3) (2, 0)
∆ = \(\frac{1}{2}\) | x₁(y₂ – y₃) + x₂(y₃ – y₁) + x₃(y₁ – y₂)
= \(\frac{1}{2}\) |1(3 – 0) + 2(0 + 1) + 2(-1 – 3)|
= \(\frac{1}{2}\)|3 + 2 – 8|
= \(\frac{1}{2}\)|-3|
= \(\frac{3}{2}\) ≠ 0
∆ ≠ 0
Hence the points are not collinear.

iii) (1, -6) (3, -4) (4, -3)
The given points are (1, -6) (3, -4) (4, -3)
Area of a triangle
∆ = \(\frac{1}{2}\) | x₁(y₂ – y₃) + x₂(y₃ – y₁) + x₃(y₁ – y₂)
= \(\frac{1}{2}\)|1(-4 + 3) + 3(-3 + 6) + 4(-6 + 4)|
= \(\frac{1}{2}\)|-1 + 9 – 8| = = \(\frac{1}{2}\)|0| = 0
∆ = 0, the points are collinear.

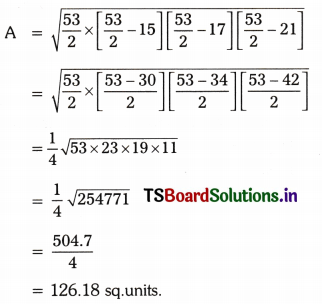
Question 3.
Find the area of the triangle whose lengths of sides are 15m, 17m, 21m (use Herons formula) (Page No. 183)
Solution:
Given : The sides of a triangle
a = 15m, b = 17m, c = 21m
S = \(\frac{a+b+c}{2}\)
= \(\frac{15+17+21}{2}\)
= \(\frac{53}{2}\)
Heron’s formula.
∆ = \(\sqrt{s(s-a)(s-b)(s-c)}\)

Question 4.
Find the area of the triangle formed by the points (0, 0) (4, 0) (4, 3) by using Heron’s formula. (Page No. 183)
Solution:
The given points are 0(0, 0), A(4, 0), B(4, 3) then the sides

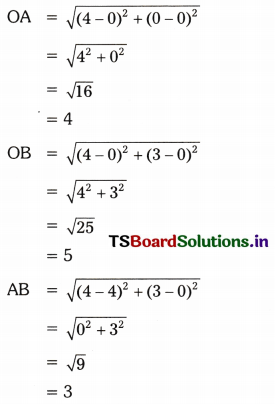
Let a = 4, b = 5, c = 3 then s = \(\frac{a+b+c}{2}\)
= \(\frac{4+5+3}{2}\) = \(\frac{12}{2}\) = 6
Heron’s formula
∆ = \(\sqrt{s(s-a)(s-b)(s-c)}\)
= \(\sqrt{6 \times(6-4)(6-5) \times(6-3)}\)
= \(\sqrt{6 \times 2 \times 1 \times 3}\)
= \(\sqrt{36}\)
= 6 sq. units.

Do This

Question 1.
Plot these points on the co-ordinate plane and join them
i) A(1, 2) B(-3, 4) C(-7, -1)
ii) P(3, -5), Q(5, -1), R(2, 1), S(1, 2)
Which gives a straight line?
Which does not? Why?
Solution:
i) A(1, 2), B(-3, 4), C(-7, -1) ABC gives the straight line because the points lie on same line.

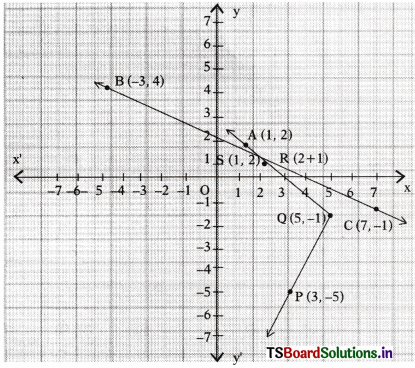
ii) P(3, -5) Q(5, -1) R(2, 1) S(1, 2) PQRS doesn’t give straight line because the points are not lie on same line.

Question 2.
Find the slope of with the given end points.
i) A(4, -6), B(7, 2)
Solution:
Slope m = \(\frac{y_2-y_1}{x_2-x_1}\)
= \(\frac{2+6}{7-4}\) = \(\frac{8}{3}\)

ii) A(8, -4), B(-4, 8)
Solution:
Slope m = \(\frac{y_2-y_1}{x_2-x_1}\) = \(\frac{8+4}{-4-8}\) = \(\frac{12}{-12}\) = -1

iii) A (-2, -5), B(1, -7)
Solution:
Slope m = \(\frac{y_2-y_1}{x_2-x_1}\) = \(\frac{-7+5}{1+2}\) = \(\frac{-2}{3}\)

Think — Discuss

Question 1.
Is y = x + 7 represent a straight line? Draw the line on the co-ordinate plane. At which point does this line intersect Y—axis? How much angle does it make with X — axis? Discuss with your friends. (Page No. 185)
Solution:
Yes, y = x + 7 represents a straight line.


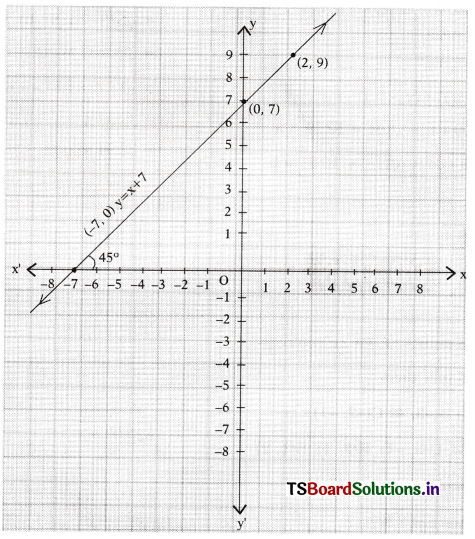
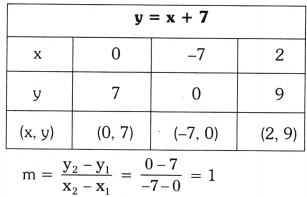
tan θ = 1 = tan 45°
∴ θ = 45°
Angle made by y = x + 7 with X — axis is 45°
[∵ (0, 7) & (-7, 0) are equidistant from the origin and hence the triangle formed is right isosceles triangle.

Question 2.
Find the slope AB with the points lying on A(3, 2) B(-8, 2). when the line \(\overline{\mathbf{A B}}\) parallel to X—axis ? Why ? Think and discuss with our friends in groups. (Page No. 188)
Solution:
Given : A(3, 2) B(-8, 2) then slope
m = \(\frac{\mathrm{y}_2-\mathrm{y}_1}{\mathrm{x}_2-\mathrm{x}_1}\)
= \(\frac{2-2}{-8-3}\)
= \(\frac{0}{-11}\) = 0
Yes, the line is parallel to X – axis as the points are of the form (x₁, k) (x₂, k).

Try This

Find the slope of \(\overline{\mathrm{AB}}\) with the points lying on (Page No. 188)

Question 1.
A(2, 1), B(2, 6)
Solution:
m = \(\frac{\mathrm{y}_2-\mathrm{y}_1}{\mathrm{x}_2-\mathrm{x}_1}\)
= \(\frac{6-1}{2-2}\) = \(\frac{5}{0}\) = ∞

Question 2.
A(-4, 2) B(-4, -2)
Solution:
m = \(\frac{\mathrm{y}_2-\mathrm{y}_1}{\mathrm{x}_2-\mathrm{x}_1}\)
= \(\frac{-2-2}{-4+4}\) = \(\frac{-4}{0}\) = ∞

Question 3.
A(-2, 8) B(-2, -2)
Solution:
m = \(\frac{\mathrm{y}_2-\mathrm{y}_1}{\mathrm{x}_2-\mathrm{x}_1}\)
= \(\frac{-2-8}{-2+2}\) = \(\frac{-10}{0}\) = ∞

Question 4.
Justify that the line \(\overline{\mathbf{A B}}\) line segment formed by given points in the above three
examples is parallel to Y—axis. What can you say about their slope ? Why?
Solution:
In the above problems, all points are of the form (k, y) where k is fixed number and y is variable.
All lines in the above problems are parallel to Y-axis.