TS 10th Class Maths Solutions Chapter 10 Mensuration InText Questions

Students can practice 10th Class Maths Study Material Telangana Chapter 10 Mensuration InText Questions to get the best methods of solving problems.

TS 10th Class Maths Solutions Chapter 10 Mensuration InText Questions

Try This

Question 1.
Consider the following situations. In each find out whether you need volume or area and why ? (Page No.245)

1. Quantity of water inside a bottle.
2. Canvas needed for making a tent.
3. Number of bags inside the lorry.
4. Gas filled in a cylinder.
5. Number of match sticks that can be put in match box.

Solution:

1. Volume : 3-d shape
2. Area : L.S.A. / T.S.A
3. Volume : 3-d shape
4. Volume : 3-d shape
5. Volume : 3-d shape

Question 2.
State 5 more such examples and ask your friends to choose what they need ? (AS₃) (Page No. 245)
Solution:

1. To paint a pillar in the shape of a cylinder.
2. To white wash the walls of a house.
3. To find the quantity of rich in a heap of rice.
4. An object is the form of a cone having a herispherical shape on its top. Quantity of ice-cream to be filled in it.
5. Later flowing through a cylindricalipipe.

Question 3.
Break the pictures in the previous figure into solids of known shapes. (AS₅) (Page No. 246)
Solution:

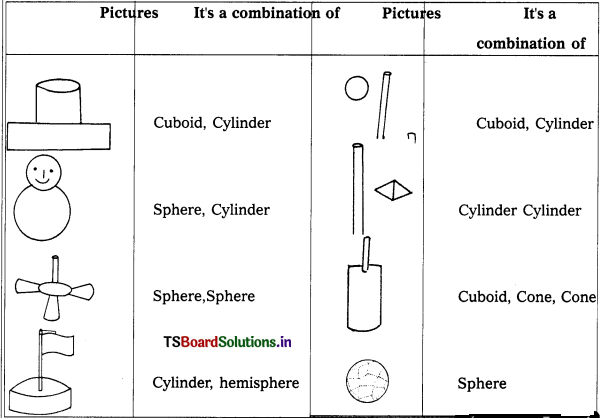
Question 4.
Think of 5 more objects around you that can be seen as a combination of shapes. Name the shapes that combined to make them. (AS₃) (Page No. 246)
Solution:
Student’s Activity.

Try This

Question 1.
Use known solid shapes and make as many objects (by combining more than two) as possible that you come across in
your daily life.
(Hint : Use clay, or balls, pip€s. paper cones, boxes like cube, cuboid etc.) (AS4, AS5) (Page No. 252)
Solution:
Student’s Activity.

Think – Discuss

Question 1.
A sphere is inscribed in a cylinder. Is the surface of the sphere equal to the curved surface of the cylinder ? If yes, explain how. (AS2, AS3) (Page No. 252)

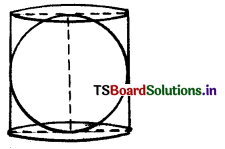
Solution:
Yes, the surface area of the sphere is equal to the curved surface area of the cylinder.
Let the radius of this cylinder be ‘r’ and its height ‘h’
Then its curved surface area = 2πrh
= 2πr (r + r)
∴ height = diameter of the sphere
= diameter of the cylinder
= 2r
= 2πr(2r)
= 4πr²
And surface area of the sphere = 4πr²
∴ C.S.A of cylinder = Surface area of sphere.

Try This

Question 1.
If the diameter of the cross-section of a wire is decreased by 5% by what percentage should the length be increased so that the volume remains the same ? (AS4) (Page No. 257)
Solution:
Radius of wire r = r and length = h₁
diameter of cross – section of wire d₁ = 2r
decreased diameter 5%
= 2r × \(\frac{5}{100}\) = \(\frac{\mathrm{r}}{10}\)
∴ decreased radius r₂ = 2r – \(\frac{\mathrm{r}}{20}\)
= \(\frac{19\mathrm{r}}{10}\) × \(\frac{1}{2}\) = \(\frac{19 \mathrm{r}}{20}\)
volume of the wire V₁ = πr₁²h₁, length = h₂ (after increased) volume of the wire V₁ (after increased) = πr₂²h₂ volumes are equal. So v₁ = v₂
πr₁²h₁ = πr₂²h₂
πr²h₁ = π \(\left(\frac{19 r}{20}\right)^2\) × h₂
h₁ = \(\frac{361}{400}\) × h₂
h₁ = \(\frac{361}{400}\) h₂
h₂ = \(\frac{400 \mathrm{~h}_1}{361}\)
increased length = h₂ – h₁
= \(\frac{400 \mathrm{~h}_1}{361}\) – h₁
= \(\frac{400 \mathrm{~h}_1-361 \mathrm{~h}_1}{361}\) = \(\frac{39 \mathrm{~h}_1}{361}\)
increased percentage
= 100 × \(\frac{39 \mathrm{~h}_1}{361}\) × \(\frac{\mathrm{h}_1}{\mathrm{~h}_1}\)
= \(\frac{3900}{361}\) = 10.8%

Question 2.
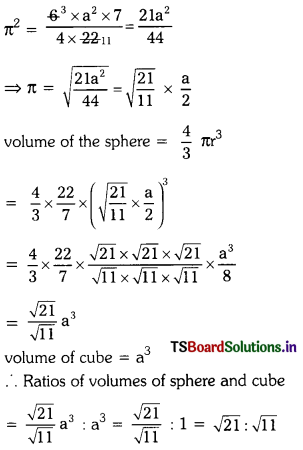
Surface areas of a sphere and cube are equal then find the ratio of their volumes. (AS4)(Page No. 257)
Solution:
radius of sphere = r, and side of cube = a
surface area of sphere = 4πr²
surface area of cube = 6a²
surface area of sphere = surface area of cube (given)
4πr² = 6a² ⇒ 4 × \(\frac{22}{7}\) × π² = 6 × a²

Think – Discuss

Question 1.
Which barrel shown in the below figure can hold more water ? Discuss with your friends. (AS₂, AS₃) (Page No. 262)

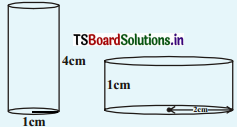
Solution:
r₁ = \(\frac{1}{2}\) = 0.5 cm; h₁ = 4 cm
Volume of the 1^st barrel = πr²h
= \(\frac{22}{7}\) × 0.5 × 0.5 × 4 = 3.142 cm³

r₂ = \(\frac{4}{2}\) = 2 cm ; h = 1 cm
Volume of the 2^nd barrel
V = πr²h = \(\frac{22}{7}\) × 2 × 2 × 1
= 12.57 cm³
Hence, the volume of the 2^nd barrel is more than the first barrel.

Do This

Question 1.
A copper rod of diameter 1cm and length 8 cm is drawn into a wire of length 18m of uniform thickness. Find the thickness of wire. (AS₄) (Page No. 263)
Solution:
Volume of the copper rod(Cylinder) = πr²h
= \(\frac{22}{7}\) × \(\frac{1}{2}\) × \(\frac{1}{2}\) × 8
= \(\frac{44}{7}\) cm²
If ‘r’ is the radius of the wire, then its volume = πr²h
∴ The volume of rod is equal to the volume of the wire. We have
⇒ \(\frac{22}{7}\) × r² × 18 m = \(\frac{44}{7}\) cm³
⇒ r² = \(\frac{44}{7}\) × \(\frac{7}{22}\) × \(\frac{1}{1800}\)
[∴ 18m = 18 × 100 cm]
⇒ r² = \(\frac{1}{900}\)
⇒ r = \(\frac{1}{30}\) cm = \(0.0 \overline{3}\) cm
∴ Thickness = d = 2 × 0.03 = 0.06 cm

Question 2.
Pravali house has a water tank in the shape of a cylinder on the roof. This is filled by pumping water from a sumpfan underground tank) which is in the shape of a cuboid. The sump has dimensions 1.57 m × 1.44m × 9.5 cm. The water tank has radius 60 cm. and height 95 cm. Find the height of the water left in the sump after the water tank has been completely filled with water from the sump which had been full of water. Compare the ca¬pacity of the tank with that of the sump. (AS₄) (Page No. 263)
Solution:
Volume of the water in the sump = [v = lbh]
= 1.57 × 1.44 × 0.95
(∵ 9.5 cm = \(\frac{9.5}{100}\) m = 0.95 m).
= 2.14776 m³ = 2147160 cm³
Volume of the tank on the roof = πr²h
= 3.14 × 60 × 60 × 95 = 1073880 cm³
∴ Volume of the water left in the sump after filling the tank
= 2147760 – 1073880
= 1073880 cm³
Let the height of the water in the tank be h.
∴ 157 × 144 × h = 1073880
h = \(\frac{1073880}{157 \times 144}\) = 47.5 cm
∴ Ratio of the volume of the sump and tank
= 21447760 : 1073880 = 2 : 1
∴ Sump can hold two times the water that can be hold in the tank.