TS 10th Class Maths Solutions Chapter 1 Real Numbers Optional Exercise

Students can practice TS 10th Class Maths Solutions Chapter 1 Real Numbers Optional Exercise to get the best methods of solving problems.

TS 10th Class Maths Solutions Chapter 1 Real Numbers Optional Exercise

Question 1.
Can the number 6ⁿ, n being a natural number, end with the digit 5 ? Give reason.
Solution:
Given number = 6ⁿ; n ∈ N
6ⁿ to be end in 5; it should be divisible by 5.
6ⁿ = (2 × 3)ⁿ
The prime factors of 6ⁿ are 2 and 3.
∴ It can’t end with the digit 5.

Question 2.
Is 7 × 5 × 3 × 2 + 3 a composite number? Justify your answer.
Solution:
Given : 7 × 5 × 3 × 2 + 3
= 3(7 × 5 × 2 + 1)
= 3 × (70 + 1)
= 3 × 71
The given number has two factors namely 3 and 71.
∴ Hence it is a composite number.

Question 3.
Prove that (2\(\sqrt{3}\) + \(\sqrt{5}\) is an irrational number. Also check whether (2\(\sqrt{3}\) + \(\sqrt{5}\))(2\(\sqrt{3}\) – \(\sqrt{5}\)) is rational or irrational.
Solution:
To prove : 2\(\sqrt{3}\) + \(\sqrt{5}\) is an irrational number. On contrary, let us suppose that 2\(\sqrt{3}\) + \(\sqrt{5}\) be a rational number then 2\(\sqrt{3}\) + \(\sqrt{5}\) = \(\frac{\mathrm{p}}{\mathrm{q}}\)
Squaring on both sides, we get

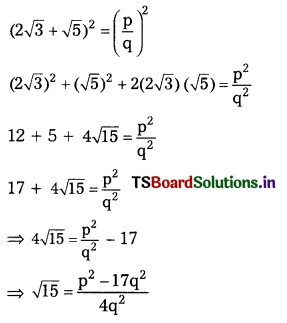
L.H.S = an irrational number
R.H.S. = p, q being integers, \(\frac{p^2-17 q^2}{4 q^2}\) is a rational number.
This is a contradiction to the fact that \(\sqrt{15}\) is an irrational. This is due to our assumption that 2\(\sqrt{3}\) + \(\sqrt{5}\) is a rational. Hence our assumption is wrong and 2\(\sqrt{3}\) + \(\sqrt{5}\) is an irrational number.
Also,
(2\(\sqrt{3}\) + \(\sqrt{5}\)) (2\(\sqrt{3}\) – \(\sqrt{5}\)) = (2\(\sqrt{3}\))² -(\(\sqrt{5}\))²
[∵ (a + b) (a – b) = a² – b²]
= 4 × 3 – 5 = 12 – 5 = 7, a rational number.

Question 4.
If x² + y² = 6xy, prove that 2 log (x +y) = logx + logy + 3 log 2
Solution:
Given : x² + y2² = 6xy Adding both sides
⇒ x² + y² + 2xy = 6xy + 2xy (x + y)² = 8xy
Taking logarithms on both sides
log (x + y)² = log 8xy
⇒ 2 log(x + y) = log 8 + log x + log y
[∵ log x^m = mlog x]
[∵ log xy = log x + log y]
= log 2³ + log x + log y
⇒ 2log (x + y) = log x + log y + 3 log 2

Question 5.
Find the number of digits in 4²⁰¹³, if log₁₀ 2 = 0.3010.
Solution:
Given log₁₀2 = 0.3010
4²⁰¹³ = (2²)²⁰¹³ = 2⁴⁰²⁶
∴ log₁₀ 2⁴⁰²⁶ = 4026 log 2
[∵ log x^m = m log x]
= 4026 × 0.3010
= 1211.826 \(\simeq\) 1212
∴ 4²⁰¹³ has 1212 digits in its expansion.