TS 10th Class Maths Bits Chapter 4 Pair of Linear Equations in Two Variables

Solving these TS 10th Class Maths Bits with Answers Chapter 4 Pair of Linear Equations in Two Variables Bits for 10th Class will help students to build their problem-solving skills.

Pair of Linear Equations in Two Variables Bits for 10th Class

Question 1.
Which of the following pairs of equations represent inconsistent system ?
A) 2x + 3y = 8
5x – 4y = 3
B) 6x + 3y = 9
x – 8y = 0
C) 2x + 5y = 11
4x + 10 y = 21
D) 3x – 4y = 6
6x – 8y = 12
Answer:
C) 2x + 5y = 11
4x + 10 y = 21

Question 2.
The pair of linear equations 3x + 4y + 5 = 0 and 12x + 16 y + 15 = 0 have ……… solution (s).
A) Unique
B) Many
C) Two
D) No
Answer:
D) No

Question 3.
The pair of linear equations px + 2y = 5 and 3x + y = 1 has unique solutions if
A) p ≠ 6
B) p = 6
C) p = 5
D) p ≠ 5
Answer:
A) p ≠ 6

Question 4.
The lines represented by 8x + 2pγ = 2 and 2x + 5y + 1 = 0 are parallel if p =
A) \(\frac{-5}{4}\)
B) \(\frac{2}{7}\)
C) 10
D) \(\frac{3}{8}\)
Answer:
C) 10

Question 5.
Solution of the equations \(\sqrt{2}\)x + \(\sqrt{3}\)y = 0 and \(\sqrt{3}\)x – \(\sqrt{8}\)y = 0
A) x = 1
y = 0
B) x = 0
y = 1
C) x = 1
y = 1
D) x = 0
y = 0
Answer:
D) x = 0
y = 0

Question 6.
The pair of equations a₁x + b₁y + c₁ = 0 and a₂x + b₂y + c₂ = 0 has a unique solution, then
A) \(\frac{\mathrm{a}_1}{\mathrm{a}_2}\) ≠ \(\frac{\mathrm{b}_1}{\mathrm{b}_2}\)
B) \(\frac{a_1}{a_2}\) = \(\frac{b_1}{b_2}\) ≠ \(\frac{c_1}{c_2}\)
C) \(\frac{a_1}{a_2}\) = \(\frac{b_1}{b_2}\) = \(\frac{c_1}{c_2}\)
D) \(\frac{b_1}{b_2}\) ≠ \(\frac{c_1}{c_2}\)
Answer:
A) \(\frac{\mathrm{a}_1}{\mathrm{a}_2}\) ≠ \(\frac{\mathrm{b}_1}{\mathrm{b}_2}\)

Question 7.
If x = 1, then the value of y satisfying the equation \(\frac{5}{x}\) + \(\frac{3}{y}\) = 6
A) 3
B) +\(\frac{1}{3}\)
C) –\(\frac{1}{3}\)
D) 1
Answer:
A) 3

Question 8.
The value of y when \(\frac{x+y}{x y}\) = 2 and \(\frac{x-y}{x y}\) = 6 is
A) \(\frac{1}{4}\)
B) –\(\frac{1}{2}\)
C) –\(\frac{7}{4}\)
D) \(\frac{5}{4}\)
Answer:
A) \(\frac{1}{4}\)

Question 9.
If ax + by = c and px + qy = r has unique solution, then
A) \(\frac{a}{b}\) = \(\frac{p}{q}\)
B) ab = pq
C) \(\frac{a}{q}\) = \(\frac{b}{p}\)
D) aq ≠ bp
Answer:
A) \(\frac{a}{b}\) = \(\frac{p}{q}\)

Question 10.
If 5x + py + 8 =0 and 10x + 15y + 12 = 0 has no solution, then p =
A) 7\(\frac{1}{2}\)
B) 6\(\frac{1}{2}\)
C) 7
D) 4
Answer:
A) 7\(\frac{1}{2}\)

Question 11.
y = 5x is a line
A) parallel to x – axis
B) parallel to y – axis
C) parallel to x = 5y
D) passes through the origin
Answer:
D) passes through the origin

Question 12.
The point (-3,-8) is in the ____ quadrant.
A) Q₁
B) Q₂
C) Q₃
D) Q₄
Answer:
C) Q₃

Question 13.
The point (7, -5) is in the _____ quadrant.
A) I
B) II
C) III
D) IV
Answer:
D) IV

Question 14.
Find the value of x if y = \(\frac{3}{4}\) x and 5x + 8y = 33
A) 2
B) 3
C) 4
D) -3
Answer:
B) 3

Question 15.
\(\frac{120}{x}\) + \(\frac{12}{x}\) = 11, then x =
A) 132
B) 11
C) 12
D) 13
Answer:
C) 12

Question 16.
Which of the following equations is not a linear equation ?
A) x – 3y = 2x + y + 8
B) 4x² – 6y = y + 6
C) 8x – 3 = 5y + 7
D) 3x = 4y
Answer:
B) 4x² – 6y = y + 6

Question 17.
Which of the following number is a solution for the equation 3(7 – 3y) + 4y = 16 ?
A) 0
B) -1
C) 1
D) 2
Answer:
C) 1

Question 18.
When two lines in the same plane may in-tersect ?
A) At two points
B) At many points
C) At only one point
D) None
Answer:
C) At only one point

Question 19.
In the following values of x and y satisfies the equation 4x + 3y = 110
A) (10, 20)
B) (15, 30)
C) (10, 30)
D) (20, 10)
Answer:
D) (20, 10)

Question 20.
3x + 2y – 80 = 0; 4x + 3y – 110 = 0 solution for this linear equations is
A) Unique
B) Infinite
C) Two
D) No
Answer:
A) Unique

Question 21.
x + 2y – 30 = 0; 2x + 4y – 66 = 0 these lines represent
A) Intersecting lines
B) Parallel lines
C) Coincident lines
D) None of these
Answer:
B) Parallel lines

Question 22.
The point of intersection of x + y = 6 and x – y = 4 is ………. (A.P. Mar. 16)
A) (5, 1)
B) (1, 5)
C) (2, 4)
D) (4, 6)
Answer:
A) (5, 1)

Question 23.
A pair of linear equations in two variables are 2x – y = 4 and 4x – 2y = 6. The pair of equations are …….. (T.S. Mar’15)
A) Consistent
B) Dependent
C) Inconsistent
D) Cannot say
Answer:
C) Inconsistent

Question 24.
The value of ‘x’ in the equation 3x – (x – 4) = 3x + 1 is …….. (T.S. Mar’16)
A) -3
B) 0
C) 3
D) 10
Answer:
C) 3

Question 25.
The sum of squares of two consecutive positive even numbers is 340, then the numbers are …… (A.P. Mar. 15)
A) 12, 14
B) 10, 12
C) 14, 16
D) 16, 18
Answer:
A) 12, 14

Question 26.
Length of the dark line given in the; diagram (A.P. Mar. 16)

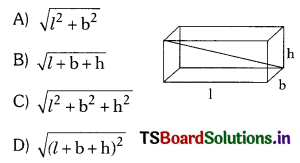
Answer:
C) \(\sqrt{l^2+\mathrm{b}^2+\mathrm{h}^2}\)

Question 27.
500x + 240y = 8, 130x + 240y = \(\frac{43}{10}\) then x = ………..
A) \(\frac{9}{200}\)
B) \(\frac{7}{20}\)
C) \(\frac{1}{100}\)
D) \(\frac{1}{10}\)
Answer:
C) \(\frac{1}{100}\)

Question 28.
In the above problem y = ……….
A) 1
B) 0
C) 10
D) none
Answer:
D) none

Question 29.
If the equations a₁x + b₁y + c₁ = 0 and a₂x + b₂y + c₂ = 0 are consistent then ……….
A) \(\frac{a_1}{a_2}\) ≠ \(\frac{b_1}{b_2}\)
B) \(\frac{a_1}{a_2}\) = \(\frac{b_1}{b_2}\)
C) \(\frac{a_1}{a_2}\) = 1
D) none
Answer:
A) \(\frac{a_1}{a_2}\) ≠ \(\frac{b_1}{b_2}\)

Question 30.
Sita has pencils and pens which are together 40 in number. If she has 5 less pencils and 5 more pens the number of pens become four times the number of pencils. Represent this situation in a linear equation form.  A) x – y = 40
B) x + y = 40
C) x – y = 7
D) all
Answer:
B) x + y = 40

Question 31.
The lines represented by 5x + 7y – 14 = 0 and 10x + 3y – 8 = 0 are ………. lines.
A) coincident
B) vertical
C) parallel
D) consistent
Answer:
D) consistent

Question 32.
The standard form of a linear equation is ……………
A) xa + y = 0
B) ax + by
C) ax + b = 0
D) ax + by + c = 0
Answer:
D) ax + by + c = 0

Question 33.
The lines 3x + 8y – 13 = 0 and -6x – 16y + 23 = 0 are ………. lines.
A) coincident
B) parallel
C) circular
D) none
Answer:
B) parallel

Question 34.
The lines represented by 5x + 3y – 7 = 0 and 6y + 10x – 14 = 0 are lines.
A) coincident
B) parallel
C) intersecting
D) none
Answer:
A) coincident

Question 35.
The pairs of equations 4x – 2y + 6 = 0 and 2x – y + 8 = 0 has …… solutions(s).
A) 1
B) 12
C) No solution
D) 10
Answer:
C) No solution

Question 36.
The number of solutions to the pair of equations 6x – 7y + 8 = 0 and 12x – 14y + 10 = 0 is
A) 1
B) 20
C) 3
D) infinite
Answer:
D) infinite

Question 37.
The number of solutions to the pair of equations 11x – 7y = 6 and 4x + 9y = 8 is
A) 4
B) 3
C) 7
D) 1
Answer:
D) 1

Question 38.
If the pair of equations kx + 14y + 8 = 0 and 3x + 7y + 6 = 0 has a unique solution then
A) k ≠ 6
B) k = 0
C) k = 7
D) none
Answer:
A) k ≠ 6

Question 39.
a₁x + b₁y₁ + c₁ = 0 and a₂x + b₂y + c₂ = 0 are ……… equations.
A) parallel
B) pair of linear
C) consistent
D) none
Answer:
B) pair of linear

Question 40.
If \(\frac{a_1}{a_2}=\frac{b_1}{b_2}=\frac{c_1}{c_2}\) then the lines will have …….. solutions.
A) infinite
B) 2
C) 3
D) 7
Answer:
A) infinite

Question 41.
3x – 2y + 6 = 0, 6x – 4y + 8 = 0 represents ……. lines
A) consistent
B) inconsistent
C) circle
D) parallel
Answer:
D) parallel

Question 42.
5x – 2y – 10 = 0,10x – 4y – 20 = 0 these are ……… lines.
A) consistent
B) parallel
C) intersecting
D) none
Answer:
A) consistent

Question 43.
The number of solutions to 4x + 6y – 7 = 0 and 8x + 5y – 8 = 0 is ………..
A) 14
B) 3
C) 4
D) 1
Answer:
D) 1

Question 44.
\(\frac{2}{x}\) + \(\frac{3}{y}\) = 2, \(\frac{12}{x}\) – \(\frac{9}{y}\) = 3 then x = ……….
A) 1
B) 4
C) 2
D) none
Answer:
C) 2

Question 45.
In the above problem y = ………
A) 2
B) -1
C) 7
D) 3
Answer:
D) 3

Question 46.
If 2^x + 3^y = 17 and 2^x+2 – 3^y+1 = 5, then y = ……….
A) 2
B) 3
C) 1
D) 7
Answer:
A) 2

Question 47.
In the above problem x = ………
A) 2
B) 4
C) 3
D) none
Answer:
C) 3

Question 48.
\(\frac{\mathbf{a x}}{\mathbf{b}}\) – \(\frac{\mathbf{b y}}{\mathbf{a}}\) = a + b, ax – by = 2ab then x = ……….
A) 3b
B) \(\frac{-3}{b}\)
C) 1
D) -2a
Answer:
A) 3b

Question 49.
In the above problem y = ………
A) – a
B) 2a
C) -a²
D) 3b – a
Answer:
A) – a

Question 50.
Slope of the line ax + by + c = 0 is ………
A) \(\frac{b}{a}\)
B) \(\frac{1}{a}\)
C) \(\frac{a}{b}\)
D) \(\frac{-a}{b}\)
Answer:
D) \(\frac{-a}{b}\)

Question 51.
The line ax + by + c = 0 does not passes through ………..
A) (0, 0)
B) (a, 0)
C) both A & B
D) none
Answer:
C) both A & B

Question 52.
If x + y = 7, x – y = 1 then 2x = ……….
A) 3
B) 4
C) 7
D) 8
Answer:
D) 8

Question 53.
In the above problem y = ………..
A) 3
B) 7
C) 1
D) 4
Answer:
A) 3

Question 54.
Slope of the line y = x is ………
A) 2
B) – 1
C) 1
D) none
Answer:
C) 1

Question 55.
The line x = 2015 is ……….
A) slope not defined
B) parallel to Y – axis
C) both A & B
D) none
Answer:
C) both A & B

Question 56.
x + \(\frac{6}{y}\) = 6, 3x – \(\frac{8}{y}\) then y = ………..
A) – 2
B) 4
C) 1
D) 2
Answer:
D) 2

Question 57.
In the above problem x = ……….
A) 3
B) 2
C) -1
D) 9
Answer:
A) 3

Question 58.
3x – 5y = -1, – y + x = -1 then (x, y) = ……..
A) (-2, -1)
B) (2, -1)
C) curve
D) none
Answer:
A) (-2, -1)

Question 59.
The value of y in -5x + 10y = 100 at x = 0 is ……….
A) 12
B) 9
C) -10
D) 10
Answer:
D) 10

Question 60.
If x + y = 36, then at y = -1, x = ………..
A) 38
B) 37
C) 80
D) 12
Answer:
B) 37

Question 61.
x + y = 10, x – y = – 4 then x = …………
A) 4
B) 3
C) 5
D) none
Answer:
B) 3

Question 62.
In the above problem y = ……….
A) 9
B) 4
C) 7
D) 13
Answer:
C) 7

Question 63.
Solution to 2x – 2y – 2 = 0,4x – 4y – 5 = 0 is …….
A) (1, 4)
B) (2, -1)
C) (8, –\(\frac{1}{4}\))
D) no solution
Answer:
D) no solution

Question 64.
The two lines 2x + y – 6 = 0 and 4x – 2y – 4 = 0 intersect at ……….
A) (2, 2)
B) (3, 2)
C) (1, -4)
D) (1, 4)
Answer:
A) (2, 2)

Question 65.
Solution to x – y = 1, 2x – 2y = 7 is ……..
A) (1, 1)
B) (1, 9)
C) (8, 4)
D) no solution
Answer:
D) no solution

Question 66.
Perimeter of rectangle = ……..
A) l + b
B) l – b
c) 2(l + b)
D) \(\frac{l+\mathrm{b}}{2}\)
Answer:
c) 2(l + b)

Question 67.
x + y = 2015 has …… number of solutions.
A) 10
B) 2014
C) 20
D) infinite
Answer:
D) infinite

Question 68.
px + 3y – (p – 3) = 0, 12x + py – p = 0 has infinitely many solutions then p = ………..
A) 7
B) 9
C) ± 71
D) ± 6
Answer:
D) ± 6

Question 69.
If 3x + 4y + 2 = 0, 9x + 12y + k = 0 represent coincident lines, then k = ………….
A) 12
B) 9
C) 6
D) 7
Answer:
C) 6

Question 70.
2x + 3y = 1, 3x – y = 7 then (x, y) = ……..
A) (2, -1)
(B) (-2, 1)
(C) (8, \(\frac{1}{4}\))
(D) (0, 3)
Answer:
A) (2, -1)

Question 71.
Slope of the line x = 2y is ………
A) 2
B) -2
C) 1
(D) \(\frac{1}{2}\)
Answer:
B) -2

Question 72.
If 7x – 8y = 9, then y = …………
A) 9 + 7x
B) \(\frac{9-7 x}{8}\)
C) \(\frac{9-7 x}{6}\)
D) none
Answer:
D) none

Question 73.
Slope of X – axis is ……….
A) 0
B) 1
C) -1
D) 2
Answer:
A) 0

Question 74.
Angle between any two parallel lines is …………
A) 70°
B) 0°
C) 100°
D) 180°
Answer:
B) 0°

Question 75.
4m – 2n = 2, 6m – 5n = 9 then n = ………..
A) 5
B) 4
C) 1
D) – 3
Answer:
D) – 3

Question 76.
In the above problem m = …………
A) – 1
B) 4
C) -31
D) 7
Answer:
A) – 1

Question 77.
2u + 3y = 2, 4u – 6y = 0 then y = ……….
A) \(\frac{1}{2}\)
B) 1
C) \(\frac{1}{31}\)
D) \(\frac{1}{3}\)
Answer:
D) \(\frac{1}{3}\)

Question 78.
In Q. No. 77 the value of u = …………
A) \(\frac{1}{21}\)
B) \(\frac{1}{2}\)
C) 2
D) 4
Answer:
B) \(\frac{1}{2}\)

Question 79.
Area of rectangle = ………….
A) l²b
B) \(\frac{l}{b}\)
C) lb
D) none
Answer:
C) lb

Question 80.
\(\frac{x+3}{2}\) – y = 2, \(\frac{x-3}{2}\) + 2y = 4 \(\frac{1}{2}\) then x = ………..
A) 1
B) 4
C) 51
D) none
Answer:
D) none

Question 81.
In the above problem y = ……….
A) 2
B) – 2
C) 7
D) 12
Answer:
A) 2

Question 82.
If ax + b = 0, then x = ………..
A) -b
B) –\(\frac{\mathrm{b}}{\mathrm{a}}\)
C) \(\frac{\mathrm{b}}{\mathrm{a}}\)
D) none
Answer:
B) –\(\frac{\mathrm{b}}{\mathrm{a}}\)

Question 83.
2x – 3y = -12 then at x = 0, y = ……….
A) 4
B) 6
C) 8
D) 12
Answer:
A) 4

Question 84.
Two parallel lines differ by ……….
A) circle
B) triangle
C) constant
D) none
Answer:
C) constant

Question 85.
x = 1 and y = –\(\frac{1}{2}\) then x – y = ………..
A) – 1
B) 1
C) –\(\frac{1}{2}\)
D) 7
Answer:
D) 7

Question 86.
If 99x + 101y = 499, 101x + 99y = 510 then x = ………
A) -1
B) 3
C) 4
D) 2
Answer:
B) 3

Question 87.
In the above problem y = ………
A) 3
B) 4
C) 2
D) 8
Answer:
C) 2

Question 88.
141x + 93y = 189, 93x + 141y = 45 then y = …….
A) – 1
B) 4
C) -2
D) 2
Answer:
A) – 1

Question 89.
In the above problem x = …….
A) 3
B) 4
C) 1
D) 2
Answer:
D) 2

Question 90.
If -x + y = – 10, then x = …………….
A) y – 3
B) y² – 1
C) y – 10
D) y + 10
Answer:
D) y + 10

Question 91.
(-a, -b) ∈ ………..
A) Q₂
B) Q₃
C) Q₁
D) Q₄
Answer:
B) Q₃

Question 92.
(2, 0) lies on ……….
A) Q₁
B) Q₂
C) x axis
D) y axis
Answer:
C) x axis

Question 93.
The line x – y = 8 intersects X – axis at ……….
A) (2, 3)
B) (1, 1)
C) (0, 8)
D) (8, 0)
Answer:
D) (8, 0)

Question 94.
The values of k for which the pair of linear equations 3x – 2y = 7 and 6x + ky + 11 = 0 has a unique solution is
A) all numbers except 4
B) all numbers expect – 4
C) 4
D) – 4
Answer:
B) all numbers expect – 4

Question 95.
The pair of linear equations -3x + 4y = 7 and \(\frac{9}{2}\) x – 6y + \(\frac{21}{2}\) = 0 has ……………….
A) infinite number of solutions
B) no solution
C) two solutions
D) unique solution
Answer:
A) infinite number of solutions

Question 96.
If ad ≠ bc then the pair of linear equations ax + by = p and cx + dy = q has ……….. solutions.
A) no
B) unique
C) 2
D) none
Answer:
C) 2

Question 97.
A line parallel to the line x + 2y + 1 = 0 is ………
A) x + y + 3 = 0
B) 2x + 4y + 1 = 0
C) x – y + 1 = 0
D) all
Answer:
B) 2x + 4y + 1 = 0

Question 98.
The pair of equations x = 3, y = 2 graphi-cally represent lines which ………
A) intersect at (3, 4)
B) intersect at (4, 3)
C) parallel
D) coincident
Answer:
A) intersect at (3, 4)

Question 99.
If the pair of equations 2x + y = 7 and 6x – py – 21 =0 has infinite number of solutions then p = ………..
A) 3
B) 4
C) 5
D) none
Answer:
D) none

Question 100.
The value of k for which the system of equations kx + 3y = 1,12x + ky = 2 has no solution is ………..
A) k = -1
B) k = 3
C) k = 2
D) k = -6
Answer:
D) k = -6

Question 101.
The line 3x + y = 7 intersects X- axis at ………..
A) (\(\frac{7}{2}\), 0)
B) (0, \(\frac{7}{2}\))
C (0, 1)
D) (0, 3)
Answer:
A) (\(\frac{7}{2}\), 0)

Question 102.
For what value of k, 2x + 3y = 4 and (k + 2)x + 6y = 3k + 2 will have infinitely many solutions ?
A) k = -1
B) k = 2
C) k = 7
D) none
Answer:
B) k = 2

Question 103.
\(\frac{x+1}{2}\) + \(\frac{y-1}{3}\) = 9, \(\frac{x-1}{3}\) + \(\frac{y+1}{2}\) = 8 then x =
A) 13
B) 17
C) -3
D) 10
Answer:
A) 13

Question 104.
In the above problem y = ……….
A) 4
B) – 3
C) 9
D) 7
Answer:
D) 7

Question 105.
If the equations (2m – 1)x + 3y – 5 = 0, 3x + (n – 1)y – 2 = 0 has infinite number of solutions then n = …………
A) 1
B) \(\frac{5}{11}\)
C) \(\frac{1}{5}\)
D) \(\frac{11}{5}\)
Answer:
D) \(\frac{11}{5}\)

Question 106.
In the above problem m = ………..
A) \(\frac{17}{4}\)
B) \(\frac{7}{4}\)
C) \(\frac{1}{2}\)
D) \(\frac{8}{3}\)
Answer:
A) \(\frac{17}{4}\)

Question 107.
A fraction becomes \(\frac{9}{11}\) if 2 is added to both numerator and denominator. If 3 is added to both numerator and denominator it be-comes is …………
A) \(\frac{17}{4}\)
B) \(\frac{7}{4}\)
C) \(\frac{1}{2}\)
D) \(\frac{8}{3}\)
Answer:
A) \(\frac{17}{4}\)

Question 108.
The ratio of incomes of two persons is 11:7 and the ratio of their expenditures is 9 : 5. If each of them manages to save ₹ 400 per month then the monthly income of first person is ………..
A) ₹ 2,200
B) ₹ 1,200
C) ₹ 800
D) ₹ 1010
Answer:
A) ₹ 2,200

Question 109.
In the above problem income of second person is ……….
A) ₹ 8,001
B) ₹ 1,100
C) ₹ 1,400
D) ₹ 4,100
Answer:
C) ₹ 1,400

Question 110.
The age of a father 8 years ago was 5 time that of his son 8 years. Hence, his age will be 8 years more than twice the age of his son. Then the present age of father is years. ( )
A) 80
B) 92
C) 24
D) 48
Answer:
D) 48

Question 111.
In the above problem age of son is ……… years.
A) 16
B) 96
C) 12
D) none
Answer:
A) 16

Question 112.
The two lines 2x – y = 1, x + 2y = 13 will intersect at …………
A) (5, 3)
B) (3, 5)
C) (1, 3)
D) (3, 9)
Answer:
B) (3, 5)

Question 113.
Identify parallel lines
A) 2x + 3y = 6, 8x + 12y = 9
B) x + y = 7, x – y = 1
C) 2x + y = 7, x – y = 1 :
D) 2x + y = 7, 3x – y = 7 .
Answer:
A) 2x + 3y = 6, 8x + 12y = 9

Question 114.
Solution to 2x + 3y = 12, 2y – 1 = x is ……….
A) (8, -1)
B) (3, 8)
C) (3, 2)
D) (1, -1)
Answer:
C) (3, 2)

Question 115.
The lines x – y = 1; 2x + y = 8 intersects at ……….
A) (1, 9)
B) (9, 3)
C) (3, 4)
D) none
Answer:
D) none

Question 116.
\(\frac{5}{x-1}\) + \(\frac{1}{y-2}\) = 2, \(\frac{6}{x-1}\) + \(\frac{-3}{y-2}\) = 1 If then x = ……….
A) 4
B) 7
C) -1
D) 3
Answer:
A) 4

Question 117.
In the above problem y = …………
A) 1
B) -1
C) 5
D) 9
Answer:
C) 5

Question 118.
If 2x + 3y = 10 and 2x – 3y = 6 then (x, y) = …………
A) (2, 5)
B) (\(\frac{2}{3}\), 4)
C) (0, 0)
D) (4, \(\frac{2}{3}\))
Answer:
D) (4, \(\frac{2}{3}\))