TS Inter 2nd Year Maths 2B Solutions Chapter 8 Differential Equations Ex 8(e)

Students must practice this TS Intermediate Maths 2B Solutions Chapter 8 Differential Equations Ex 8(e) to find a better approach to solving the problems.

TS Inter 2nd Year Maths 2B Solutions Chapter 8 Differential Equations Ex 8(e)

I.
Find the I.F. of the following differential equations by transforming them into linear form.

Question 1.
x \(\frac{d y}{d x}\) – y = 2x² sec² 2x.
Solution:
The given equation can be expressed as
\(\frac{d y}{d x}-\frac{y}{x}\) = 2x sec² 2x
This is of the form \(\frac{d y}{d x}\) + Py = Q where the
Integrating factor I.F = e^{∫ P dx}, P = – \(\frac{1}{x}\)
= e^{– ∫ \(\frac{1}{x}\) dx}
= e^{– log x}
= e^{log x-1} = \(\frac{1}{x}\)

Question 2.
y \(\frac{d y}{d x}\) – x = 2y³
Solution:
The given equation can be written as
\(\frac{d x}{d y}-\frac{x}{y}\) = 2y²
and the integrating factor I.F = e^{∫ P dy}
= e^{– ∫ \(\frac{1}{y}\) dy}
= e^{– log y}
= e^{log y-1} = \(\frac{1}{y}\).

II. Solve the following differential equations.

Question 1.
\(\frac{d y}{d x}\) + y tan x = cos³ x
Solution:
Given \(\frac{d y}{d x}\) + y tan x = cos³ x
which is of the form \(\frac{d y}{d x}\) + Py = Q where
P = tan x and Q = cos³ x
∴ Integrating Factor I.F. = e^{∫ P dx}
= e^{∫ tan x dx}
= e^{log sec x} = sec x
General solution is y. sec x = ∫ Q (I.F.) dx
= ∫ cos³ x sec x dx
= ∫ cos² x dx
= \(\int \frac{1+\cos 2 x}{2} d x=\frac{1}{2} x+\frac{1}{4} \sin 2 x\)
= \(\frac{1}{2}\) (x + sin x cos x) + c
\(\frac{y}{\cos x}\) = \(\frac{1}{2}\) (x + sin x cos x) + c
⇒ 2y = cos x (x + sin x cos x) + c cos x
= x cos x + sin x cos² x + c cos x is the solution.

Question 2.
\(\frac{d y}{d x}\) + y sec x = tan x
Solution:
This is of the form \(\frac{d y}{d x}\) + Py = Q
where P = sec x and Q = tan x
∴ Integrating Factor I.F. = e^{∫ sec x dx}
= e^{log (sec x + tan x)}
= sec x + tan x
∴ General solution is
y . e^{∫ P dx} = ∫ Q . e^{∫ P dx} dx + c
∴ y (sec x + tan x) = ∫ tan x (sec x + tan x) + c
= ∫ sec x tan x dx . ∫ tan² x dx
= sec x + ∫ (sec² x – 1) dx + c
= sec x + tan x – x + c
∴ y (sec x + tan x) sec x + tan x – x + c is the solution.

Question 3.
\(\frac{d y}{d x}\) – y tan x = e^x sec x.
Solution:
This is of the form \(\frac{d y}{d x}\) + Py = Q where
P = – tan x and Q = e^x sec x.
∴ Integrating Factor IF. = e^{∫ P dx} dx
= e^{∫ tan x} dx
= e^{log cos x} = cos x
∴ General solution is y . e^{∫ P dx} dx = ∫ Q . e^{∫ P dx} dx + c
∴ y . cos x = ∫ e^x sec x cos x dx + c
= ∫ e^x dx + c
= e^x dx + c
y = e^x sec x + c sec x. is the solution.

Question 4.
x \(\frac{d y}{d x}\) + 2y = log x.
Solution:
The equation can be written as
\(\frac{\mathrm{dy}}{\mathrm{dx}}+\frac{2}{\mathrm{x}} \mathrm{y}=\frac{\log \mathrm{x}}{\mathrm{x}}\)
This is of the form \(\frac{d y}{d x}\) + Py = Q where
I.F. = e^{∫ P dx} where P = \(\frac{2}{x}\), and Q = \(\frac{\pi}{2}\)
= e^{∫ \(\frac{2}{x}\)}
= e^{log x2} = x².
y . e^{∫ P dx} = ∫ Q . e^{∫ P dx} dx + c

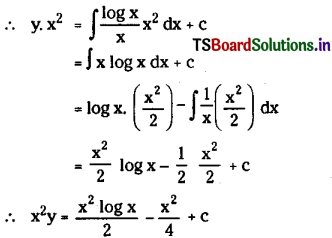
Question 5.
(1 + x²)\(\frac{d y}{d x}\) + y = e^tan-1 x
Solution:
The equation can be written as
\(\frac{d y}{d x}+\frac{y}{1+x^2}=\frac{e^{\tan ^{-1} x}}{1+x^2}\)

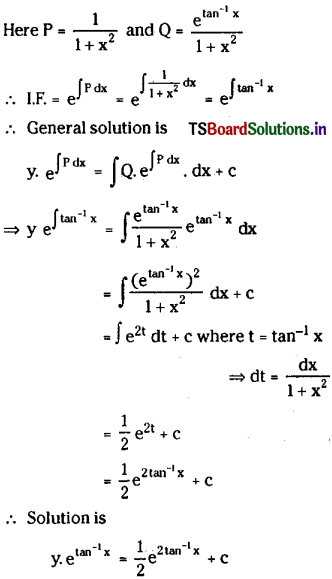
Question 6.
\(\frac{d y}{d x}+\frac{2 y}{x}\) = 2x²
Solution:
The given equation can be written as
\(\frac{d y}{d x}+\frac{2 y}{x}\) = 2x²
∴ I.F.= e^{∫ P dx}
= e^{∫ \(\frac{2}{x}\) dx}
= e^{2 log x}
= e^{log x2} = x²
∴ Solution is y . x² = ∫ 2x² . x² dx
= 2 ∫ x⁴ dx
= 2 \(\frac{x^5}{5}\) + c.

Question 7.
\(\frac{d y}{d x}+\frac{4 x}{1+x^2} y=\frac{1}{\left(1+x^2\right)^2}\)
Solution:
This is of the form \(\frac{d y}{d x}\) + Py = Q where
P = \(\frac{4 x}{1+x^2}\) and Q = \(\frac{1}{\left(1+x^2\right)^2}\)
∴ I.F = e^{∫ P dx}
= \(e^{\int \frac{4 x}{1+x^2} d x}\)
= e^{2 log (1 + x2)}
= (1 + x²)² dx + c
= x + c is the solution.

Question 8.
x \(\frac{d y}{d x}\) + y = (1 + x) e^x
Solution:
The given equation can be written as
\(\frac{d y}{d x}+\frac{y}{x}=\frac{1+x}{x} e^x\)
∴ I.F = e^{∫ P dx}
= e^{∫ \(\frac{1}{x}\) dx}
= e^{log x} = x
∴ Solution is
y . x = ∫ \(\frac{(1+x)}{x}\) e^x . x dx + c
= ∫ (1 + x) e^x dx + c
= ∫ e^x dx + ∫ x e^x dx + c
= e^x + x e^x – e^x + c
= x e^x + c is the solution.

Question 9.
\(\frac{d y}{d x}+\frac{3 x^2}{1+x^3} y=\frac{1+x^2}{1+x^3}\)
Solution:

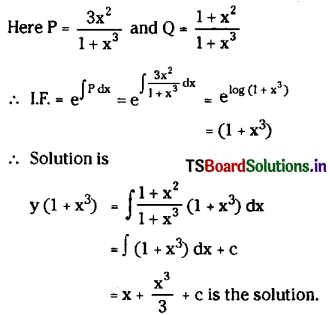
Question 10.
\(\frac{d y}{d x}\) – y = – 2 e^-x
Solution:
Here P = – 1 and Q = – 2 e^-x
∴ I.F. = e^{∫ P dx} dx
= e^{∫ – 1 dx} dx
∴ Solution is
y . e^-x = ∫ – 2 e^-x e^-x dx
= – 2 ∫ e^-2x dx
= \(\frac{(-2)}{(-2)}\) e^-2x + c
= e^-2x + c
∴ y = e^-x + C e^x is the solution.

Question 11.
(1 + x²) \(\frac{\mathrm{dy}}{\mathrm{dx}}\) + y = Tan^-1 x
Solution:
The equation can be written as

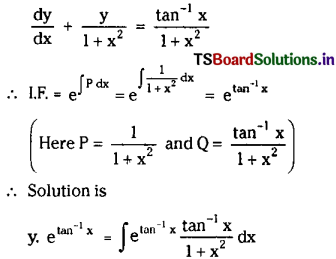
= ∫ t e^t dt where t = tan^-1 x
= t e^t – e^t
= e^{tan-1 x (tan-1 – 1)} + c
y = (tan^-1 x – 1) + c e^{– tan-1 x} is the solution.

Question 12.
\(\frac{d y}{d x}\) + y tan x = sin x
Solution:
We have P = tan x and Q = sin x
∴ I.F = e^{∫ P dx} dx
= e^{∫ tan x dx} dx
= e^{log sec x} dx = sec x
∴ Solution is
y sec x = ∫ sin x . sec x dx + c
= ∫ tan x dx
= log |sec x| + c is the solution.

III. Solve the following differential equations.

Question 1.
cos x + y sin x = sec² x
Solution:
The given equation can be written as
\(\frac{d y}{d x}\) + sin x sec x =sec x
Here P = sin x sec x – tan x and Q = sec³ x
∴ I.F. = e^{∫ P dx} dx
= e^{∫ tan x dx} dx
= e^{log (sec x)} = sec x
∴ Solution is
y sec x = ∫ sec⁴ x dx + c
= ∫ (1 + tan² x) sec² x dx + c
= tan x + \(\frac{\tan ^3 x}{3}\) + c.

Question 2.
sec x dy = (y + sin x) dx
Solution:
The given equation can be written as
sec x \(\frac{d y}{d x}\) = y + sin x
⇒ \(\frac{d y}{d x}=\frac{y}{\sec x}+\frac{\sin x}{\sec x}\)
= y cos x + sin x cos x
\(\frac{d y}{d x}\) – y cos x = sin x cos x
I.F. = e^{∫ P dx}
= e^{– ∫ cos x dx}
= e^{– sin x}
∴ Solution is y e^{– sin x}
= ∫ sin x cos x e^{– sin x} dx
= ∫ t e^{– t} dt where t = sin x
= t (- e^{– t}) + ∫ e^{– t} dt
= – e^{– t} (t + 1) + c
= – e^{– sin x} (sin x + 1) + c
y = – (sin x + 1) + c e^{sin x} is the solution.

Question 3.
x log x \(\frac{d y}{d x}\) + y = 2 log x
Solution:
The equation can be written as

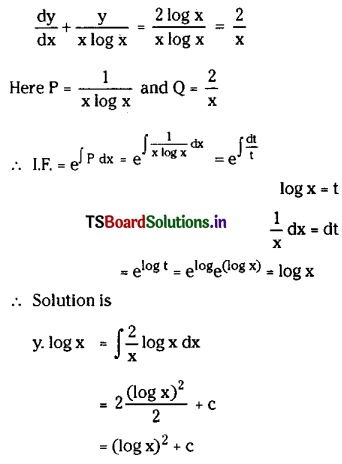
Question 4.
(x + y + 1) \(\frac{d y}{d x}\) = 1
Solution:
From the given equation
\(\frac{d y}{d x}=\frac{1}{x+y+1}\)
\(\frac{d y}{d x}\) = x + y + 1
∴ \(\frac{d y}{d x}\) – x = (y + 1)
This is of the form \(\frac{d x}{d y}\) + x = Q(y)
P = – 1 and Q = (y + 1)
∴ I.F. = e^{∫ P dy} = e^{– y}
∴ Solution is
x e^{– y} = ∫ (y + 1) e^{– y} dy
= ∫ e^{– y} y dy + ∫ e^{– y} dy
= – y e^{– y} + ∫ e^{– y} dy – e^{– y}
= – y e^{– y} – e^{– y} – e^{– y} + c
= – y e^{– y} – 2 e^{– y} + c
x = – y – 2 + ce^y
= – (y + 2) ce^y is the solution.

Question 5.
x (x – 1) \(\frac{d y}{d x}\) – y = x³ (x – 1)³
Solution:
The equation can be written as

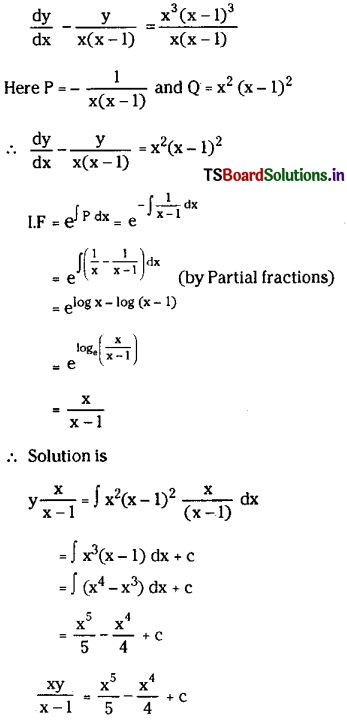
Question 6.
(x + 2y³) \(\frac{d y}{d x}\) = y.
Solution:
The given differential equation is
(x + 2y³) \(\frac{d y}{d x}\) = y

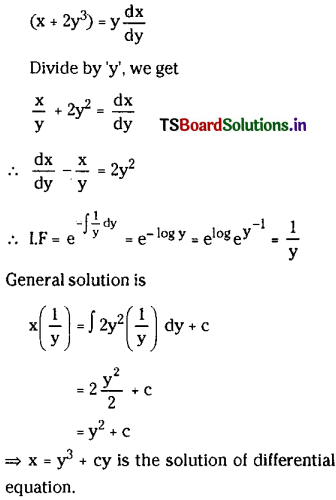
Question 7.
(1 – x²) \(\frac{d y}{d x}\) + 2xy = x \(\sqrt{1-\mathbf{x}^2}\)
Solution:
Dividing by (1 – x²) both sides

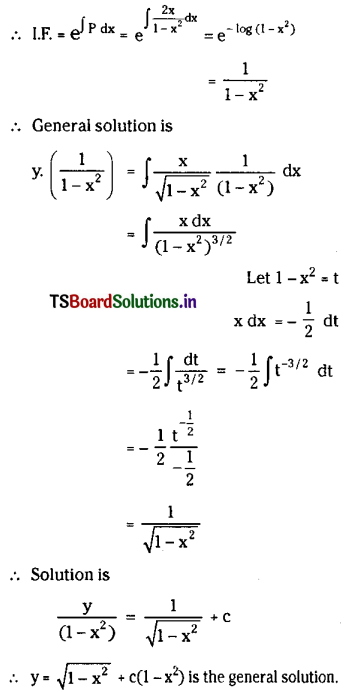
Question 8.
x (x – 1) \(\frac{d y}{d x}\) – (x – 2) y = x³ (2x – 1)
Solution:
Dividing the given equation by x(x – 1) we get
\(\frac{d y}{d x}+\frac{(-x+2)}{x(x-1)} y=\frac{x^2(2 x-1)}{x-1}\)
Now \(\frac{2-x}{x(x-1)}=\frac{A}{x}+\frac{B}{x-1}\)
∴ 2 – x = A (x – 1) + Bx
Put x = 1 both sides, 1 = B
and A + B = – 1
⇒ A = – 2
∴ \(\frac{2-x}{x(x-1)}=\frac{-2}{x}+\frac{1}{x-1}\)
∴ I.F = \(e^{\int \frac{2-x}{x(x-1)} d x}=e^{\int\left(\frac{-2}{x}+\frac{1}{x-1}\right) d x}\)
= e^{log (x – 1) – 2 log x}
= e^{log (x – 1) – log x2}
= \(\frac{x-1}{x^2}\)
∴ General Solution is \(y\left(\frac{x-1}{x^2}\right)=\int \frac{x^2(2 x-1)}{x-1}\left(\frac{x-1}{x^2}\right) d x\)
= ∫ (2x – 1) dx + c
= x² – x + c
∴ y (x – 1) = x² (x² – x + c) is the solution of the differential equation.

Question 9.
\(\frac{d y}{d x}\) (x² y³ + xy) = 1
Solution:

Question 10.
\(\frac{d y}{d x}\) + x sin 2y = x³ cos² y
Solution:
Dividing by cos² y we get
sec² y \(\frac{d y}{d x}\) + 2x tan y = x³ …………….(1)
[∴ \(\frac{\sin 2 y}{\cos ^2 y}=\frac{2 \sin y \cos y}{\cos ^2 y}\) = 2 tan y]
Let tan y = t then sec² y \(\frac{d y}{d x}\) = \(\frac{d t}{d x}\)
∴ \(\frac{d t}{d x}\) + 2xt = x³
Here, P = 2x and Q = x³
∴ I.F = e^{∫ P dx} = e^x2
∴ t . e^x2 = ∫ x³ e^x2 dx
= ∫ x² . x . e^x2 dx
= \(\frac{1}{2}\) ∫ z . e^z dz
where z = x²
= \(\frac{1}{2}\) e^z (z – 1) + c
= \(\frac{1}{2}\) e^x2 (x² – 1) + c
∴ tan y e^x2 = \(\frac{1}{2}\) e^x2 (x² – 1) + c
∴ Solution of the given differential equation is tan y = \(\frac{1}{2}\) (x² – 1) + c e-^x2

Question 11.
y² + (x – \(\frac{1}{y}\)) \(\frac{d y}{d x}\) = 0.
Solution:

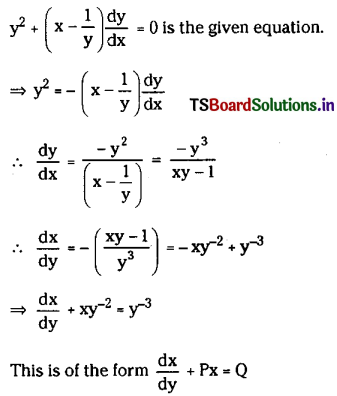
Where P = y^-2 and Q = y^-3
∴ I.F. = e^{∫ P dx}
= e∫^{y-2 dy}
= \(e^{-\frac{1}{y}}\)
∴ Solution is
x \(e^{-\frac{1}{y}}\) = ∫ \(e^{-\frac{1}{y}}\) y^-3 dy + c
= ∫ e^-y-1 y^-3 dy + c
= ∫ e^-y-1 y^-2 y^-1 dy + c
Let y^-1 = t then – y^-2 dy = dt
∴ x \(e^{-\frac{1}{y}}\) = – ∫ t e^-t dt + c
= – [- t e^-t + ∫ e^-t dt] + c
= t e^-t + e^-t + c
= e^-t (t + 1) + c
= \(e^{-\frac{1}{y}}\) (\(\frac{1}{y}\) + 1) + c
∴ x = (\(\frac{1}{y}\) + 1) + c \(e^{\frac{1}{y}}\)
⇒ xy = 1 + y + y . c\(e^{\frac{1}{y}}\)
∴ Solution of the given differential equation is xy = 1 + y + cy \(e^{\frac{1}{y}}\).