Students must practice this TS Intermediate Maths 2A Solutions Chapter 2 De Moivre’s Theorem Ex 2(b) to find a better approach to solving the problems.
TS Inter 2nd Year Maths 2A Solutions Chapter 2 De Moivre’s Theorem Exercise 2(b)
I.
Question 1.
Find all the values of
i) (1 – i√3)1/3
ii) (- i)1/6
iii) (1 + i)2/3
iv) (- 16)1/4
v) (- 32)1/5
Solution:
z = 21/3 \(\left(\frac{1}{2}-\frac{i \sqrt{3}}{2}\right)^{1 / 3}\)
= 21/3 \(\left[\cos \frac{\pi}{3}-i \sin \frac{\pi}{3}\right]^{1 / 3}\)
= 21/3 \(\left(\cos \frac{\pi}{9}-i \sin \frac{\pi}{9}\right)\)
General solution be = 21/3 cis (6k – 1) \(\frac{\pi}{9}\), k = 0, 1, 2, ……………
ii) (- i)1/6

![]()
iii) z = (1 + i)2/3
iv) z = (- 16)1/4
= (2) [(- 1)]1/4
= (2) [cos 2nπ + π) + i sin (2nπ + π)]1/4
= 2 [cis (2n + 1)π]1/4
= 2 [cis (2n + 1) \(\frac{\pi}{5}\)]
v) z = (- 32)1/5
z = 2 (- 1)1/5
= 2 [cos (2n + 1)π + i sin (2n + 1)π]1/5
= 2 [cis (2n+ 1) \(\frac{\pi}{5}\)].
![]()
Question 2.
If ABC arc angles of a triangle such that x = cis A, y = cis B, z = cis C then find the value of xyz.
Solution:
A + B + C = π
Now xyz = eiA . eiB . eiC
= ei(A + B + C)
= ei(π)
cos π + i sin π
xyz = – 1 + 0
Question 3.
i) If x = cis θ then find the value of (x6 + \(\frac{1}{x^6}\))
ii) Find cube roots of 8.
Solution:
i) x6 = eiθ
\(\frac{1}{x^6}\) = e– 6iθ
x6 + \(\frac{1}{x^6}\) = eiθ + e– 6θi
= cos 6θ + i sin 6θ + cos 6θ – isin 6θ
= 2 cos 6θ.
ii) x = (8)1/3
x3 – 8 = 0
(x – 2) (x2 + 2x + 4)
x = 2, x = \(\frac{-2 \pm \sqrt{4-16}}{2}\)
x = \(\frac{-2 \pm 2 \sqrt{3} \mathbf{i}}{2}\)
x = – 1 ± √3i
Roots are 2, 2ω, 2ω2.
![]()
Question 4.
If 1, ω, ω2 are cube roots of unity, then prove that
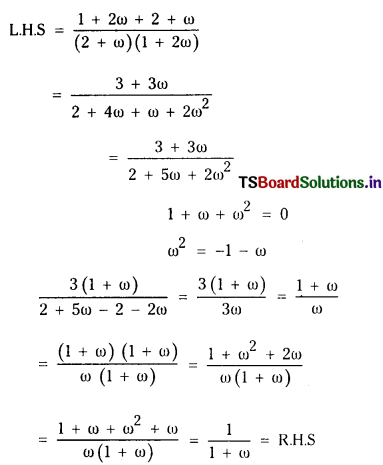
i) \(\frac{1}{2+\omega}+\frac{1}{1+2 \omega}=\frac{1}{1+\omega}\)
ii) (2 – ω) (2 – ω2) (2 – ω10) (2 – ω11) = 49
iii)(x + y + z) (x + yω + zω) (x + yω + zω) = x3 + y3 + z3 – 3xyz
Solution:
\(\frac{1}{2+\omega}+\frac{1}{1+2 \omega}=\frac{1}{1+\omega}\)
ii) (2 – ω) (2 – ω2) (2 – ω10) (2 – ω11) = 49
L.H.S = (4 – 2(ω + ω2) + ω3) (4 – 2 (ω10 + ω11) + ω21)
= (4 – 2 (- 1) + 1) (4 – 2 (ω + ω2) + (ω3)7)
= (7) (4 + 2 + 1) = 49.
iii) (x + y + z) (x + yω + zω) (x + yω + zω) = x3 + y3 + z3 – 3xyz
LH.S = (x + y + z) (x + yω + zω2) (x + yω2 + zω)
= [x2 + xyω + xzω + yx + y2ω + yzω2 + zx + zyω + z2ω] (x + yω + zω)
= [x + xy(1 + ω) + yz (ω + ω2) + zx (w2 + 1) + z2 (ω2) + y2ω] (x + yω2 + zω)
= [x3 + x2y (1 + ω) + xyz(- 1) + zx2(1 + ω2) + z2xω2 + xy2ω + yx2ω2 + 2ω(- ω) – y2zω2 – xyz + z2yω + y3 + x2zω – zxy – yz2ω – z2xω2 + z3 + zy2ω2]
= x3 + y3 + z3 – 3xyz.
![]()
Question 5.
Prove that – ω and – ω2 are roots of z2 – z + 1 = 0 where ω and ω2 are the complex cube roots of unity.
Solution:
z2 – z + 1 = 0
z = \(\frac{1 \pm \sqrt{1-4}}{2}\)
z = \(\frac{1 \pm \sqrt{3} i}{2}\)
z = \(\frac{-[-1 \mp \sqrt{3} i]}{2}\)
z = – ω, – ω2
Question 6.
If 1, ω, ω2 are the cube roots of unity, then find the values of the following.:
i) (a + b)3 + (aω + bω2)3 + (aω2 + bω)3
ii) (a + 2b)2 + (aω2 + 2bω)2 + (aω + 2bω2)2
iii) (1 – ω + ω2)3
iv) (1 – ω) (1 – ω2) (1 – ω4) (1 – ω8)
v) \(\left(\frac{a+b \omega+c \omega^2}{c+a \omega+b \omega^2}\right)+\left(\frac{a+b \omega+c \omega^2}{b+c \omega+a \omega^2}\right)\)
vi) (1 + ω)3 + (1 + ω2)3
viij (1 – ω + ω2)5 + (1 + ω – ω2)5
Solution:
i)(a + b)3 + (aω + bω2)3 + (aω2 + bω)3
= a3 + b3 + 3a2b + 3ab2 + a3ω3 + b3ω6 + 3a2ω2 . bω2 + 3aω . b2ω4 + a3ω6 . b3ω3 + 3a2bω4 . ω + 3b2ω2 . aω2
= a3 + b3 . 3a2b (1 + ω + ω) + a3 + b3 + 3b2a (ω2 + ω + 1) + a3 . b3
= 3 (a3 + b3)
ii) (a + 2b)2 + (aω2 + 2bω)2 + (aω + 2bω2)2
= a2 + 4b2 + a2ω4 + 4b2ω2 + 4abω3 + a2ω2 + 4b2ω4 + 4abω3
= a2 (1 + ω + ω2) + 4b2 (1 + ω2 + ω) + 4ab (1 + ω + ω2)
= 12ab
iii) (1 – ω + ω2)3
Now, 1 + ω + ω2 = 0
1 + ω2 = – ω
= (- ω – ω)3
= (- 2)3 ω3
= – 8.
![]()
iv) (1 – ω) (1 – ω2) (1 – ω4) (1 – ω8)
= (1 – ω – ω2 + ω3) (1 – ω) (1 – ω2)
= (1 – ω – ω2 + ω3) (1 – ω – ω2 + ω3)
= (1 + 1 + 1) (1 + 1 + 1)
=9
v) \(\left(\frac{a+b \omega+c \omega^2}{c+a \omega+b \omega^2}\right)+\left(\frac{a+b \omega+c \omega^2}{b+c \omega+a \omega^2}\right)\)
= \(\frac{\omega^2\left(a+b \omega+c \omega^2\right)}{\left(c \omega^2+a \omega^3+b \omega^4\right)}+\frac{\left(a \omega^2+b \omega^3+c \omega^4\right)}{\omega^2\left(b+c \omega+a \omega^2\right)}\)
= ω2 + \(\frac{1}{\omega^2}\)
= ω2 + \(\frac{\omega}{\omega^3}\)
= ω2 + ω = – 1
vi) (1 + ω)3 + (1 + ω2)3
= (- ω2)3 + (- ω)3
= – 1 + (- 1) = – 2.
vii) (1 – ω + ω2)5 + (1 + ω – ω2)5
1 + ω2 = – ω
= (- 2ω)5 + (- 2ω2)5
= (- 2)5 (ω2 + ω)
= (- 2)5 (- 1) = 32.
![]()
II. Question 1.
Solve the following equations.
i) x4 – 1 = 0
ii) x5 + 1 = 0
iii) x9 – x5 + x4 – 1 = 0
iv) x4 + 1 = 0
Solution:
i) x4 – 1 = 0
(x2 – 1) (x2 + 1) = 0
(x – 1) (x + 1) (x – i) (x + i) = 0
x = 1, – 1, i, – i.
ii) x5 = – 1
x = (- 1)1/5
x = [cos (2n + 1) π + i sin (2n + 1) π]1/5 n = 0, 1, 2, 3, 4
= cos(2n + 1) \(\frac{\pi}{5}\) + i sin(2n + 1) \(\frac{\pi}{5}\)
iii) x9 – x5 + x4 – 1 = 0
x5 (x4 – 1) + (x4 – 1) = 0
(x4 – 1) (x5 + 1) = 0
(x – 1) (x + 1) (x – i) (x + 1) (x5 + 1) = 0
x = ± 1, ± i, cis (2n + 1) \(\frac{\pi}{5}\).
iv) x4 + 1 = 0
x = (- 1)1/4
x = [cos (2n + 1)π + isin(2n + 1)π]1/4
x = cos (2n + 1)\(\frac{\pi}{4}\) + i sin(2n + 1)\(\frac{\pi}{4}\)
n = 0, 1, 2, 3.
![]()
Question 2.
Find the common roots of x12 – 1 = 0 and x4 + x2 + 1 = 0.
Solution:
x12 – 1 = 0
(x4)3 – 1 = o
(x4 – 1) [x8 – x4 + 1] = 0
(x – 1) (x + 1) (x – i) (x + i) (x8 + x4 + 1) = 0 (or)
x = (1)1/12
x = [cos 2nπ + i sin2nπ]1/12 n = 0, 1, 2, …………. 11 ……………(1)
x4 + x2 + 1 = 0
(x2 – 1) (x4 + x2 + 1) = 0
x6 – 1 = 0
x = (1)1/6
x = [cos 2nπ + i sin 2nπ]1/6
= \(\cos \frac{2 n \pi}{6}+i \sin \frac{2 n \pi}{6}\), n = 0, 1, 2, 3, 4, 5 …………..(2)
Common roots to (1) and (2)
cis \(\frac{\pi}{3}\), cis \(\frac{2 \pi}{3}\), cis \(\frac{4 \pi}{3}\), cis \(\frac{5 \pi}{3}\).
Question 3.
Find the number of 15th roots of unity, which are also 25th roots of unity.
Solution:
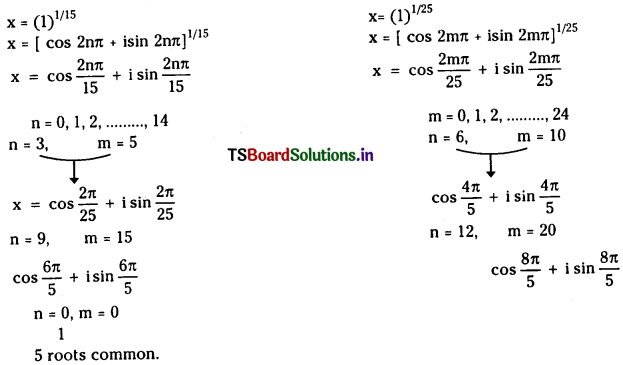
![]()
Question 4.
If the cube roots of unity are 1, ω, ω, then find the roots of the equation (x – 1)3 + 8 = 0.
Solution:
(x – 1)3 = – 8
(x – 1) = (- 8)1/3
x – 1 = – 2
x – 1 = – 2ω
x – 1 = – 2ω2
∴ x = – 1
x = – 2ω + 1
x = – 2ω2 + 1
Question 5.
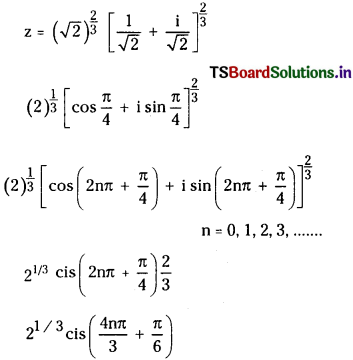
Find the roduct of all values of (1 + i)\(\frac{4}{5}\).
Solution:
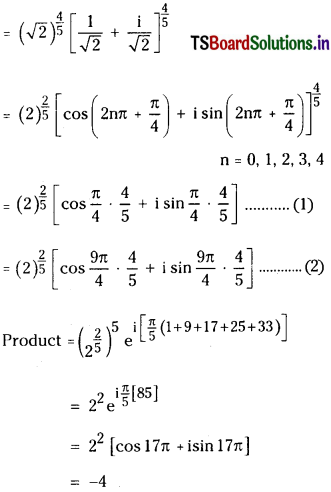
![]()
Question 6.
If z2 + z + 1 = 0, where z is a complex number, prove that
\(\left(z+\frac{1}{z}\right)^2+\left(z^2+\frac{1}{z^2}\right)^2+\left(z^3+\frac{1}{z^3}\right)^2\) + \(\left(z^4+\frac{1}{z^4}\right)^2+\left(z^5+\frac{1}{z^5}\right)^2+\left(z^6+\frac{1}{z^6}\right)^2\) = 12.
Solution:
z = ω satisfy
L.H.S = \(\left(z+\frac{1}{z}\right)^2+\left(z^2+\frac{1}{z^2}\right)^2+\left(z^3+\frac{1}{z^3}\right)^2\) + \(\left(z^4+\frac{1}{z^4}\right)^2+\left(z^5+\frac{1}{z^5}\right)^2+\left(z^6+\frac{1}{z^6}\right)^2\)
= (- 1)2 + (- 1)2 + (2)2 + (- 1)2 + (- 1)2 + (2)2 = 12.
III.
Question 1.
1, α, α2, α3, ……………. αn – 1 be the nth roots of unity then prove that 1p + αp + (α2)p + (α3)p + ………….. + (αn – 1)p = {0 if p ≠ kn, n if p = kn. where p, k ∈ N.
Solution:
Now xn – 1 = 0
x = (1)1/n
x = [cos 2nπ + i sin 2nπ]1/n
x = \(\cos \frac{2 m \pi}{n}+i \sin \frac{2 m \pi}{n}\)
α = \(\cos \frac{2 m \pi}{n}+i \sin \frac{2 m \pi}{n}\)
αp = \(\cos \frac{2 m p \pi}{n}+i \sin \frac{2 m p \pi}{n}\)
Now p = kn
1 + 1 + 1 + …………. nterms = n
If p ≠ kn value then1p + αp + (α2)p + (α3)p + ………….. + (αn – 1)p = 0.
![]()
Question 2.
Prove the sum of 99th powers of the roots of the equation x7 – 1 = 0 is zero and hence deduce the roots of x6 + x5 + x4 + x3+ x2 + x + 1 = 0.
Solution:
x7 – 1 = 0
x = (1)1/7
x = (cos 2kπ + sin 2kπ)1/7
k = 0, 1, 2, ………., 6
x1 = 1, x2 = \(e^{\frac{2 \pi}{7} i}\), x2 = \(e^{\frac{4 \pi}{7} i}\), x3 = \(e^{\frac{6 \pi}{7} i}\), ………….. x6 = \(\frac{12 \pi}{7} \mathrm{i}\)
x199 + x299 + x399 + …………..
199 + \(\mathrm{e}^{\frac{2 \pi}{7} \cdot 99 \mathrm{i}}+\mathrm{e}^{\frac{4 \pi}{7} \cdot 99 \mathrm{i}}+\ldots \ldots . \mathrm{e}^{\frac{12 \pi}{7} \cdot 99 \mathrm{i}}\) = 0
Roots of x6 + x5 + x4 + x3+ x2 + x + 1 = 0 be \(\cos \frac{2 k \pi}{7}+i \sin \frac{2 k \pi}{7}\); k = 1, 2, 3, 4, 5, 6.
∵ (x7) – 1 = (x – 1) (x6 + x5 + x4 + x3+ x2 + x + 1) = 0
x = 1 is one root
⇒ cis \(\frac{2 k \pi}{7}\); k = 1, 2, 3, 4, 5, 6.
Question 3.
If n is a positive integer, show that (P + iQ)1/n + (P – iQ)1/n = 2 (P2 + Q2)1/2n cos(\(\frac{1}{n}\) tan-1 \(\frac{Q}{P}\))
Solution:
P = r cos θ
Q = r sin θ
(P + iQ)1/n = [r cos θ + i sin θ]1/n
= r1/n \(\left[\cos \frac{\theta}{n}+i \sin \frac{\theta}{n}\right]\)
(P – iQ)1/n = [r cos θ – i sin θ]1/n
= r1/n \(\left[\cos \frac{\theta}{n}-i \sin \frac{\theta}{n}\right]\)
(P + iQ)1/n + (P – iQ)1/n = r1/n [2 cos \(\frac{\theta}{n}\)]
= 2r1/n cos \(\frac{\theta}{n}\)
Now P2 + Q2 = r2
r = (P2 + Q2)1/2
tan θ = \(\frac{Q}{P}\); θ = tan-1 \(\frac{Q}{P}\)
= 2 (P2 + Q2)1/2n cos(\(\frac{1}{n}\) tan-1 \(\frac{Q}{P}\)).
Question 4.
Show that one value of \(\left[\frac{1+\sin \frac{\pi}{8}+i \cos \frac{\pi}{8}}{1+\sin \frac{\pi}{8}-i \cos \frac{\pi}{8}}\right]^{\frac{8}{3}}\) is – 1.
Solution:
\(\left[\frac{1+\cos \left(\frac{\pi}{2}-\frac{\pi}{8}\right)+i \sin \left(\frac{\pi}{2}-\frac{\pi}{8}\right)}{1+\cos \left(\frac{\pi}{2}-\frac{\pi}{8}\right)-i \sin \left(\frac{\pi}{2}-\frac{\pi}{8}\right)}\right]^{\frac{8}{3}}\)
= \(\left[\frac{2 \cos ^2 \frac{3 \pi}{16}+2 i \sin \frac{3 \pi}{16} \cos \frac{3 \pi}{16}}{2 \cos ^2 \frac{3 \pi}{16}-2 i \sin \frac{3 \pi}{16} \cos \frac{3 \pi}{16}}\right]^{\frac{8}{3}}\)
= \(\left[\frac{\cos \frac{3 \pi}{16}+i \sin \frac{3 \pi}{16}}{\cos \frac{3 \pi}{16}-i \sin \frac{3 \pi}{16}}\right]^{\frac{8}{3}}\)
= \(\frac{\mathrm{e}^{\frac{\mathrm{i} \pi}{2}}}{\mathrm{e}^{\frac{-\mathrm{i} \pi}{2}}}\) = eiπ = – 1.
Question 5.
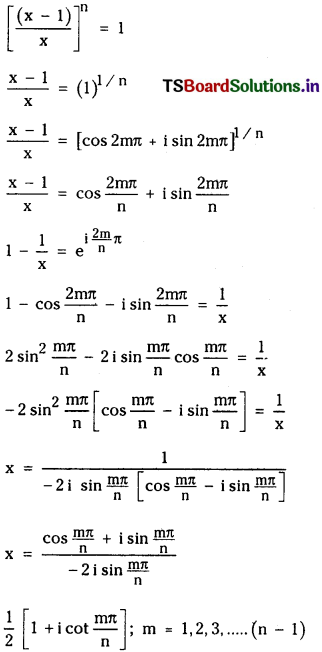
Solve (x – 1)n = xn, n is a positive integer.
Solution: