TS Inter 2nd Year Maths 2A Solutions Chapter 1 Complex Numbers Ex 1(a)

by

Students must practice this TS Intermediate Maths 2A Solutions Chapter 1 Complex Numbers Ex 1(a) to find a better approach to solving the problems.

TS Inter 2nd Year Maths 2A Solutions Chapter 1 Complex Numbers Exercise 1(a)

I. Question 1.
If z1 =(2, – 1); z2 = (6, 3) find z1 – z2.
Solution:
z1 = 2 – i; z2 = 6 + 3i
z1 – z2 = (2 – i) – (6 + 3i)
= (2 – 6) + (- 1 – 3)i
= – 4 – 4i (or)
z1 – z2 = (- 4, – 4).

Question 2.
If z1 = (3, 5) and z2 = (2, 6) find z1 . z2.
Solution:
z1 = 3 + 5i; z2 = 2 + 6i
z1 . z2 = (3 + 5i) (2 + 6i)
= 6 + 18i + 10i + 30i2
= 6 – 30 + 28i
= – 24 + 28i = (- 24, 28).

TS Board Inter 2nd Year Maths 2A Solutions Chapter 1 Complex Numbers Ex 1(a)

Question 3.
Write the additive inverse of the following complex nwnbers:
i) (√3, 5)
ii) (- 6, 5) + (10, – 4)
iii) (2, 1) (- 4, 6)
Solution:
i) z1 = √3 + 5
a + ib is additive inverse then
z1 + a + ib = 0 + 0i
(√3 + a) + (5 + b)i = 0 + 0i
a = – √3, b = – 5
∴ Additive inverse of z1 is – √3 – 5i (- √3, – 5).

ii) z1 = – 6 + 5i; z2 = 10 – 4i
z1 + z2 = (- 6 + 10) + i (5 – 4) = 4 + i.
Additive inverse be a + ib
(z1 + z2) + a + ib = 0 + 0i
(4 + a) + (1 + b)i = 0 + 0i
a = – 4
b = – 1
∴ – 4 – i = (- 4, – 1)

iii) z1 = 2 + i; z2 = – 4 + 6i
z1z2 = (2 + i) (- 4 + 6i)
= – 8 + 12i – 4i + 6i2
= – 8 – 6 + 8i
= – 14 + 8i
z1z2 + a + ib = 0 + 0i
(- 14 + a) + (8 + b) i = 0 + 0i
a = 14, b = – 8
∴ Additive inverse of z1z2 be 14 – 8i or (14, – 8).

TS Board Inter 2nd Year Maths 2A Solutions Chapter 1 Complex Numbers Ex 1(a)

II. Question 1.
If z1 = (6, 3); z2 =(2,- 1) find z1/z2.
Solution:
z1 = 6 + 3i; z2 = 2 – i

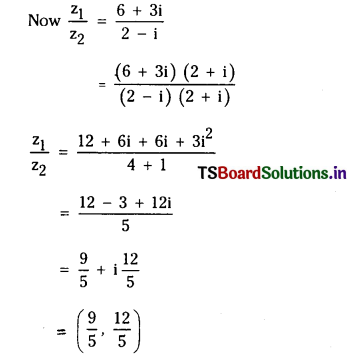
TS Inter 2nd Year Maths 2A Solutions Chapter 1 Complex Numbers Exercise 1(a) 1

Question 2.
If z = (cos θ, sin θ) find z – \(\frac{1}{z}\).
Solution:
z – \(\frac{1}{z}\) = (cos θ + i sin θ) – \(\)
= cos θ + i sin θ – \(\frac{(\cos \theta-i \sin \theta)}{(\cos \theta+i \sin \theta)(\cos \theta-i \sin \theta)}\)
= cos θ + i sin θ – \(\frac{(\cos \theta-i \sin \theta)}{\left(\cos ^2 \theta+\sin ^2 \theta\right)}\)
= 2i sin θ
= θ + 2i sin θ
= (0, 2 sin θ).

TS Board Inter 2nd Year Maths 2A Solutions Chapter 1 Complex Numbers Ex 1(a)

Question 3.
Write the multiplicative inverse of the following complex numbers:
i) (3, 4)
ii) (sin θ, cos θ)
iii) (7, 24)
iv)(- 2, 1)
Solution:
i) z1 = 3 + 4i
a + ib is multiplicative inverse of z1
z (a + ib) = 1 + 0i
a + ib = \(\frac{1}{\mathrm{z}_1}\)
a + ib = \(\frac{1}{3+4 i}\)
a + ib = \(\frac{3-4 i}{(3+4 i)(3-4 i)}\)
= \(\frac{3-4 i}{9+16}=\frac{3}{25}-\frac{4}{25} i\)
= \(\left(\frac{3}{25}, \frac{-4}{25}\right)\)

ii) z = sin θ + i cos θ
a + ib is multiplicative inverse of z1
z1 . (a + ib) = 1 + 0i
a + ib = \(\frac{1}{\mathrm{z}_1}\)
= \(\frac{1}{\sin \theta+i \cos \theta}\)
= \(\frac{\sin \theta-i \cos \theta}{(\sin \theta+i \cos \theta)(\sin \theta-i \cos \theta)}\)
= \(\frac{\sin \theta-i \cos \theta}{\sin ^2 \theta+\cos ^2 \theta}\)
= (sin θ, – cos θ).

TS Board Inter 2nd Year Maths 2A Solutions Chapter 1 Complex Numbers Ex 1(a)

iii) z1 = 7 + 24i
a + ib is multiplicative inverse of z1
z1 . a + ib = 1 + 0i
a + ib = \(\frac{1}{7+24 i}\)
= \(\frac{7-24 \mathrm{i}}{(7+24 \mathrm{i})(7-24 \mathrm{i})}\)
= \(\frac{7-24 i}{49+576}\)
= \(\frac{7}{625}-\frac{24}{625} 1=\left(\frac{7}{625}, \frac{-24}{625}\right)\)

iv) z1 = – 2 + i
z1 . (a + ib) = 1 + oi
z1 = \(\frac{1}{a+i b}\)
a + ib = \(\frac{1}{z_1}\)
= \(\frac{1}{-2+1}\)
= \(\frac{-2-i}{(-2+i)(-2-i)}\)
= \(\frac{-2-i}{4+1}\)
= \(\left(\frac{-2}{5}, \frac{-1}{5}\right)\)

Leave a Comment