TS 10th Class Maths Solutions Chapter 10 Mensuration Optional Exercise

Students can practice 10th Class Maths Study Material Telangana Chapter 10 Mensuration Optional Exercise to get the best methods of solving problems.

TS 10th Class Maths Solutions Chapter 10 Mensuration Optional Exercise

Question 1.
A golf ball has diameter equal to 4.1cm. Its surface has 150 dimples each of radius 2mm. Calculate total surface area which is exposed to the surroundings (Assume that the dimples are all hemispherical) [π = \(\frac{22}{7}\)]
Solution:
Area exposed = surface area of the ball – total area of 150 dimples with radius 2 mm
= 4πr² – 150 × πr²
= 4 × \(\frac{22}{7}\) × \(\frac{4.1}{2}\) × \(\frac{4.1}{2}\) – 150 × \(\frac{22}{7}\) × \(\frac{2}{10}\) × \(\frac{2}{10}\)
[∵ 2 mm = \(\frac{2}{10}\) cm]
= 52.831 – 18.85 = 33.972 cm²

Question 2.
A cylinder of radius 12 cm. contains water to a depth of 20 cm. when a spherical iron ball is dropped in to the cylinder and thus the level of water is raised by 6.75 cm. Find the radius of the ball. [π = \(\frac{22}{7}\)]
Solution:
Rise in the water level is seen as a cylinder of radius ‘r’ = r₁ = 12 cm
Height, h = 6.75 cm
Volume of the rise = Volume of the spherical iron ball dropped
πr₁²h = \(\frac{4}{3}\) πr₂³
r₁²h = \(\frac{4}{3}\) π₂³
12 × 12 × 6.75 cm³ = \(\frac{4}{3}\) × r₂³ cm³
r₂³ = \(\frac{3}{4}\) × 12 × 12 × 6.75
= 9 × 12 × 6.75
= 108 × 5.75
r₂³ = 729
r₂³ = 9 × 9 × 9
∴ 729 = (3 × 3) × (3 × 3) × (3 × 3)
∴ Radius of the ball r = r₂ = 9 cm.

Question 3.
A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm. and height of the cylindrical and conical portion are 12 cm. and 7 cm. respectively. Find the volume of the solid toy. [π = \(\frac{22}{7}\)]
Solution:

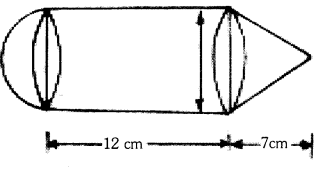
Volume of the toy
= volume of the hemisphere + volume of the cylinder + volume of the cone.
= \(\frac{2}{3}\) πr³ + πr²h₁ + \(\frac{1}{3}\) πr²h₂
= πr²(\(\frac{2}{3}\)r + h₁ + \(\frac{\mathrm{h}_2}{3}\))
= \(\frac{22}{7}\) × \(\frac{4.2}{2}\) × \(\frac{4.2}{2}\)[\(\frac{2}{3}\) × \(\frac{4.2}{2}\) + 12 + \(\frac{7}{3}\)]
= 11 × 0.6 × 2.1 [1.4 + 12 + \(\frac{7}{3}\)]
= 13.86[13.4 + \(\frac{7}{3}\)]
= 13.86 × \(\left[\frac{40.2+7}{3}\right]\)
= \(\frac{13.86 \times 47.2}{3}\) = 218.064 cm³

(or)

Hemisphere :
Radius = \(\frac{\text { diameter }}{2}\) = \(\frac{4.2}{2}\) = 2.1 cm
V = \(\frac{2}{3}\) πr³ = \(\frac{2}{3}\) × \(\frac{22}{7}\) × 2.1 × 2.1 × 2.1
= 19.404 cm³

Cylinder :
Radius, r = \(\frac{\mathrm{d}}{2}\) = \(\frac{4.2}{2}\) = 2.1 cm
height, h = 12 cm
V = πr²h = \(\frac{22}{7}\) × 2.1 × 2.1 × 12
= 166.32 cm³

Cone :
Radius, r = \(\frac{\mathrm{d}}{2}\) = \(\frac{4.2}{2}\) = 2.1cm
Height, h = 7 cm
V = \(\frac{1}{3}\) πr²h = \(\frac{1}{3}\) × \(\frac{22}{7}\) × 2.1 × 2.1 × 7
= 32.34 cm³
∴ Total volume = 19.404 + 166.32 + 32.34
= 218.064 cm³.

Question 4.
Three metal cubes with edges 15 cm., 12 cm. and 9 cm. respectively are melted together and formed into a single cube. Find the diagonal of this cube.
Solution:
Edges l₁ = 15 cm, l₂ = 12 cm, l₁₃ = 9 cm.

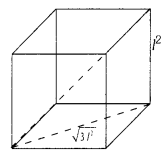
Volume of the resulting cube = Sum of the volumes of the three given cubes
L³ = l³₁h
L³ = l₁³ + l₂³ + l₃³
L³ = 15³ + 12³ + 9³
L³ = 3375 + 1728 + 729
L³ = 5832 = 18 × 18 × 18
∴ Edge of the new cube l = 18 cm
Diagonal = \(\sqrt{3 l^2}\) = \(\sqrt{3 \times 18^2}\)
= \(\sqrt{3 \times 324}\) = \(\sqrt{972}\) = 31.176 cm.

Question 5.
A hemi-spherical bowl of internal diameter 36 cm. contains a liquid. This liquid is to be filled in cylindrical bottles of radius 3 cm. and height 6 cm. How many bottles are required to empty the bowl ?
Solution:
Let the number of bottles required = n.
Then total valume of a ’n’ bottles = volume of the hemispherical bowl.
n. πr₁²h = \(\frac{2}{3}\) πr₂²h
Bottle :
Radius, r₁ = 3 cm
Height, h = 6 cm
Volume, V = πr₁²h
= \(\frac{22}{7}\) × 3 × 3 × 6 = \(\frac{1188}{7}\)
∴ Total volume of n bottles = n × \(\frac{1188}{7}\) cm³.
Bowl :

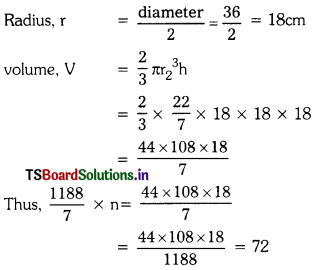
∴ 72 bottles are required to empty the bowl.